A small coin is placed at a distance r from the centre of a gramophone record.The rotational speed of the record is gradually increased. If the coefficient of friction between the coin and the record is μ, the minimum angular frequency of the record for which the coin will fly off is given by :
√2μgr
√μg2r
√μgr
2√μgr
The correct Answer is:C
To solve the problem of determining the minimum angular frequency of a gramophone record at which a coin will fly off, we can follow these steps:
Step 1: Identify the Forces Acting on the Coin
When the gramophone record rotates, the coin experiences two main forces:
1. Centrifugal Force: This force acts outward and is given by the formula:
Fcentrifugal=mrω2
where m is the mass of the coin, r is the distance from the center of the record, and ω is the angular frequency of the record.
2. Frictional Force: This force acts inward and is given by:
Ffriction=μN
where μ is the coefficient of friction and N is the normal force acting on the coin.
Step 2: Establish the Relationship Between Forces
For the coin to remain on the record, the frictional force must be equal to or greater than the centrifugal force. At the point of flying off, these forces are equal:
Ffriction=Fcentrifugal
Substituting the expressions for these forces, we get:
μN=mrω2
Step 3: Determine the Normal Force
The normal force N acting on the coin is equal to the weight of the coin, which can be expressed as:
N=mg
where g is the acceleration due to gravity.
Step 4: Substitute the Normal Force into the Equation
Substituting N=mg into the force balance equation gives:
μ(mg)=mrω2
Step 5: Simplify the Equation
We can cancel m from both sides of the equation (assuming m≠0):
μg=rω2
Step 6: Solve for Angular Frequency ω
Rearranging the equation to solve for ω:
ω2=μgr
Taking the square root of both sides, we find:
ω=√μgr
Final Answer
Thus, the minimum angular frequency of the record for which the coin will fly off is:
ω=√μgr
When the gramophone record rotates, the coin experiences two main forces:
1. Centrifugal Force: This force acts outward and is given by the formula:
Fcentrifugal=mrω2
where m is the mass of the coin, r is the distance from the center of the record, and ω is the angular frequency of the record.
Ffriction=μN
where μ is the coefficient of friction and N is the normal force acting on the coin.
For the coin to remain on the record, the frictional force must be equal to or greater than the centrifugal force. At the point of flying off, these forces are equal:
Ffriction=Fcentrifugal
Substituting the expressions for these forces, we get:
μN=mrω2
The normal force N acting on the coin is equal to the weight of the coin, which can be expressed as:
N=mg
where g is the acceleration due to gravity.
Substituting N=mg into the force balance equation gives:
μ(mg)=mrω2
We can cancel m from both sides of the equation (assuming m≠0):
μg=rω2
Rearranging the equation to solve for ω:
ω2=μgr
Taking the square root of both sides, we find:
ω=√μgr
Thus, the minimum angular frequency of the record for which the coin will fly off is:
ω=√μgr
Similar Questions
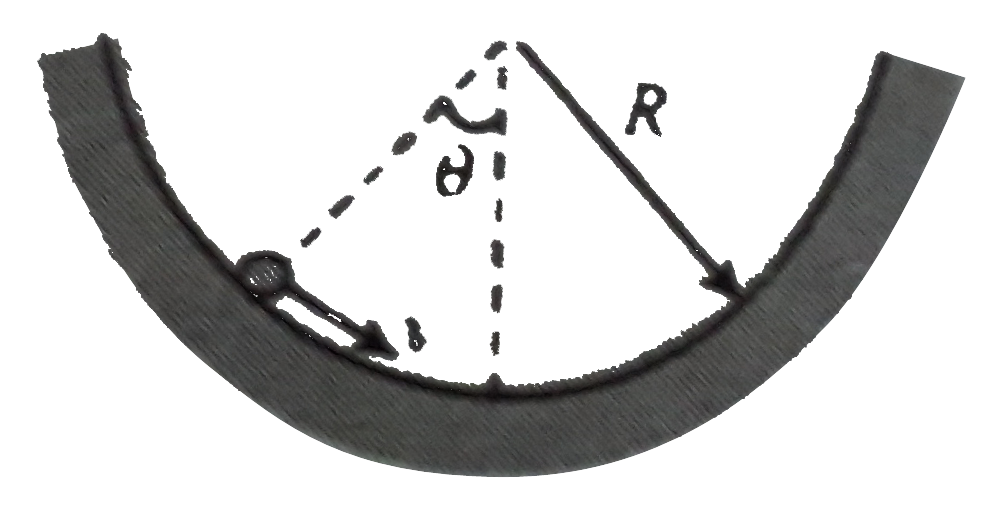
A coin is pushed down tangentially from position θ on a cylindrical surface with a velocity v as shown in Fig. If the coefficient of friction between the coin and surface is μ find the tangential acceleration of the coin
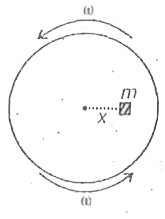
View SolutionA block of mass m is kept on horizontal turn table at x distance from the centre. If coefficient of friction between block and surface of turn table is μ, then maximum angular speed of the table so that block does not slip
View Solution
Knowledge Check
A disc revovles with a speed of 3313rev/min and has a radius of 15 cm Two coins are palaced at 4 cm and 14 cm away from the center of the record If the coefficient of friction between the coins and the record is 0.5 which of the coins will revolve with the road ?
AABBCBoth A and BDNeither A nor B
A person stands in contact against the inner wall of a rotor of radius r. The coefficient of friction between the wall and the clothing is μ and the rotor is rotating about vertical axis. The minimum angular speed of the rotor so that the person does not slip downward is
View SolutionA gramophone record is revolving with an angular velocity ω. A coin is placed at a distance R from the centre of the record. The static coefficient of friction is μ. The coin will revolve with the record if
View SolutionA disc starts rotating with a constant angular acceleration of 1rad/s2 about a fixed vertical axis perpendicular to its plane and passing through its centre. A coin of mass 10gm is placed on the disc at a distance of 10cm from the axis. The coefficent of friction between the disc and the coin is 0.2. The value of the frictional force on the coin at t=1s will be
View SolutionIn the diagram shown,the coefficient of friction between the blocks is equal to mu.Find out the maximum angular speed with which the system can be rotated so that no slipping takes place?
_PHY_E05_037_Q01.png)
View SolutionA block of mass m kept on rough horizontal turn table at a distance x from the centre. If the coefficient of friction between the table and block is μ then maximum speed of point P on turn table so that the block does not slip is

View SolutionA coin is kept at distance of 10 cm from the centre of a circular turn table. If μ=0.8 the frequency of rotation at which the coin just begins to slip is
View SolutionA 70 kg man stands in contact against the inner wall of a hollow cylindrical drum of radius 3 m rotating about its vertical axis with 200 rev/min. The coefficient of friction between the wall and his clothing is 0.15 m What is the minimum rotational speed of the cylinder to enable the man to remain stuck to the wall (without falling) when the floor is suddenly removed ?
View Solution
