A gramophone record is revolving with an angular velocity ω. A coin is placed at a distance R from the centre of the record. The static coefficient of friction is μ. The coin will revolve with the record if
r=μgω2
r<ω2μg
r≤μgω2
r≥μgω2
The correct Answer is:c
To solve the problem, we need to analyze the forces acting on the coin placed on a gramophone record that is rotating with an angular velocity ω. Here’s a step-by-step solution:
Step 1: Identify the Forces Acting on the Coin
The coin experiences two main forces:
1. Centrifugal Force: This is the outward force experienced due to the circular motion of the coin. It can be expressed as:
Fcentrifugal=mω2R
where m is the mass of the coin, ω is the angular velocity, and R is the distance from the center of the record to the coin.
2. Frictional Force: This is the force that provides the necessary centripetal force to keep the coin moving in a circle. The maximum static frictional force can be expressed as:
Ffriction=μmg
where μ is the coefficient of static friction and g is the acceleration due to gravity.
Step 2: Set Up the Condition for the Coin to Revolve
For the coin to revolve with the record without slipping, the frictional force must be equal to or greater than the centrifugal force. Thus, we have the inequality:
μmg≥mω2R
Step 3: Simplify the Inequality
We can cancel the mass m from both sides of the inequality (assuming m≠0):
μg≥ω2R
Step 4: Rearrange to Find the Maximum Radius
Rearranging the inequality gives us:
R≤μgω2
This means that the coin will revolve with the record if the distance R from the center of the record is less than or equal to μgω2.
Conclusion
Thus, the condition for the coin to revolve with the gramophone record is:
R≤μgω2
The coin experiences two main forces:
1. Centrifugal Force: This is the outward force experienced due to the circular motion of the coin. It can be expressed as:
Fcentrifugal=mω2R
where m is the mass of the coin, ω is the angular velocity, and R is the distance from the center of the record to the coin.
Ffriction=μmg
where μ is the coefficient of static friction and g is the acceleration due to gravity.
For the coin to revolve with the record without slipping, the frictional force must be equal to or greater than the centrifugal force. Thus, we have the inequality:
μmg≥mω2R
We can cancel the mass m from both sides of the inequality (assuming m≠0):
μg≥ω2R
Rearranging the inequality gives us:
R≤μgω2
This means that the coin will revolve with the record if the distance R from the center of the record is less than or equal to μgω2.
Thus, the condition for the coin to revolve with the gramophone record is:
R≤μgω2
Similar Questions
A long playing record revolves with a speed of 33(14) rev/min. and has a radius of 15 cm. Two coins are placed at 4 cm and 14 cm away from the centre of the record. If the coefficients of friction between the coin and the record is 0.15, which of the two coins will revolve with record ?
View SolutionA long plying record revolves with a speed of 3313rev min−1, and has a radius of 15 cm. Two coins are placed at 4 cm and 14 cm away from the centre of the record. If the coefficient of friction between the coins and the record is 0.15, which of the two coins will revolve with the record ? Take g=9.8ms−2.
View Solution
Knowledge Check
A disc revovles with a speed of 3313rev/min and has a radius of 15 cm Two coins are palaced at 4 cm and 14 cm away from the center of the record If the coefficient of friction between the coins and the record is 0.5 which of the coins will revolve with the road ?
AABBCBoth A and BDNeither A nor BA ring of radius R is rotating with an angular speed ω0 about a horizontal axis. It is placed on a rough horizontal table. The coefficient of kinetic friction is μk. The time after it starts rolling is.
AωoμkR2gBωog2μkRC2ωoRμkgDωoR2μkg
An α is moving along a circle of radius R with a constant angular velocity ω. Point A lies in the same plane at a distance 2R from the centre. Point A records magnetic field produced by the α-particle. If the minimum time interval between two successive time at which A records zero magnetic field is t, the angular speed ω, in terms of t, is
View SolutionA gramophone disc rotates at 60 rpm . A coin of mass 18 g is placed at a distance of 8 cm from the centre . Calculate centrifugal force on the coin . Take π2=9.87 .
View SolutionA disc of radius R=2m starts rotating wth constant angular acceleration α=(2rad)/s2. A block of mass m=2kg is kept at a distance R2 from the centre of disc. The coeffiecient of friction between disc and block is μs=0.4,μk=0.3. Acceleration of block when it just slips w.r.t. ground and w.r.t. disc are respectively−(g=10ms−2)
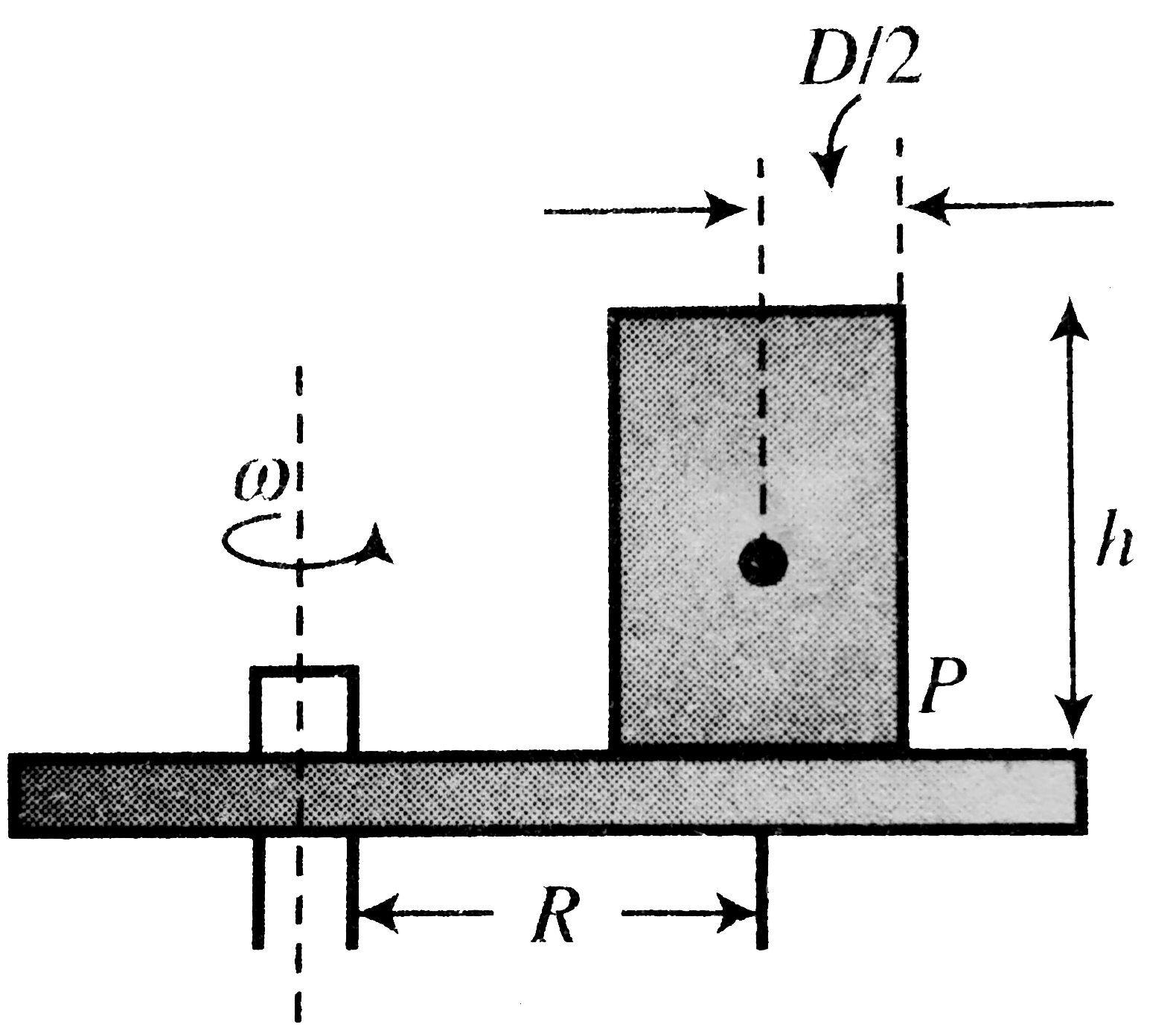
View SolutionA cylinder rests on a horizontal rotating disc, as shown in the figure. Find at what angular velocity, ω, the cylinder falls off the disc, if the distance between the axes of the disc and cylinder is R, and the coefficient of friction μ>D/h where D is the diameter of the cylinder and It is its height.
View SolutionA small coin is placed at a distance r from the centre of a gramophone record.The rotational speed of the record is gradually increased. If the coefficient of friction between the coin and the record is μ, the minimum angular frequency of the record for which the coin will fly off is given by :
View SolutionA cylinder of mass m radius R is spined to a clockwise angular velocity ωo and then gently placed on an inclined plane for which coefficient of friction μ=tanθ,θ is the angle of inclined plane with the horizontal. The centre of mass of cylinder will remain stationary for time

View Solution
