A body of mass 1 kg is rotated in a horizontal circle of radius 1 m and moves with velocity 2ms−1 The work done in 10 revolutions is
40 J
20 J
4×2π(10)J
zero
The correct Answer is:D
To solve the problem of calculating the work done in 10 revolutions by a body of mass 1 kg rotating in a horizontal circle of radius 1 m with a velocity of 2 m/s, we can follow these steps:
1. Understanding the Motion:
The body is moving in a circular path. After completing 10 revolutions, it returns to its initial position.
2. Displacement Calculation:
Since the body returns to its starting point after 10 revolutions, the net displacement is zero. Displacement is defined as the shortest distance from the initial to the final position.
3. Work Done Formula:
The work done W is given by the formula:
W=F⋅d
where F is the force applied and d is the displacement.
4. Force Analysis:
In circular motion, the only force acting on the body (tension in the string or centripetal force) is directed towards the center of the circle. This force is always perpendicular to the direction of motion (tangential direction).
5. Work Done Calculation:
Since the displacement after 10 revolutions is zero, the work done is:
W=F⋅0=0
6. Conclusion:
Therefore, the work done in 10 revolutions is zero.
Final Answer:
The work done in 10 revolutions is 0J.
---
The body is moving in a circular path. After completing 10 revolutions, it returns to its initial position.
Since the body returns to its starting point after 10 revolutions, the net displacement is zero. Displacement is defined as the shortest distance from the initial to the final position.
The work done W is given by the formula:
W=F⋅d
where F is the force applied and d is the displacement.
In circular motion, the only force acting on the body (tension in the string or centripetal force) is directed towards the center of the circle. This force is always perpendicular to the direction of motion (tangential direction).
Since the displacement after 10 revolutions is zero, the work done is:
W=F⋅0=0
Therefore, the work done in 10 revolutions is zero.
The work done in 10 revolutions is 0J.
Similar Questions
An object of mass 10 kg falls from rest through a vertical distance of 10 m and acquires a velocity of 10ms−1. The work done by the push of air on the object is (g=10ms−2)
View SolutionA body of mass 100 g is rotating in a circular path of radius r with constant speed. The work done in one complete revolution is
View Solution
Knowledge Check
A body of mass m is moving in a circle of radius r with a constant speed v. The work done by the centripetal force in moving the body over half the circumference of the circle is
Amv2/rBzeroCmv2/rDr2/mv2
A body of mass 5 kg moving on a horizontal surface with a velocity of 10ms−1 comes to rest in 2s. The force required to make this body move with a velocity of 10ms−1 on the same surface is
View SolutionThe breaking tension of a string is 10 N. A particle of mass 0.1 kg tied to it is rotated along a horizontal circle of radius 0.5 meter. The maximum speed with which the particle can be rotated without breaking the string is
View SolutionIf a particle of mass m is moving in a horizontal circle of radius r with a centripetal force (−1/r2) , the total energy is
View SolutionA body of mass 2kg moving on a horizontal surface with an initial velocity of 4ms−1 comes to rest after 2 second. If one wants to keep this body moving on the same surface with a velocity of 4ms−1 the force required is
View SolutionA particle moves in a circle of radius 4 m with a linear velocity of 20ms−1. Find the angular velocity.
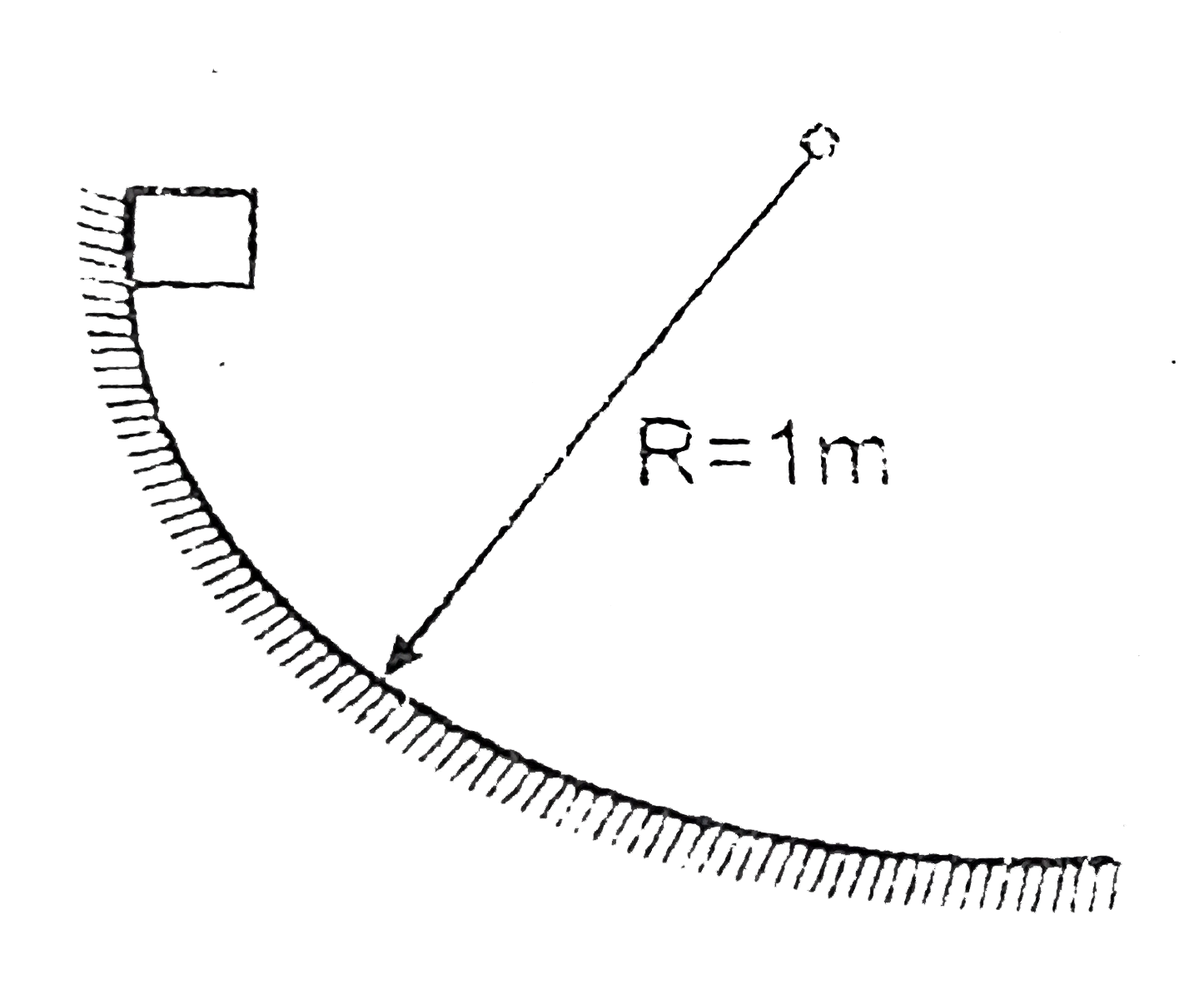
View SolutionA block of mass 1 kg slides down a vertical curved track that is one quadrant of a circle of radius 1m. Its speed ast the the bottom si 2m/s. The work done by frictional force is :
View SolutionA body of mass 10 kg initially at rest acquires velocity 10ms−1. What is the work done ?
View Solution
