Two spherical bodies of masses m and 6m and radii R and 2R respectively are released in free space with initial separation between their centres equal to 10 R . If they attract each other due to gravitational force only , then the distance covered by smaller sphere just before collisions will be
6 R
7.5 R
2.5 R
9 R
The correct Answer is:A
To solve the problem, we will follow these steps:
Step 1: Understand the initial conditions
We have two spherical bodies:
- Mass of the first body, m1=m and radius R1=R
- Mass of the second body, m2=6m and radius R2=2R
The initial separation between their centers is given as 10R.
Step 2: Calculate the distance between their surfaces
The distance between the surfaces of the two spheres can be calculated as:
Distance between surfaces=Initial separation−(Radius of first sphere+Radius of second sphere)
=10R−(R+2R)=10R−3R=7R
Step 3: Determine the gravitational force acting on each sphere
The gravitational force F between the two spheres can be calculated using Newton's law of gravitation:
F=Gm1m2d2
Where d is the distance between the centers of the two spheres, which is 10R:
F=Gm⋅6m(10R)2=6Gm2100R2=3Gm250R2
Step 4: Calculate the accelerations of both spheres
The acceleration a1 of the smaller sphere (mass m) is given by:
a1=Fm=3Gm250R2m=3Gm50R2
The acceleration a2 of the larger sphere (mass 6m) is given by:
a2=F6m=3Gm250R2⋅6m=Gm100R2
Step 5: Relate the distances covered by both spheres
Let d1 be the distance covered by the smaller sphere and d2 be the distance covered by the larger sphere. Since both spheres start from rest, we can use the ratio of their accelerations:
d1d2=a1a2=3Gm50R2Gm100R2=32
Step 6: Set up the equation for total distance
We know that the total distance covered by both spheres must equal the distance between their surfaces:
d1+d2=7R
Substituting d1=32d2 into the equation:
32d2+d2=7R
52d2=7R
d2=14R5
Step 7: Calculate d1
Now substituting back to find d1:
d1=32d2=32⋅14R5=21R5
Conclusion
The distance covered by the smaller sphere just before collision is:
6R
We have two spherical bodies:
- Mass of the first body, m1=m and radius R1=R
- Mass of the second body, m2=6m and radius R2=2R
The distance between the surfaces of the two spheres can be calculated as:
Distance between surfaces=Initial separation−(Radius of first sphere+Radius of second sphere)
=10R−(R+2R)=10R−3R=7R
The gravitational force F between the two spheres can be calculated using Newton's law of gravitation:
F=Gm1m2d2
Where d is the distance between the centers of the two spheres, which is 10R:
F=Gm⋅6m(10R)2=6Gm2100R2=3Gm250R2
The acceleration a1 of the smaller sphere (mass m) is given by:
a1=Fm=3Gm250R2m=3Gm50R2
a2=F6m=3Gm250R2⋅6m=Gm100R2
Let d1 be the distance covered by the smaller sphere and d2 be the distance covered by the larger sphere. Since both spheres start from rest, we can use the ratio of their accelerations:
d1d2=a1a2=3Gm50R2Gm100R2=32
We know that the total distance covered by both spheres must equal the distance between their surfaces:
d1+d2=7R
32d2+d2=7R
52d2=7R
d2=14R5
Now substituting back to find d1:
d1=32d2=32⋅14R5=21R5
The distance covered by the smaller sphere just before collision is:
6R
Similar Questions
Two spherical bodies of masses m and 5m and radii R and 2R respectively, are released in free space with initial separation between their centres equal to 12 R. If they attract each other due to gravitational force only, the distance covered by smaller sphere just before collision is
View SolutionTwo spherical bodies of mass M and 5M & radii R & 2R respectively are released in free space with initial separation between their centres equal to 12R. If they attract each other due to gravitational force only, then the distance covered by the smallar body just before collision is
View Solution
Knowledge Check
Two spheres each of mass M and radius R are separated by a distance of r. The gravitational potential at the midpoint of the line joining the centres of the spheres is
A−GMrB−2GMrC−GM2rD−4GMr
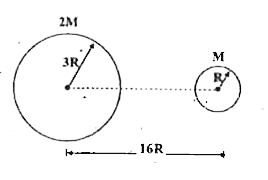
Two spherical bodies of masses 2M and M and radii 3R and R respectively are held at a distance 16R from each other in free space. When they are released they start approaching each other due to the gravitational force of attraction , then find
(a) the ratio of their accelerations during their motion
(b) their velocitites at the time of impact.
View SolutionTwo homogeneous spheres A and B of masses m and 2m having radii 2a and a respectively are placed in touch . The distance of the centre of mass from the first sphere is
View SolutionTwo concentric shells have masses M and m and their radii are R and r, respectively, where R>r. What is the gravitational potential at their common centre?
View SolutionTwo concentric spherical shells of masses and radii m1,r1andm2,r2 respectively are as shown. The gravitational field intensity at point P is

View SolutionTwo spheres each of mass M and radius R are separated by a distance of r. The gravitational potential at the midpoint of the line joining the centres of the spheres is
View SolutionTwo spherical nuclei have mass number 216 and 64 with their radii R1andR2 respectively. The ratio, R1R2 is equal to
View SolutionTwo objects of masses m and 4m are at rest at an infinite separation. They move towards each other under mutual gravitational attraction. If G is the universal gravitaitonal constant, then at separation r
View Solution