Two spherical bodies of mass M and 5M & radii R & 2R respectively are released in free space with initial separation between their centres equal to 12R. If they attract each other due to gravitational force only, then the distance covered by the smallar body just before collision is
2.5 R
4.5 R
7.5 R
1.5 R
The correct Answer is:C
To solve the problem step by step, we will analyze the motion of the two spherical bodies under the influence of their mutual gravitational attraction.
Step 1: Understanding the System
We have two spherical bodies:
- Body 1: Mass = M, Radius = R
- Body 2: Mass = 5M, Radius = 2R
The initial separation between their centers is 12R.
Step 2: Setting Up the Problem
Let the distance covered by the smaller body (mass M) before collision be xm and the distance covered by the larger body (mass 5M) be x5M.
Since they are moving towards each other, the total distance covered by both bodies when they collide will equal the initial separation minus the sum of their radii:
xm+x5M=12R−(R+2R)=9R
Step 3: Applying Newton's Second Law
The gravitational force acting on each body can be expressed as:
F=G⋅M⋅5M(12R)2=5GM2144R2
Using Newton's second law, we can express the accelerations of both bodies:
- For mass M:
F=M⋅am⟹am=FM=5GM144R2
- For mass 5M:
F=5M⋅a5M⟹a5M=F5M=GM144R2
Step 4: Finding the Ratio of Accelerations
The ratio of the accelerations can be expressed as:
ama5M=5GM144R2GM144R2=5
Step 5: Relating Distances Covered
Using the ratios of their accelerations, we can relate the distances covered:
xmx5M=a5Mam=15
This implies:
xm=5x5M
Step 6: Substituting into the Distance Equation
Now substituting xm into the equation xm+x5M=9R:
5x5M+x5M=9R⟹6x5M=9R⟹x5M=9R6=1.5R
Step 7: Finding xm
Now substituting back to find xm:
xm=5x5M=5⋅1.5R=7.5R
Conclusion
The distance covered by the smaller body (mass M) just before collision is:
7.5R
We have two spherical bodies:
- Body 1: Mass = M, Radius = R
- Body 2: Mass = 5M, Radius = 2R
Let the distance covered by the smaller body (mass M) before collision be xm and the distance covered by the larger body (mass 5M) be x5M.
xm+x5M=12R−(R+2R)=9R
The gravitational force acting on each body can be expressed as:
F=G⋅M⋅5M(12R)2=5GM2144R2
- For mass M:
F=M⋅am⟹am=FM=5GM144R2
- For mass 5M:
F=5M⋅a5M⟹a5M=F5M=GM144R2
The ratio of the accelerations can be expressed as:
ama5M=5GM144R2GM144R2=5
Using the ratios of their accelerations, we can relate the distances covered:
xmx5M=a5Mam=15
This implies:
xm=5x5M
Now substituting xm into the equation xm+x5M=9R:
5x5M+x5M=9R⟹6x5M=9R⟹x5M=9R6=1.5R
Now substituting back to find xm:
xm=5x5M=5⋅1.5R=7.5R
The distance covered by the smaller body (mass M) just before collision is:
7.5R
Topper's Solved these Questions
Similar Questions
Two spherical bodies of masses m and 5m and radii R and 2R respectively, are released in free space with initial separation between their centres equal to 12 R. If they attract each other due to gravitational force only, the distance covered by smaller sphere just before collision is
View SolutionTwo spherical bodies of masses 2M and M and radii 3R and R respectively are held at a distance 16R from each other in free space. When they are released they start approaching each other due to the gravitational force of attraction , then find
(a) the ratio of their accelerations during their motion
(b) their velocitites at the time of impact.
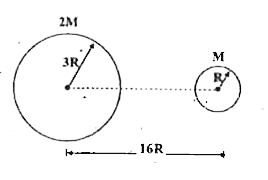
View Solution
Knowledge Check
Two spherical bodies of masses m and 5m and radii R and 2R respectively, are released in free space with initial separation between their centres equal to 12 R. If they attract each other due to gravitational force only, the distance covered by smaller sphere just before collision is
A15R2B13R2C10RD17R2Two spherical bodies of masses m and 4m and radii R and 2R respectively are released in free space with initial separation between their centres equal to 15 R. If they attract each other due to gravitational force only, then the distance covered by smaller sphere just before collision will be
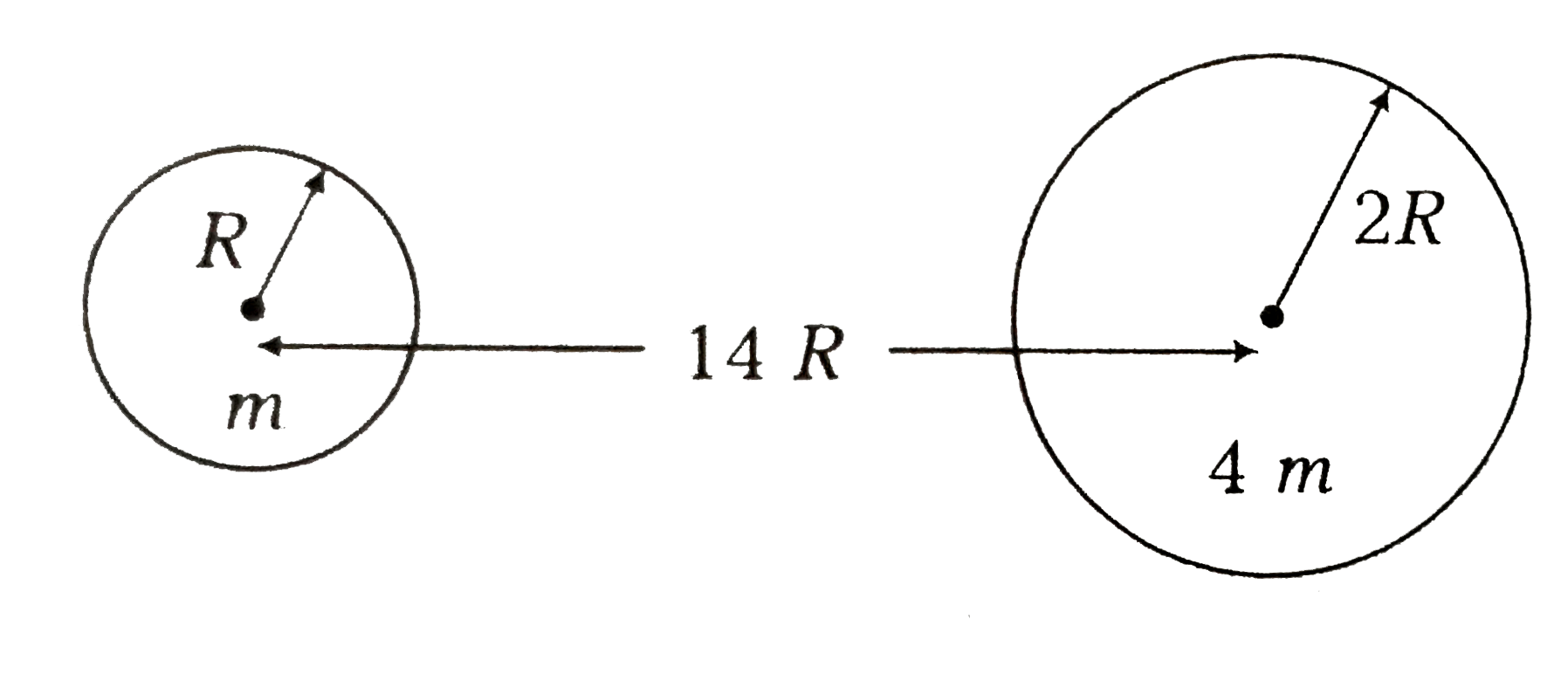 A5 RB9.6 RC2.5 RD6 R
A5 RB9.6 RC2.5 RD6 RWhich of the following statements is true ?
(i) A car of mass M is tied by one end of a massless rope of length 10m. The other end of the rope is in the hands of a man of mass M. The entire system is on a smooth horizontal surface.The man is at x=0 and the cart at x=10m. If the man pulls the cart by the rope, the man and the cart will meet at the point x=5m.
(ii) Two spherical bodies of mass M and 5M and radii R and 2R, respectively , are released in free space with initial separation between their centers equal to 12R. If they attract each other due to the gravitational force only , then the distance covered by the smaller body just before collision is 7.5R.
(iii) Two skaters A and B , having masses 40kg and 60kg , respectively stand facing each other 10m apart on a horizontal smooth surface. They pull on a rope stretched between them , the distance covered by A, when skaters meet is 6m.
(iv) A ballon (mass M) is attached to light rope of length L. To the other end of the rope a boy (mass m) is hanging in air. The system is at rest. The distance travelled by the balloon (in downward direction) when the boy touches the ballon is MLM+m.A(i),(ii)B(i),(ii),(iii)C(iii),(iv)Dall of the above
Two spherical bodies of masses m and 6m and radii R and 2R respectively are released in free space with initial separation between their centres equal to 10 R . If they attract each other due to gravitational force only , then the distance covered by smaller sphere just before collisions will be
View SolutionTwo lead balls of masses m and 5m having radii R and 2R are separated by 12R. If they attract each other by gravitational force, the distance covered by small sphere before they touch each other is
View SolutionTwo identical spheres each of mass M and radius R are separated by a distance 3R. The force of attraction between them is proportional to
View SolutionTwo particles of mass m1 and m2, approach each other due to their mutual gravitational attraction only. Then
View SolutionTwo particles of mass m1 and m2, approach each other due to their mutual gravitational attraction only. Then
View Solution
