The electric potential at. Point A is 20 V and B is – 20 V. The work done by an external force in moving electron slowly from B to A is
(a)−6.4×10−18J
(b)+6.4×10−18J
(c)+4×10−20J
(d)−4×10−20J
The correct Answer is:A
To solve the problem of finding the work done by an external force in moving an electron from point B to point A, we can follow these steps:
1. Identify the Electric Potentials:
- The electric potential at point A (V_A) is given as 20 V.
- The electric potential at point B (V_B) is given as -20 V.
2. Understand the Work Done Formula:
- The work done (W) by an external force in moving a charge (Q) in an electric field is given by the formula:
W=Q⋅(VA−VB)
- Here, VA is the potential at point A, and VB is the potential at point B.
3. Determine the Charge of the Electron:
- The charge of an electron (Q) is approximately:
Q=−1.6×10−19C
4. Calculate the Potential Difference:
- Substitute the values of VA and VB into the formula for potential difference:
VA−VB=20−(−20)=20+20=40V
5. Calculate the Work Done:
- Now substitute the charge and the potential difference into the work done formula:
W=(−1.6×10−19)⋅(40)
- Performing the multiplication:
W=−6.4×10−18J
6. Conclusion:
- The work done by the external force in moving the electron from point B to point A is:
W=−6.4×10−18J
Final Answer:
The work done by an external force in moving the electron slowly from B to A is −6.4×10−18J.
---
- The electric potential at point A (V_A) is given as 20 V.
- The electric potential at point B (V_B) is given as -20 V.
- The work done (W) by an external force in moving a charge (Q) in an electric field is given by the formula:
W=Q⋅(VA−VB)
- Here, VA is the potential at point A, and VB is the potential at point B.
- The charge of an electron (Q) is approximately:
Q=−1.6×10−19C
- Substitute the values of VA and VB into the formula for potential difference:
VA−VB=20−(−20)=20+20=40V
- Now substitute the charge and the potential difference into the work done formula:
W=(−1.6×10−19)⋅(40)
- Performing the multiplication:
W=−6.4×10−18J
- The work done by the external force in moving the electron from point B to point A is:
W=−6.4×10−18J
The work done by an external force in moving the electron slowly from B to A is −6.4×10−18J.
Similar Questions
The electric potential at point A is 20V and at B is −40V. Find the work done by an external and electrostatic force in moving an electron slowly from B to A.
View SolutionA charge Q is placed at the origin. The electric potential due to this charge at a given point in space is V. The work done by an external force in bringing another charge q from infinity up to the point is
View Solution
Knowledge Check
A force F acting on an object varies with distance x as shown in the figure. The work done by the force in moving the object from x=0 to x = 20 m is
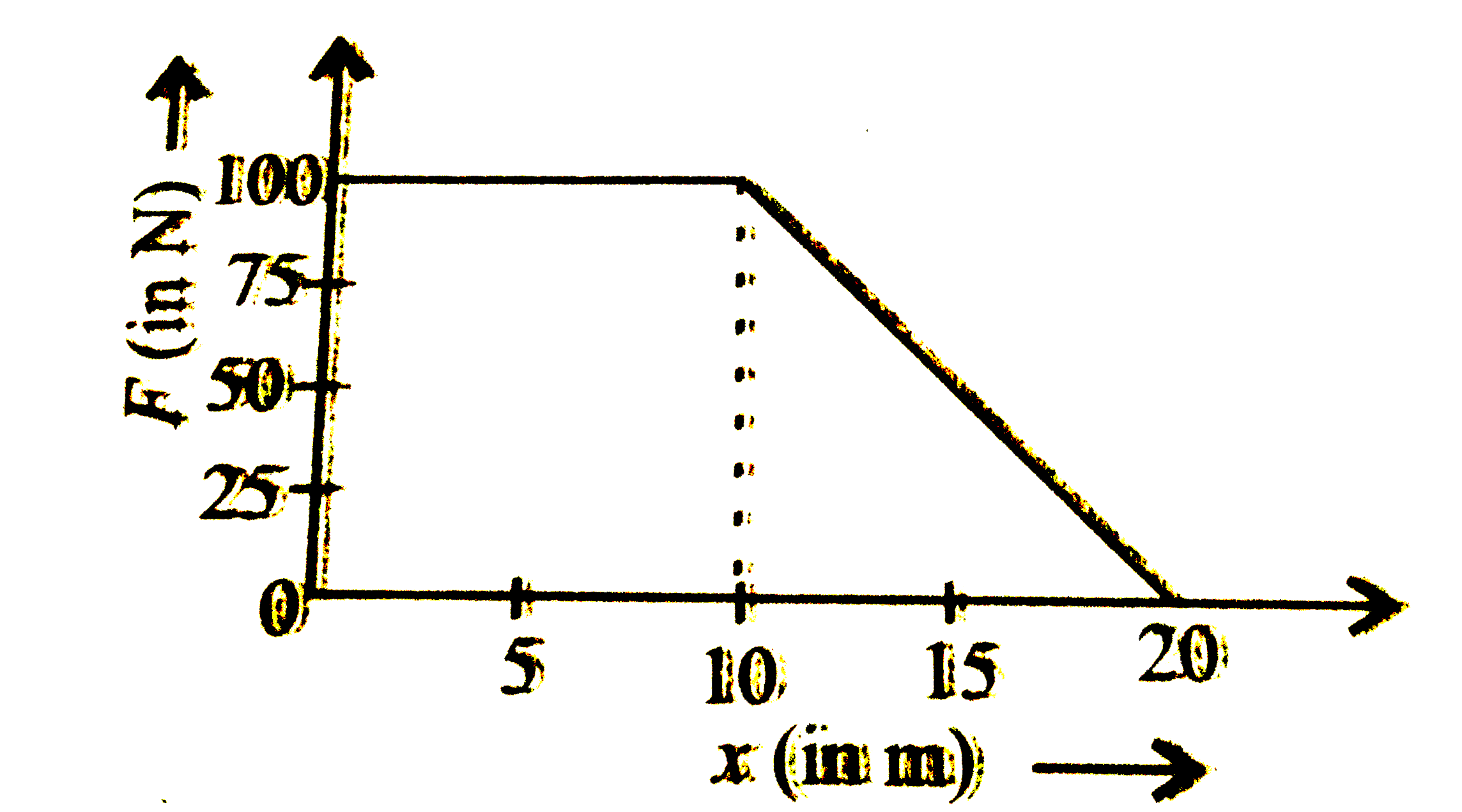 A500 JB1000 JC1500 JD2000 J
A500 JB1000 JC1500 JD2000 J
Potential at a point A is 3 volt and at a point B is 7V, an electron is moving towards A form B.
View SolutionA particle is moving in a conservative force field from point A to point B.UA and UB are the potential energies of the particle at point A and B and WC is the work done by conservative forces in the process of taking the particle from A and B, which of the following is true?
View Solution1μC charge is shifted from A to B, and it is found that work done by an external force is 40μJ. In doing so against electrostatic forces, find potential difference VA−VB.
View SolutionTwo points P and Q are maintained at the potentials of 10V and -4V, respectively. The work done in moving 100 electrons from P to Q is:
View SolutionFind out potential at P and Q due to the two point mass system Find out work done by external agent in bringing unit mass from P to Q Also find work done by gravitational force.
 .
.
View SolutionA body weights 500N on the surface of the earth. How much would it weights half-way below the surface of the earth?
View SolutionA small mass m is moved slowly from the surface of the earth to a height h above the surface. The work done (by an external agent) in doing this is
View Solution
