The electric potential at point A is 20V and at B is −40V. Find the work done by an external and electrostatic force in moving an electron slowly from B to A.
The correct Answer is:A, B
To find the work done by an external and electrostatic force in moving an electron slowly from point B to point A, we can follow these steps:
Step 1: Identify the electric potentials at points A and B
- The electric potential at point A (VA) is given as 20 V.
- The electric potential at point B (VB) is given as -40 V.
Step 2: Calculate the change in electric potential (ΔV)
- The change in electric potential when moving from B to A is calculated as:
ΔV=VA−VB=20V−(−40V)=20V+40V=60V
Step 3: Determine the charge of the electron
- The charge of an electron (q) is approximately:
q=−1.6×10−19 C
Step 4: Calculate the change in potential energy (ΔPE)
- The change in potential energy when moving the electron from B to A is given by:
ΔPE=q⋅ΔV=(−1.6×10−19 C)⋅(60 V)
- Performing the multiplication:
ΔPE=−1.6×60×10−19=−96×10−19 J=−9.6×10−18 J
Step 5: Calculate the work done by the external force (W_ext)
- The work done by the external force is equal to the change in potential energy:
Wext=ΔPE=−9.6×10−18 J
Step 6: Calculate the work done by the electrostatic force (W_elec)
- The work done by the electrostatic force is the negative of the change in potential energy:
Welec=−ΔPE=−(−9.6×10−18 J)=9.6×10−18 J
Final Results
- Work done by external force:
Wext=−9.6×10−18 J
- Work done by electrostatic force:
Welec=9.6×10−18 J
- The electric potential at point A (VA) is given as 20 V.
- The electric potential at point B (VB) is given as -40 V.
- The change in electric potential when moving from B to A is calculated as:
ΔV=VA−VB=20V−(−40V)=20V+40V=60V
- The charge of an electron (q) is approximately:
q=−1.6×10−19 C
- The change in potential energy when moving the electron from B to A is given by:
ΔPE=q⋅ΔV=(−1.6×10−19 C)⋅(60 V)
- Performing the multiplication:
ΔPE=−1.6×60×10−19=−96×10−19 J=−9.6×10−18 J
- The work done by the external force is equal to the change in potential energy:
Wext=ΔPE=−9.6×10−18 J
- The work done by the electrostatic force is the negative of the change in potential energy:
Welec=−ΔPE=−(−9.6×10−18 J)=9.6×10−18 J
- Work done by external force:
Wext=−9.6×10−18 J
- Work done by electrostatic force:
Welec=9.6×10−18 J
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Example Type 55videos
ELECTROSTATICS
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Example Type 64videos
ELECTROSTATICS
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises50videos
ELECTROSTATIC POTENTIAL AND CAPACITORS
- BOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions135videos
GRAVITATION
Similar Questions
The electric potential at. Point A is 20 V and B is – 20 V. The work done by an external force in moving electron slowly from B to A is
View SolutionA charge Q is placed at the origin. The electric potential due to this charge at a given point in space is V. The work done by an external force in bringing another charge q from infinity up to the point is
View SolutionPotential at a point A is 3 volt and at a point B is 7V, an electron is moving towards A form B.
View Solution1μC charge is shifted from A to B, and it is found that work done by an external force is 40μJ. In doing so against electrostatic forces, find potential difference VA−VB.
View SolutionFigures (a) and (b) show the field lines of a positive and negative point charge respectively.
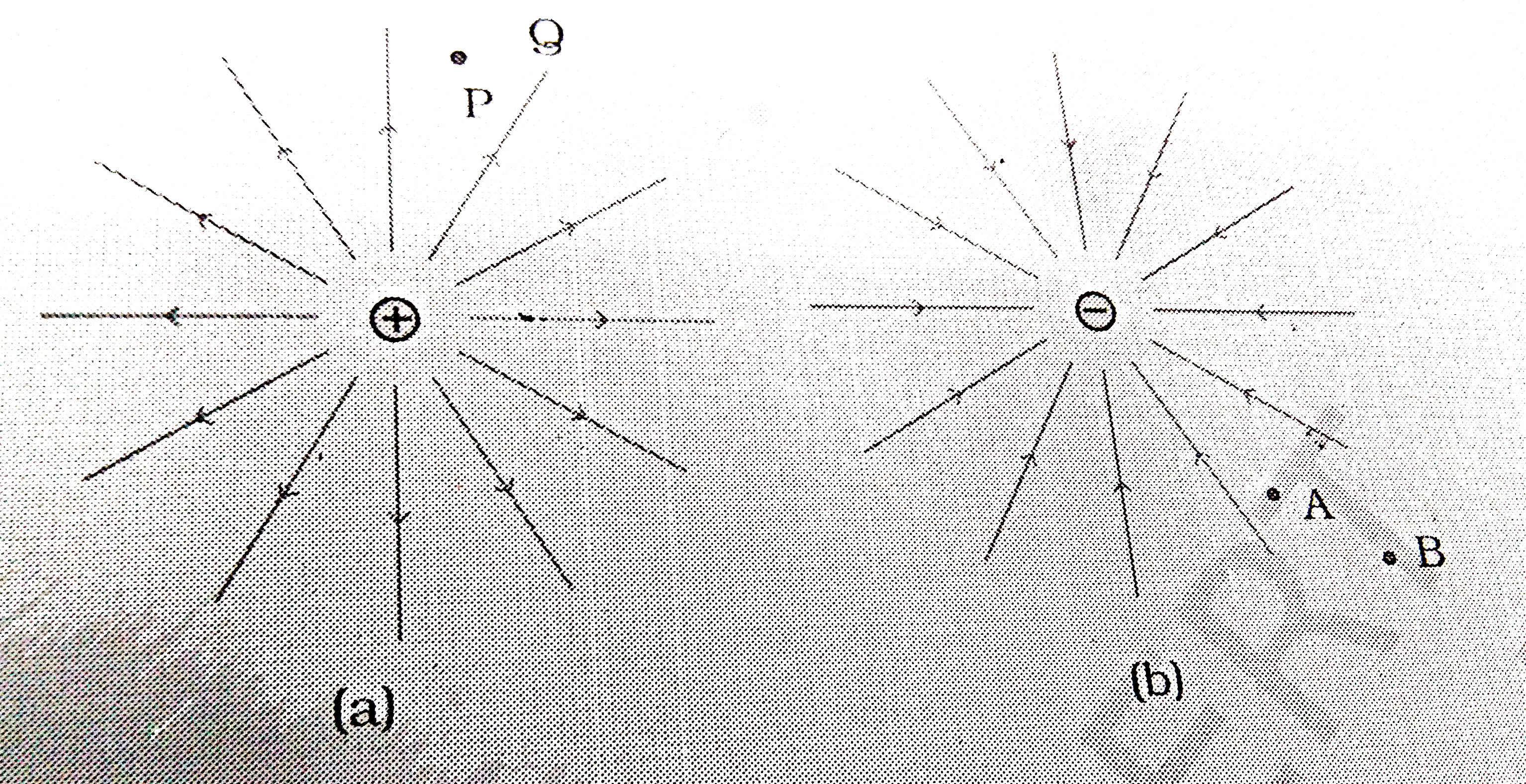
(a) Give the signs of the potential difference VP−VQ,VB−VA.
(b) Give the sign of the potential energy difference of a small negative charge between the points Q and P, A and B.
(c) Give the sign of the work done by the field in moving a small positive charge from Q to P.
(d) Give the sign of the work done by the external agency in moving a small negative charge from B to A.
(e) Does the kinetic energy of a small negative charge increase or decrease in going from B to A?View Solution1μC charge is shifted from A to B and it is found that work done by external force is 80μJ against electrostic forces, find VA−VB
View SolutionTwo points P and Q are maintained at the potentials of 10V and -4V, respectively. The work done in moving 100 electrons from P to Q is:
View SolutionA charge is moved in an electric field of a fixed charge distribution from point A to another point B slowly. The work done by external agent in doing so is 100J . What is the change in potential energy of the charge as it moves from A to B ? What is the work done by the electric field of the charge distribution as the charge moves from A to B ?
View SolutionA charge is moved in an electric field of a fixed charge distribution from point A to another point B slowly. The work done by external agent in doing so is 100J. What is the change in potential energy of the charge as it moves from A to B ? What is the work done by the electric field of the charge distribution as the charge moves from A to B ?
View SolutionA particle is moving in a conservative force field from point A to point B.UA and UB are the potential energies of the particle at point A and B and WC is the work done by conservative forces in the process of taking the particle from A and B, which of the following is true?
View Solution

