A slab consists of two parallel layers of copper and brass of the same thichness and having thermal conductivities in the ratio 1:4 If the free face of brass is at 100∘C anf that of copper at 0∘C the temperature of interface is .
(a)80∘C
(b)20∘C
(c)60∘C
(d)40∘C
The correct Answer is:A
To solve the problem step by step, we will use the concept of thermal conduction through two layers of materials with different thermal conductivities.
1. Identify the Given Data:
- Thermal conductivity of copper (K_c) = 1K
- Thermal conductivity of brass (K_b) = 4K
- Temperature of brass face (T_b) = 100°C
- Temperature of copper face (T_c) = 0°C
- Let the thickness of each layer be 'd'.
2. Set Up the Temperature Gradient:
- Let the temperature at the interface between the two materials be θ (in °C).
- The temperature difference across the brass layer is (T_b - θ) = (100 - θ)°C.
- The temperature difference across the copper layer is (θ - T_c) = (θ - 0)°C = θ°C.
3. Apply the Heat Conduction Equation:
- According to Fourier's law of heat conduction, the heat flow (Q) through each layer must be equal since they are in steady state.
- For brass:
Q=Kb⋅A⋅(Tb−θ)d=4K⋅A⋅(100−θ)d
- For copper:
Q=Kc⋅A⋅(θ−Tc)d=1K⋅A⋅θd
4. Equate the Heat Flow:
- Since both expressions for Q are equal:
4K⋅A⋅(100−θ)d=1K⋅A⋅θd
- The area (A) and thickness (d) cancel out, leading to:
4(100−θ)=θ
5. Solve for θ:
- Expanding the equation:
400−4θ=θ
- Rearranging gives:
400=5θ
- Therefore, solving for θ:
θ=4005=80°C
6. Conclusion:
- The temperature at the interface is θ = 80°C.
- Thermal conductivity of copper (K_c) = 1K
- Thermal conductivity of brass (K_b) = 4K
- Temperature of brass face (T_b) = 100°C
- Temperature of copper face (T_c) = 0°C
- Let the thickness of each layer be 'd'.
- Let the temperature at the interface between the two materials be θ (in °C).
- The temperature difference across the brass layer is (T_b - θ) = (100 - θ)°C.
- The temperature difference across the copper layer is (θ - T_c) = (θ - 0)°C = θ°C.
- According to Fourier's law of heat conduction, the heat flow (Q) through each layer must be equal since they are in steady state.
- For brass:
Q=Kb⋅A⋅(Tb−θ)d=4K⋅A⋅(100−θ)d
- For copper:
Q=Kc⋅A⋅(θ−Tc)d=1K⋅A⋅θd
- Since both expressions for Q are equal:
4K⋅A⋅(100−θ)d=1K⋅A⋅θd
- The area (A) and thickness (d) cancel out, leading to:
4(100−θ)=θ
- Expanding the equation:
400−4θ=θ
- Rearranging gives:
400=5θ
- Therefore, solving for θ:
θ=4005=80°C
- The temperature at the interface is θ = 80°C.
Similar Questions
A slab consists of two layers of different materials of the same thickness and having thermal conductivities K1 and K2. The equivalent thermal conductivity of the slab is
View SolutionTwo plates of equal area are placed in contact with each other. The thickness of the plates are 2.0 cm and 3.0 cm respectively. The outer face of first plate is at −25∘C and that of second plate is at +25∘C. The conductivities of the plates are in the ratio 2:3. Calculate the temperature of the common surface of the plates.
View Solution
Knowledge Check
The slab shown in the figure consists of two parallel layers of cooper anb brass of same thickness and having thermal conductivites in the ratio 4:1. IF the free face of the brass is at 100∘C and that of copper is at 0∘C, then the temperature of the interface will be
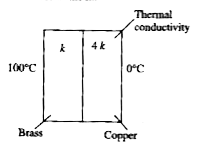 A20∘CB30∘CC40∘CD25∘C
A20∘CB30∘CC40∘CD25∘CTwo cylinders P and Q have the same length and diameter and are made of different materials having thermal conductivities in the ratio 2:3. These two cyinders ar combined to make a cylinder. One end of P is kept at 100∘C and another end of Q at 0∘C. THe temperature at the interface of P and Q is
A30∘CB40∘CC50∘CD60∘CA wall has two layers A and B, each made of different materials. Both the layers have the same thickness. The thermal conductivity of the material of A is twice that of B. Under thermal equilibrium , the temperature differeence across the wall is 36∘C. The temperature difference across the layer A is
A6∘CB12∘CC18∘CD24∘C
Three rods of the same dimensions have thermal conductivities 3k , 2k and k . They are arranged as shown, with their ends at 100∘C,50∘C and 0∘C. The temperature of their junction is :-
_E01_022_Q01.png)
View SolutionA slab consists of two parallel layers of iron and brass 10 cm and 10.9 cm thick and of thermal conductivities 500Wm−1K−1and109Wm−1K−1 respectively. The area of opposite faces are 2m2 each and are at temperatures 373 K and 273 K. Find heat conducted per second across the slab and also the temperature of the interface.
View SolutionTwo walls of thickness in the ratio 1:3 and thermal conductivities in the ratio 3:2 form a composite wall of a building. If the free surfaces of the wall be at temperatures 30∘C and 20∘C, respectively, what is the temperature of the interface?
View SolutionTwo rods of similar area of cross section and length are joined as shown in the figure. The ratio of their thermal conductivity is 2:3. The free ends are maintained at 100∘C and 0∘C respectivley. THE temperature at junction in steady state is
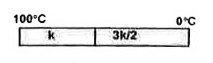
View SolutionTwo strings of copper are stretched to the same tension. If their cross-section area are in the ratio 1 : 4 , then the respective wave velocities will be
View Solution
