A current of 2.34 A flows in resistance of 11.111111Ω The potential difference across the given resistance with due regard for the significant figure is
(a)26.000 A
(b)26.00 A
(c)26.0 V
(d)26 V
The correct Answer is:C
To solve the problem step by step, we will use Ohm's Law, which states that the potential difference (V) across a resistor is equal to the current (I) flowing through it multiplied by the resistance (R).
Step 1: Identify the given values
- Current (I) = 2.34 A
- Resistance (R) = 11.111111 Ω
Step 2: Apply Ohm's Law
Using the formula:
V=I×R
Substituting the values we have:
V=2.34A×11.111111Ω
Step 3: Perform the multiplication
Calculating the product:
V=2.34×11.111111
V=25.99999974V
Step 4: Round off to significant figures
Now, we need to round the result to the correct number of significant figures. The current (2.34 A) has three significant figures, and the resistance (11.111111 Ω) has eight significant figures. Therefore, we should round the potential difference to three significant figures.
The value 25.99999974 rounded to three significant figures is:
V≈26.0V
Final Answer
The potential difference across the given resistance is:
26.0V
---
- Current (I) = 2.34 A
- Resistance (R) = 11.111111 Ω
Using the formula:
V=I×R
V=2.34A×11.111111Ω
Calculating the product:
V=2.34×11.111111
V=25.99999974V
Now, we need to round the result to the correct number of significant figures. The current (2.34 A) has three significant figures, and the resistance (11.111111 Ω) has eight significant figures. Therefore, we should round the potential difference to three significant figures.
V≈26.0V
The potential difference across the given resistance is:
26.0V
Similar Questions
A current 0.2A flows in a wire of resistance 15Ω. Find the potential difference across the ends of the wire.
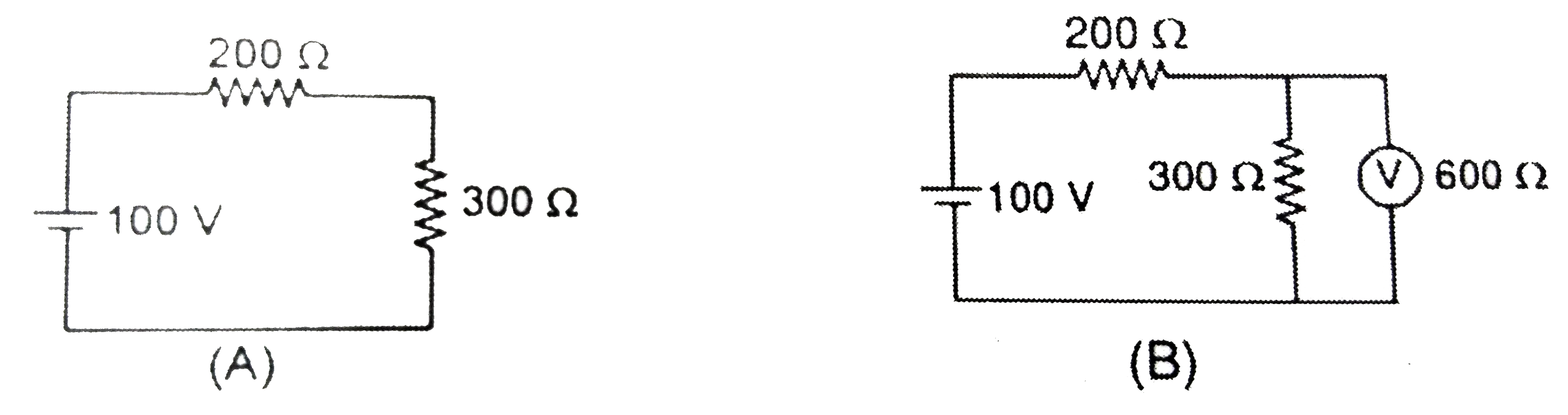
View SolutionFind potential difference across the resistance 300Ω in A and B.
View SolutionA current of 0.2 A flows through a wire whose ends are at a potential difference of 15 V. Calculate :
the resistance of the wire,
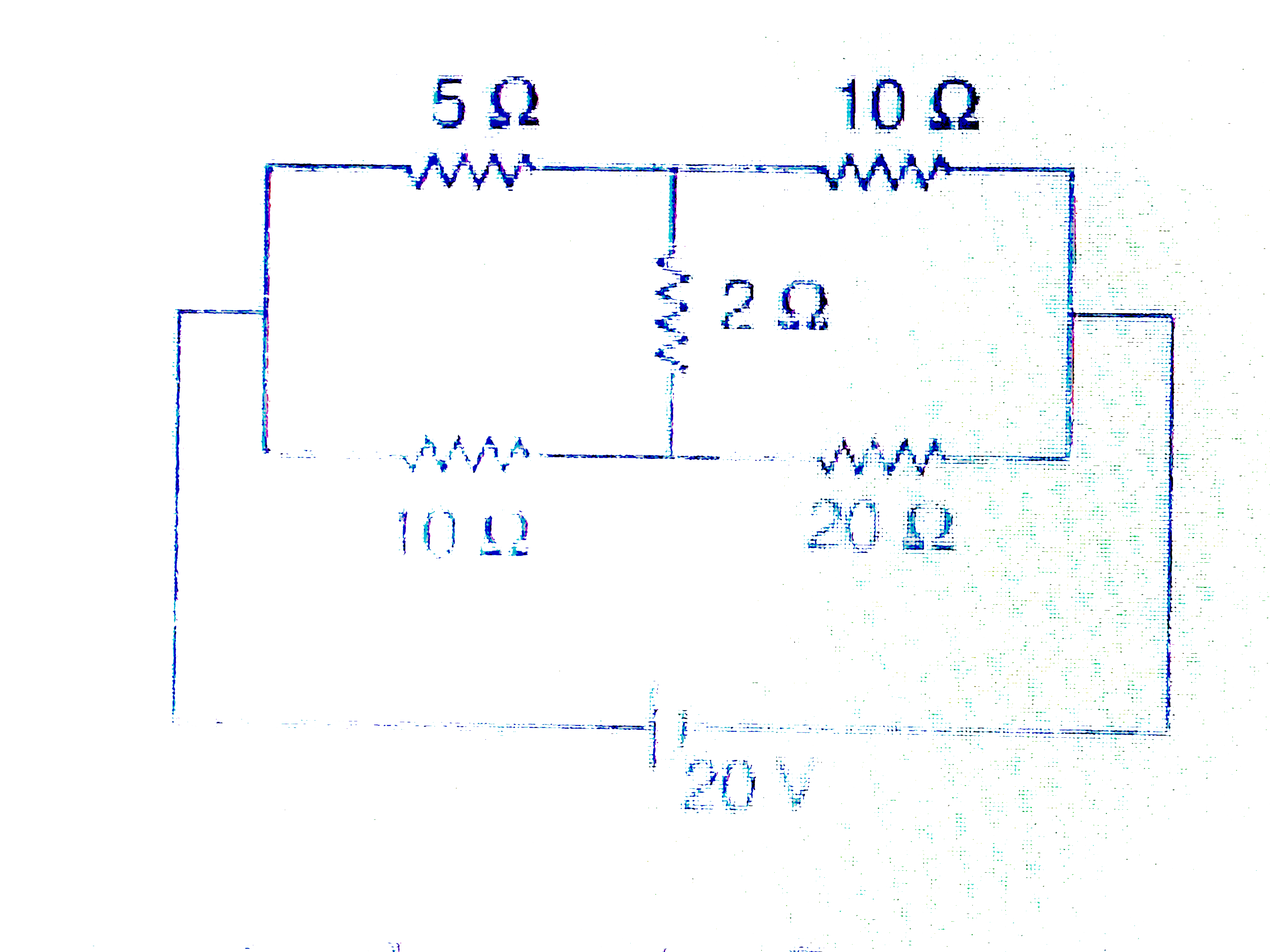
View SolutionIn the given circuit diagram find the potential difference across 2Ω resistance (in volts).
View SolutionA voltmeter of resistance 994Ω is connected across a cell of emf 1 V and internal resistance 6Ω. Find the potential difference across the voltmeter, that across the terminals of the cell and percantage error in the reading of the voltmeter.
View Solution100J of heat are produced each second in a 4Ω resistance. Find the potential difference across the resistor.
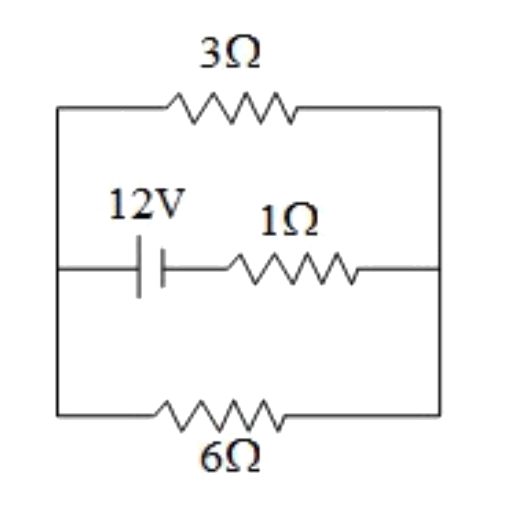
View SolutionThe current flowing in 3Ω Resistance will be
View SolutionIn the given circuit, if the potential difference across the internal resistance r1 is equal to E, then the resistance R is equal to:
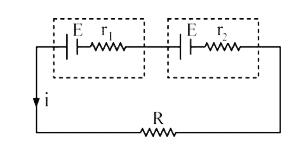
View SolutionThe resistance across P and Q in the given figure is

View SolutionCalculate the potential difference across the ends of a wire of resistance 2Ω'whenacurrentof1.5 A passes through it.
View Solution