A wire loop that encloses an area of 20cm2 has a resistance of 10Ω The loop is placed in a magnetic field of 2.4 T with its plane perpendicular to the field . The loop is suddenly removed from the field. How much charge flows past a given point in the wire ?
12×10−4C
3.6×10−1C
4.8×10−4C
2.4×10−3C
The correct Answer is:C
To solve the problem step by step, we will use Faraday's law of electromagnetic induction and the relationship between induced electromotive force (emf), current, resistance, and charge.
Step 1: Understand the problem
We have a wire loop with:
- Area A=20cm2=20×10−4m2
- Resistance R=10Ω
- Magnetic field B=2.4T
The loop is removed from the magnetic field, which means the magnetic flux through the loop changes.
Step 2: Calculate the initial magnetic flux
The magnetic flux Φ through the loop is given by:
Φ=B⋅A
Substituting the values:
Φinitial=2.4T×20×10−4m2=2.4×20×10−4=4.8×10−4Wb
Step 3: Determine the final magnetic flux
When the loop is removed from the magnetic field, the final magnetic flux Φfinal is:
Φfinal=0Wb
Step 4: Calculate the change in magnetic flux
The change in magnetic flux ΔΦ is:
ΔΦ=Φfinal−Φinitial=0−4.8×10−4=−4.8×10−4Wb
Step 5: Calculate the induced emf
According to Faraday's law, the induced emf E is equal to the negative rate of change of magnetic flux:
E=−ΔΦΔt
For the purpose of finding the charge, we can consider the magnitude:
E=|ΔΦ|Δt
Step 6: Calculate the current
The current I induced in the loop can be calculated using Ohm's law:
I=ER
Step 7: Relate current to charge
The charge Q that flows through the wire can be expressed as:
Q=I⋅Δt
Substituting I:
Q=ER⋅Δt
Step 8: Substitute for charge using change in flux
Since we are interested in the total charge that flows, we can express it as:
Q=|ΔΦ|R
Substituting the values:
Q=4.8×10−4Wb10Ω=4.8×10−5C
Final Answer
The total charge that flows past a given point in the wire is:
Q=4.8×10−5C
We have a wire loop with:
- Area A=20cm2=20×10−4m2
- Resistance R=10Ω
- Magnetic field B=2.4T
The magnetic flux Φ through the loop is given by:
Φ=B⋅A
Substituting the values:
Φinitial=2.4T×20×10−4m2=2.4×20×10−4=4.8×10−4Wb
When the loop is removed from the magnetic field, the final magnetic flux Φfinal is:
Φfinal=0Wb
The change in magnetic flux ΔΦ is:
ΔΦ=Φfinal−Φinitial=0−4.8×10−4=−4.8×10−4Wb
According to Faraday's law, the induced emf E is equal to the negative rate of change of magnetic flux:
E=−ΔΦΔt
For the purpose of finding the charge, we can consider the magnitude:
E=|ΔΦ|Δt
The current I induced in the loop can be calculated using Ohm's law:
I=ER
The charge Q that flows through the wire can be expressed as:
Q=I⋅Δt
Substituting I:
Q=ER⋅Δt
Since we are interested in the total charge that flows, we can express it as:
Q=|ΔΦ|R
Substituting the values:
Q=4.8×10−4Wb10Ω=4.8×10−5C
The total charge that flows past a given point in the wire is:
Q=4.8×10−5C
Similar Questions
A circular loop of area 1cm2 , carrying a current of 10 A , is placed in a magnetic field of 0.1 T perpendicular to the plane of the loop. The torque on the loop due to the magnetic field is
View SolutionA conducting square loop is placed in a magnetic field B with its plane perpendicular to the field. Now the sides of the loop start shrinking at a constant rate α. the induced emf in the loop at an instant when its side is a is
View SolutionA circular loop is placed in magnetic field B=2t. Find the direction of induced current produced in the loop.
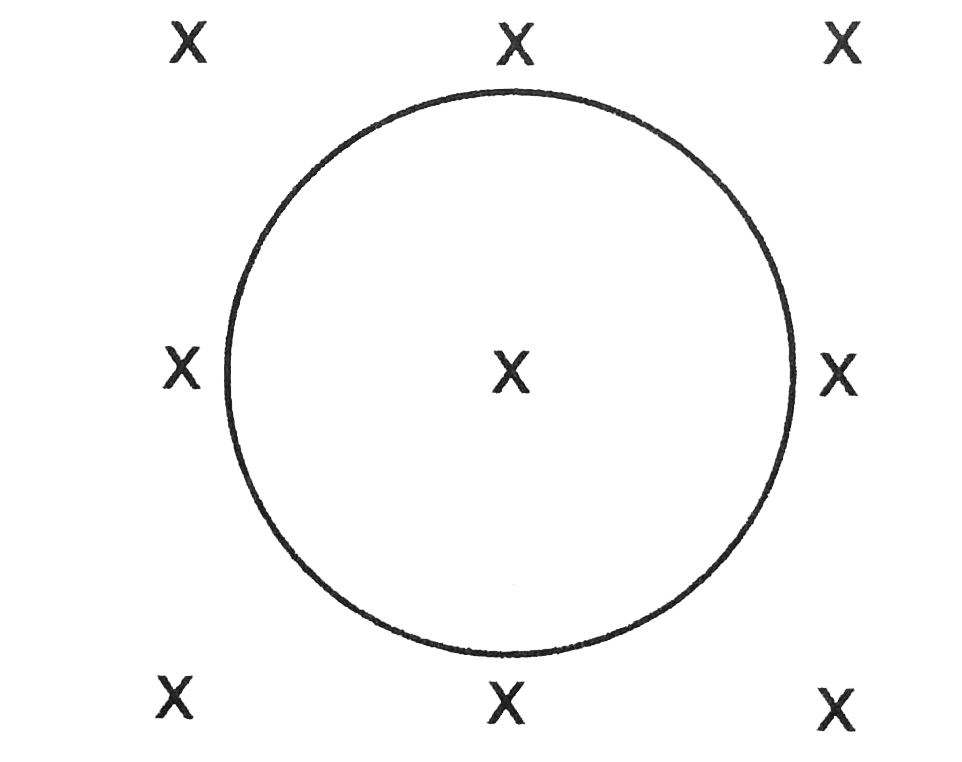
View SolutionA loop of Irregular shape made of flexible conducting wire carrying clockwise current is placed in uniform inward magnetic field, such that its plane is perpendicular to the field. Then the loop
View SolutionA conducting circular loop is placed is a uniform magnetic field B =0.20 T with its plane perpendicular to the field . Somehow, the radius of the loop starts shrinking at a constant rate of 1.0mms−1. Find the induced emf in the loop at an instant when the radius is 2 cm.
View SolutionA conducintg circular loop having a redius of 5.0cm, is placed perpendicular to a magnetic field of 0.50 T. It is removed from the field in 0.50 s. Find the average emf produced In the loop during this time.
View SolutionA current carrying circular loop of radius 50 mm, made of a very thin wire has inductance 0.26 μH and is placed in the uniform magnetic field 0,5 mT. The plane of the loop is perpendicular to the magnetic field lines. The resistance of loop can be neglected. Now the magnetic field has been turned off. Find the current in the loop (approximately)
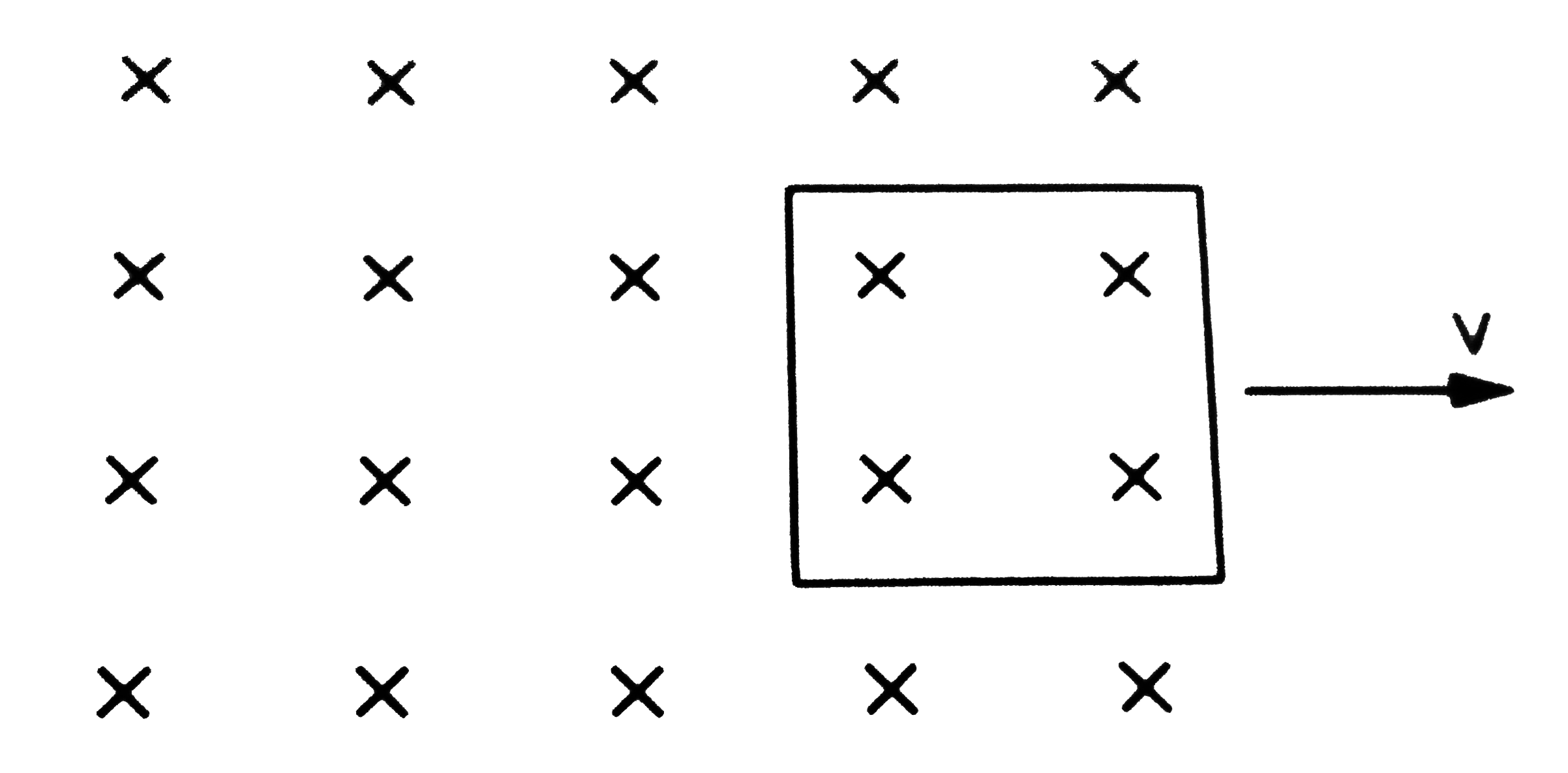
View SolutionShows a square loop having 100 turns, an area of 2.5X10−3m2 and a resistance of 100Ω. The magnetic field has a magnitude B =0.40 T. Find the work done in pulling the loop out of the field, slowly and uniformly is 1.0 s.
View SolutionA circular loop of radius R is kept in a uniform magnetic field pointing perpendicular into the plane of paper. When a current l flows in the loop, the tension produced in the loop is

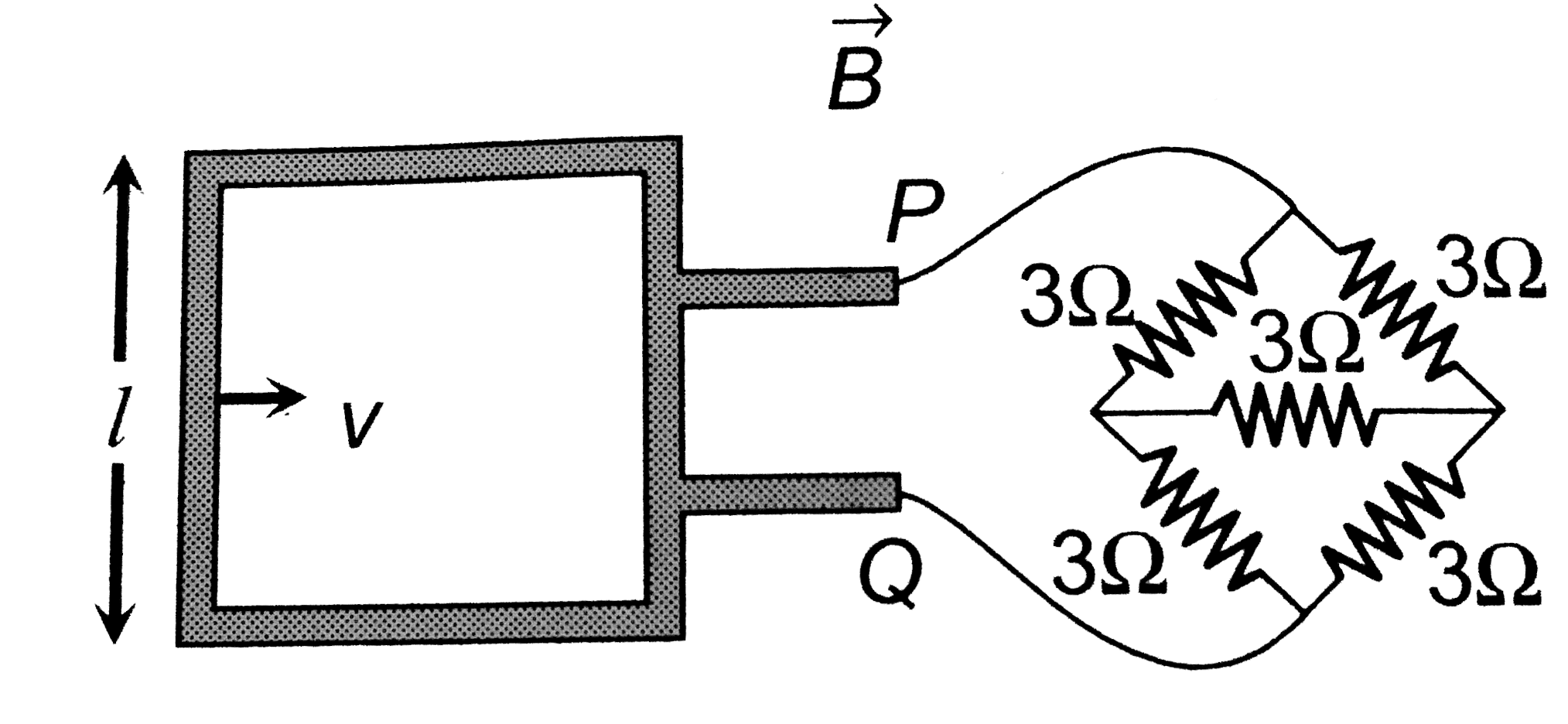
View SolutionA square metallic wire loop of side 0.1 m and resistance of 1Ω is moved with a constant velocity in a magnetic field of 2wb/m2 as shown in figure. The magnetic field field is perpendicular to the plane of the loop, loop is coonected to a network of resistances. what should be the velocity of loop so as to have a steady current of 1mA in loop?
View Solution
