A uniform meter scale of mass 1kg is placed on table such that a part of the scale is beyond the edge. If a body of mass 0.25kg is hung at the end of the scale then the minimum length of scale that should lie on the table so that it does not tilt is
90 cm
80 cm
70 cm
60 cm
The correct Answer is:D
To solve the problem, we need to determine the minimum length of the meter scale that must lie on the table to prevent it from tilting when a mass is hung at its end. We will use the principles of rotational equilibrium.
1. Identify the Forces and Distances:
- The mass of the scale (M) = 1 kg.
- The mass of the hanging body (m) = 0.25 kg.
- The gravitational force acting on the scale = M * g = 1 kg * 9.8 m/s² = 9.8 N.
- The gravitational force acting on the hanging mass = m * g = 0.25 kg * 9.8 m/s² = 2.45 N.
- Let the length of the scale that lies on the table be denoted as L (in meters).
- The length of the scale that is beyond the edge of the table is 1−L (since the total length of the scale is 1 meter).
2. Determine the Center of Mass:
- The center of mass of the uniform meter scale is located at its midpoint, which is at 0.5 m from one end.
- If L is the length of the scale on the table, then the distance from the edge of the table to the center of mass of the scale is L−0.5 m.
3. Set Up the Rotational Equilibrium Condition:
- For the scale to be in rotational equilibrium, the clockwise moments about the edge of the table must equal the counterclockwise moments.
- The moment due to the hanging mass is:
Moment due to hanging mass=m⋅g⋅(1−L)
- The moment due to the weight of the scale is:
Moment due to scale=M⋅g⋅(L−0.5)
- Setting these moments equal gives:
0.25⋅9.8⋅(1−L)=1⋅9.8⋅(L−0.5)
4. Simplify the Equation:
- Cancel out 9.8 from both sides:
0.25(1−L)=(L−0.5)
- Expanding both sides:
0.25−0.25L=L−0.5
5. Rearranging the Equation:
- Bringing all terms involving L to one side:
0.25+0.5=L+0.25L
0.75=1.25L
6. Solving for L:
- Dividing both sides by 1.25:
L=0.751.25=0.6 m=60 cm
7. Conclusion:
- The minimum length of the scale that should lie on the table to prevent it from tilting is 60 cm.
- The mass of the scale (M) = 1 kg.
- The mass of the hanging body (m) = 0.25 kg.
- The gravitational force acting on the scale = M * g = 1 kg * 9.8 m/s² = 9.8 N.
- The gravitational force acting on the hanging mass = m * g = 0.25 kg * 9.8 m/s² = 2.45 N.
- Let the length of the scale that lies on the table be denoted as L (in meters).
- The length of the scale that is beyond the edge of the table is 1−L (since the total length of the scale is 1 meter).
- The center of mass of the uniform meter scale is located at its midpoint, which is at 0.5 m from one end.
- If L is the length of the scale on the table, then the distance from the edge of the table to the center of mass of the scale is L−0.5 m.
- For the scale to be in rotational equilibrium, the clockwise moments about the edge of the table must equal the counterclockwise moments.
- The moment due to the hanging mass is:
Moment due to hanging mass=m⋅g⋅(1−L)
- The moment due to the weight of the scale is:
Moment due to scale=M⋅g⋅(L−0.5)
- Setting these moments equal gives:
0.25⋅9.8⋅(1−L)=1⋅9.8⋅(L−0.5)
- Cancel out 9.8 from both sides:
0.25(1−L)=(L−0.5)
- Expanding both sides:
0.25−0.25L=L−0.5
- Bringing all terms involving L to one side:
0.25+0.5=L+0.25L
0.75=1.25L
- Dividing both sides by 1.25:
L=0.751.25=0.6 m=60 cm
- The minimum length of the scale that should lie on the table to prevent it from tilting is 60 cm.
Similar Questions
A uniform chain of mass 4 kg and length 2 m is kept on table such that 3/10th of the chain hanges freely from the edge of the table. How much work has to be done in pulling the entire chain on the table?
View SolutionA uniform chain of mass m and length I is kept on the table with a part of it overhanging (see figure). If the coefficient of friction between the table and the chain is 1/3 then find the maximum length of the chain that can overhang such that the chain remain in equilibrium.
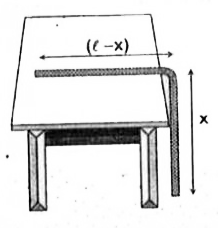
View Solution
Knowledge Check
A uniform chain of length 2m is kept on a table such that a length of 60cm hangs freely from the edge of the table . The total mass of the chain is 4kg What is the work done in pulling the entire the chain the on the table ?
A12.9JB6.3 JC3.6 JD2.0 J
A uniform cable of mass 'M' and length 'L' is placed on a horizontal surface such that its (1n)th part is hanging below the edge of the cable upto the surface, the work done should be :
View SolutionA uniform chain of length 4m is kept on a table such that a length of 120 cm hangs freely from the edge of the table. The total mass of the chain is 4 kg What is the work done in pulling the entire chain on the table ?
View SolutionA uniform chain of mass m and length l hangs on a thread and touches the surface of a table by its lower end. Thread breaks suddenly. Find the force exerted by the table on the chain when half of its length has fallen on the table. The fallen part does not form heap
View SolutionA uniform chain of mass m and length l hanges on a thread and touches the surface of a table by its lower end. Find the force exerted by the table on the chain when half of its length has fallen on the table. The fallen part does not form heap.

View SolutionA uniform metre scale can be balanced at the 70.0 cm mark when a mass of 0.05 kg is hung from the 94.0 cm mark.
Find the mass of the metre scale.
View SolutionA body of mass 20 kg is resting on a table. A light string is attached to it and passed over a smooth pulley at the edge of the table and a mass 3kg is attached at the free end. What is the acceleration of the system of the coefficient of kinetic friction between the mass and the table is 0.04 ? What is the tension in the string ?
View SolutionA uniform chain of length l and mass m is placed on a smooth table with one-fourth of its length hanging over the edge. The work that has to be done to pull the whole chain back onto the table is :
View Solution
