A charge Q is uniformly distributed over the surface of two conducting concentric spheres of radii R and r (Rgtr). Then, potential at common centre of these spheres is
KQ(R+r)Rr
KQ(R+r)(R2+r2)
KQ√R2+r2
KQ(1R−1r)
The correct Answer is:B
To find the potential at the common center of two concentric conducting spheres with radii R and r (where R>r), we can follow these steps:
Step 1: Understand the Charge Distribution
We have a total charge Q uniformly distributed over the surfaces of both spheres. Let:
- Q1 be the charge on the inner sphere (radius r)
- Q2 be the charge on the outer sphere (radius R)
We know that:
Q1+Q2=Q
Step 2: Calculate the Surface Charge Densities
The surface charge density σ for both spheres can be defined as:
- For the inner sphere:
σ1=Q14πr2
- For the outer sphere:
σ2=Q24πR2
Step 3: Express Charges in Terms of Charge Densities
From the surface charge densities, we can express Q1 and Q2:
- Q1=σ1⋅4πr2
- Q2=σ2⋅4πR2
Step 4: Write the Potential at the Center
The potential V at the common center O due to a charged sphere is given by:
- For the inner sphere:
V1=kQ1r
- For the outer sphere:
V2=kQ2R
Thus, the total potential at point O is:
VO=V1+V2=kQ1r+kQ2R
Step 5: Substitute Q1 and Q2 in Terms of Q
Using Q1+Q2=Q, we can express VO in terms of Q:
VO=k(Q1r+Q2R)
Step 6: Find Q1 and Q2 in Terms of Q
From the charge density equations:
Q1=σ1⋅4πr2=Q4π(r2+R2)⋅4πr2=Qr2r2+R2
Q2=σ2⋅4πR2=Q4π(r2+R2)⋅4πR2=QR2r2+R2
Step 7: Substitute Back into the Potential Equation
Now substituting Q1 and Q2 into the potential equation:
VO=k(Qr2r(r2+R2)+QR2R(r2+R2))
Step 8: Simplify the Expression
This simplifies to:
VO=kQ(r+Rr2+R2)
Final Answer
Thus, the potential at the common center of the spheres is:
VO=kQ(r+R)r2+R2
We have a total charge Q uniformly distributed over the surfaces of both spheres. Let:
- Q1 be the charge on the inner sphere (radius r)
- Q2 be the charge on the outer sphere (radius R)
Q1+Q2=Q
The surface charge density σ for both spheres can be defined as:
- For the inner sphere:
σ1=Q14πr2
- For the outer sphere:
σ2=Q24πR2
From the surface charge densities, we can express Q1 and Q2:
- Q1=σ1⋅4πr2
- Q2=σ2⋅4πR2
The potential V at the common center O due to a charged sphere is given by:
- For the inner sphere:
V1=kQ1r
- For the outer sphere:
V2=kQ2R
VO=V1+V2=kQ1r+kQ2R
Using Q1+Q2=Q, we can express VO in terms of Q:
VO=k(Q1r+Q2R)
From the charge density equations:
Q1=σ1⋅4πr2=Q4π(r2+R2)⋅4πr2=Qr2r2+R2
Q2=σ2⋅4πR2=Q4π(r2+R2)⋅4πR2=QR2r2+R2
Now substituting Q1 and Q2 into the potential equation:
VO=k(Qr2r(r2+R2)+QR2R(r2+R2))
This simplifies to:
VO=kQ(r+Rr2+R2)
Thus, the potential at the common center of the spheres is:
VO=kQ(r+R)r2+R2
Similar Questions
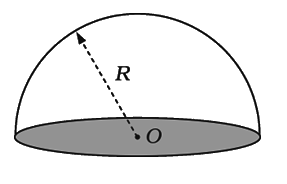
Charge Q is uniformly distributed throughout the volume of a solid hemisphere of radius R metres. Then the potential at centre O of hemisphere in volts is:
View SolutionA charge Q has been divided on two concentirc conducting spheres of radii R1andR2(R1>R2) such that the surface charge densities on both the sphere is same. Find the potential at their common center.
View SolutionA point charge q is placed at a distance of r from cebtre of an uncharged conducting sphere of rad R(<r). The potential at any point on the sphere is
View SolutionA charge Q is distributed over two concentric hollow spheres of radii r and R(>r) such that the surface charge densities are equal. Find the potential at the common centre.
View SolutionA charge Q is distributed over two concentric hollow spheres of radii r and R(>r) such that the surface charge densities are equal. Find the potential at the common centre.
View SolutionA charge Q is distributed over two concentric hollow spheres of radii r and R(>r) such that the surface charge densities are equal. Find the potential at the common centre.
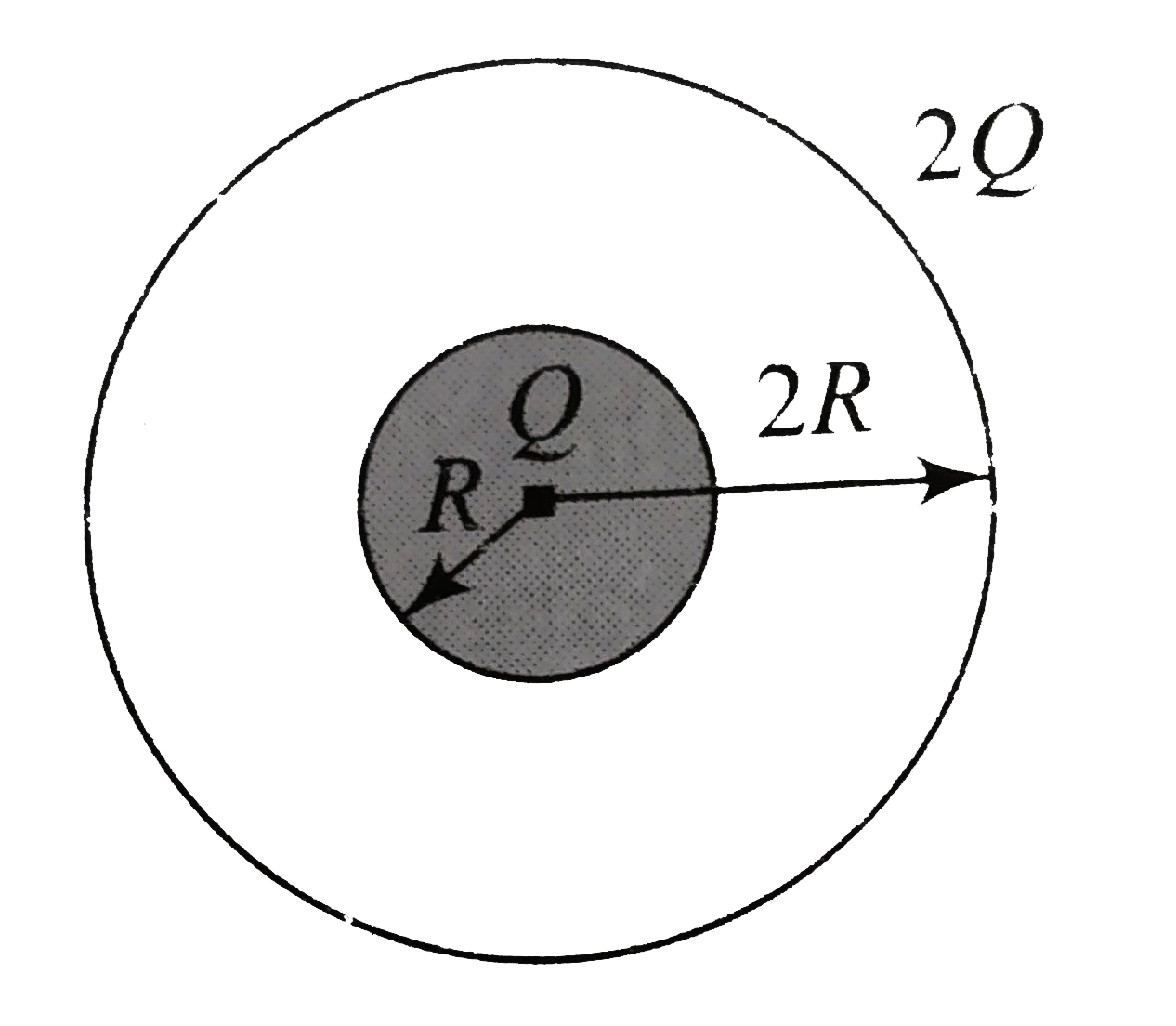
View SolutionA charge Q is uniformly distributed in a dielectric sphere of radius R (having dielectric constant unity). This dielectric sphere is enclosed by a concentric spherical shell of radius 2R and having uniformly distributed charge 2Q. Which of the following graph correctly represents variation of electric field with distance r from the common centre?
View SolutionA charge q is uniformly distributed over a quarter circular ring of radius r. The magnitude of electric field strength at the centre of the ring will be
View SolutionA charge .q. is distrubuted over two concertric hollow conducting sphere of radii r and R (<r) such that their surface charge densite are equal. The potential at their common centre is
View SolutionA charge q is distributed uniformly on the surface of a sold sphere of radius R. It covered by a concentric hollow conduction sphere of radius 2R. Find the charges inner and outer surface of hollow sphere if it is earthed.

View Solution
