A particle is revolving in a circular path as shown in figure in the horizontal plane such that the angular velocity of the particle about the point O is constant and is equal to 1rad/s. Distance of the particle from O is given byR=R0−βt where R0and β are constant. The speed of the particle as a function of time is: 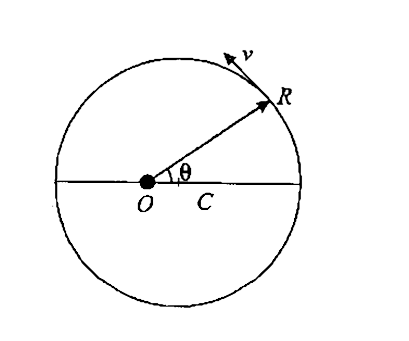
√β2+1
(R0−βt)
√β2+(R0−βt)2
β
The correct Answer is:C
Step by step video & image solution for A particle is revolving in a circular path as shown in figure in the horizontal plane such that the angular velocity of the particle about the point O is constant and is equal to 1 rad//s. Distance of the particle from O is given byR=R_(0)-betat where R_(0)and beta are constant. The speed of the particle as a function of time is: by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
A particle is revolving in a circular path of radius 2 m with constant angular speed 4 rad/s. The angular acceleration of particle is
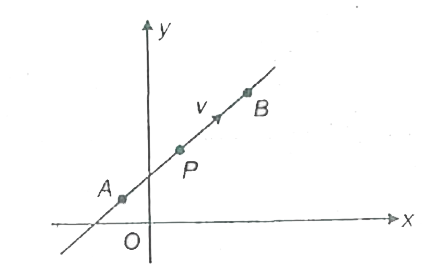
View SolutionA particle P is moving along a straight line as shown in the figure. During the motion of the particle from A to B the angular momentum of the particle about O
 View Solution
View SolutionA particle is moving with constant speed v in xy plane as shown in figure. The magnitude of its angular velocity about point O is
 View Solution
View SolutionA particle mass parallel to x-axis with constant velocity v as shown in the figure. The angular velocity of the particle about the origin O

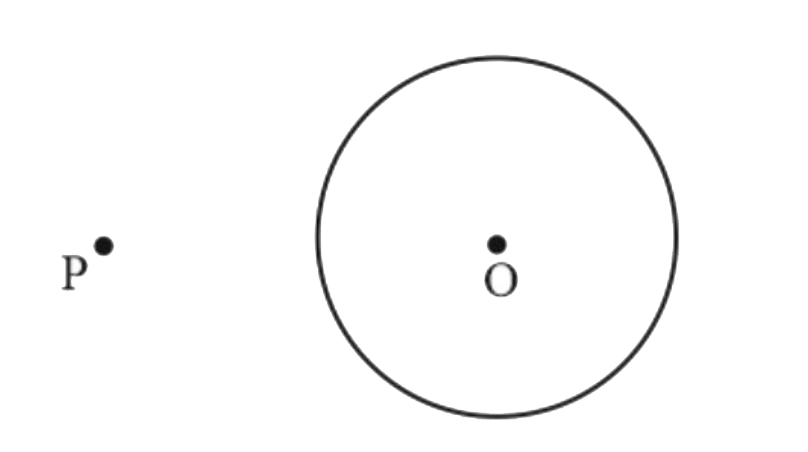
View SolutionConsider a particle , moving in a circle with constant speed . P is a point outside the circle , in the same plane . Then , the angular momentum of the particle about the point P is
 View Solution
View SolutionA particle is moving in a circular path of radius r under the action of a force F. If at an instant velocity of particle is v, and speed of particle is increasing, then
View SolutionA particle is moving with constant speed v along x - axis in positive direction. Find the angular velocity of the particle about the point (0, b), when position of the particle is (a, 0).
View Solution
A particle is moving on a circular path of radius r with uniform velocity v. The change in velocity when the particle moves from P to Q is (∠POQ=40∘)View SolutionA particle revolving in a circular path completes first one third of circumference in 2 sec, while next one third in 1 sec. The average angular velocity of particle will be – ("in "rad//sec)
View SolutionA particle moves in a circular path with constant speed .Find out a point about which the angular momentum of the particle is constant and another point about which changes with time.
View Solution

