Select the correct relation between azimuthal quantum number (l) and magnetic quantum (m)
l=m−12
m=1+2
m=2l2+1
l=2m+1
The correct Answer is:A
To solve the question regarding the relationship between the azimuthal quantum number (l) and the magnetic quantum number (m), we can follow these steps:
Step 1: Understand the Definitions
- The azimuthal quantum number (l) determines the shape of the orbital and can take on integer values from 0 to n-1, where n is the principal quantum number.
- The magnetic quantum number (m) describes the orientation of the orbital in space and can take on integer values from -l to +l, including 0.
Step 2: Determine the Range of m
- For a given value of l, the magnetic quantum number m can take values:
m=−l,−l+1,...,0,...,l−1,l
- This means that the total number of possible values for m is given by:
Total values of m=2l+1
Step 3: Establish the Relationship
- From the above, we can derive that for any given l:
m can take values from −l to +l
- Therefore, the relationship can be expressed as:
l=m−12
or alternatively,
m=2l+1
Step 4: Conclusion
- Based on the derived relationships, we can conclude that the correct relation between the azimuthal quantum number (l) and the magnetic quantum number (m) is:
m=2l+1
Step 5: Check Options
- After establishing the relationship, we can check the provided options to find the correct one. According to the solution, Option A is the correct choice.
Final Answer
- The correct relation between the azimuthal quantum number (l) and the magnetic quantum number (m) is:
m=2l+1
- The azimuthal quantum number (l) determines the shape of the orbital and can take on integer values from 0 to n-1, where n is the principal quantum number.
- The magnetic quantum number (m) describes the orientation of the orbital in space and can take on integer values from -l to +l, including 0.
- For a given value of l, the magnetic quantum number m can take values:
m=−l,−l+1,...,0,...,l−1,l
- This means that the total number of possible values for m is given by:
Total values of m=2l+1
- From the above, we can derive that for any given l:
m can take values from −l to +l
- Therefore, the relationship can be expressed as:
l=m−12
or alternatively,
m=2l+1
- Based on the derived relationships, we can conclude that the correct relation between the azimuthal quantum number (l) and the magnetic quantum number (m) is:
m=2l+1
- After establishing the relationship, we can check the provided options to find the correct one. According to the solution, Option A is the correct choice.
- The correct relation between the azimuthal quantum number (l) and the magnetic quantum number (m) is:
m=2l+1
Similar Questions
Quantum Numbers
View SolutionAzimuthal quantum number defines.
View SolutionAzimuthal quantum number determines the
View SolutionThe ground state energy of hydrogen atom is −13.6eV. Consider an electronic state Ψ of He+ whose energy, azimuthal quantum number and magnetic quantum number are −3.4eV,2 and 0, respectively. Which of the following statement(s) is(are) true for the state Ψ?
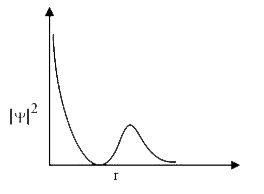
View SolutionThe graph for an orbital between |φ|2 and r(radial distance) is shown below. The sum of principle quantum number, azimuthal, quantum number and magnetic quantum number of this orbital is ____________.
View SolutionFor the principal quantum number n = 2, the possible values of azimuthal quantum number and magnetic quantum number respectively are
View SolutionAn electron in an atom can be completely designated with the help of four quantum numbers. Out of these, the first three i.e., principal (n), azimuthal (l) and magnetic (m) quantum number are obtained from the solution of Shrodinger wave equation while the spin(s) quantum number arises from the spin of the electron around its axis clockwise or anticlockwise. Of these principal quantum number tells about the size, azimuthal quantum number about the shape and magnetic quantum signifies the orientation of the electron orbital.
How many electrons in a given atom have the following set of quantum numbers?
n=3,l=2,m=+2,s=−1/2
View SolutionAn electron in an atom can be completely designated with the help of four quantum numbers. Out of these, the first three i.e., principal (n), azimuthal (l) and magnetic (m) quantum number are obtained from the solution of Schrödinger wave equation while the spin(s) quantum number arises from the spin of the electron around its axis clockwise or anticlockwise. Of these principal quantum number tells about the size, azimuthal quantum number about the shape and magnetic quantum signifies the orientation of the electron orbital.
The electronic configuration of P in H3PO4 is
View SolutionAccording to the bohr's atomic model, the relation between principal quantum number (n) and radius of orbit (r) is
View SolutionPrincipal quantum number is related to
View Solution
