The temperature of 20 L of nitrogen was increased from 10 K to 30 K at a constant pressure. Change in volume will be
20 L
40 L
60 L
80 L
The correct Answer is:B
To solve the problem, we will use the direct relationship between volume and temperature at constant pressure, which is given by Charles's Law. According to Charles's Law, the volume of a gas is directly proportional to its absolute temperature when pressure is held constant.
1. Identify the Initial Conditions:
- Initial Volume (V1) = 20 L
- Initial Temperature (T1) = 10 K
- Final Temperature (T2) = 30 K
2. Use Charles's Law:
Charles's Law states that:
V1T1=V2T2
Where:
- V1 = initial volume
- T1 = initial temperature
- V2 = final volume
- T2 = final temperature
3. Substitute Known Values:
Plugging in the known values into the equation:
20L10K=V230K
4. Cross-Multiply to Solve for V2:
20L×30K=V2×10K
600=10V2
V2=60010=60L
5. Calculate the Change in Volume:
The change in volume (ΔV) can be calculated as:
ΔV=V2−V1
ΔV=60L−20L=40L
6. Conclusion:
The change in volume is 40 L.
Final Answer:
The change in volume will be 40 L.
- Initial Volume (V1) = 20 L
- Initial Temperature (T1) = 10 K
- Final Temperature (T2) = 30 K
Charles's Law states that:
V1T1=V2T2
Where:
- V1 = initial volume
- T1 = initial temperature
- V2 = final volume
- T2 = final temperature
Plugging in the known values into the equation:
20L10K=V230K
20L×30K=V2×10K
600=10V2
V2=60010=60L
The change in volume (ΔV) can be calculated as:
ΔV=V2−V1
ΔV=60L−20L=40L
The change in volume is 40 L.
The change in volume will be 40 L.
Similar Questions
Knowledge Check
A sample of gas has a volume of V1 litre at temperature t1.∘C. When the temperature of the gas is changed to t2.∘C at constant pressure, then the volume of the gas was found to increase by 10%. The percentage increase in temperature is
A0.1B(10+2730t1)%C20%D(0.1+t−11)%
Forty calories of heat is needed to raise the temperature of 1 mol of an ideal monatomic gas from 20∘C to 30∘C at a constant pressure. The amount of heat required to raise its temperature over the same interval at a constant volume (R=2calmol−1K−1) is
View Solution600 mL of nitrogen at 30 K is cooled to 5 K at the same pressure. Calculate the new volume.
View SolutionCalculate the maximum work down when pressure on 10g of hydrogen is reduced from 20 to 1atm at a constant temperature of 273K. The gas behaves ideally. Will there be any change in internal energy. Also calculate q.(R=2calK−1mol−1)
View SolutionWhat happens to the pressure of a sample of helium gas if the temperature is increased from 200 K to 800 K, with no change in volume?
View SolutionCan the temperature of a gas increased keeping its pressure and volume constant ?
View SolutionThe volume of a gas at 20∘C is 200 ml. If the temperature is reduced to −20∘C at constant pressure, its volume will be :-
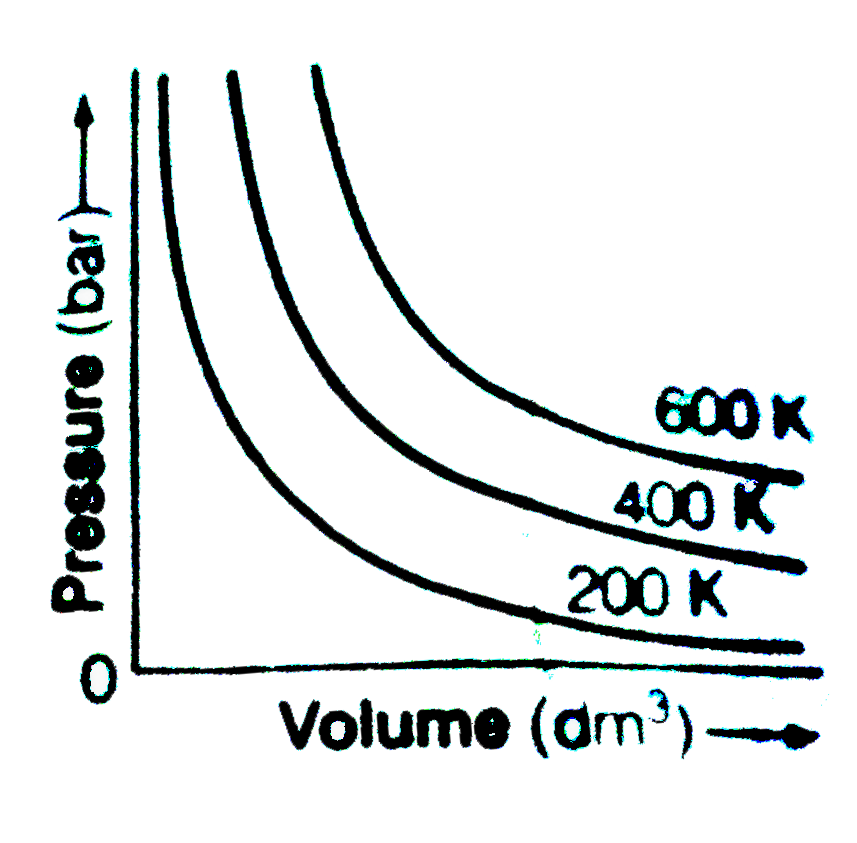
View SolutionThe variation of pressure with volume of the gas at different temperatures can be graphically represented as shown in figure. On the basis of this graph answer the following question.
(i) How will the volume of a gas change if its pressure is increased at constant temperature ?
(ii) At a constant pressure, how will the volume of a gas change if the temperature is increased from 200 K to 400 K ?
View Solution
