The temperature of 20 litres of nitrogen was increased from 100 K to 300 K at a constant pressure. Change in volume will be
80 litre
60 litre
40 litre
20 litre
The correct Answer is:C
To solve the problem of finding the change in volume of nitrogen gas when its temperature is increased from 100 K to 300 K at constant pressure, we can use Charles's Law. Here’s a step-by-step solution:
Step 1: Understand Charles's Law
Charles's Law states that at constant pressure, the volume of a gas is directly proportional to its absolute temperature. Mathematically, it can be expressed as:
V1T1=V2T2
where:
- V1 = initial volume
- T1 = initial temperature
- V2 = final volume
- T2 = final temperature
Step 2: Identify the Given Values
From the problem, we have:
- V1=20 liters
- T1=100 K
- T2=300 K
Step 3: Substitute the Values into Charles's Law
We can substitute the known values into the equation:
20100=V2300
Step 4: Solve for V2
Cross-multiply to solve for V2:
20×300=100×V2
6000=100V2
Now, divide both sides by 100:
V2=6000100=60 liters
Step 5: Calculate the Change in Volume
The change in volume (ΔV) is calculated as:
ΔV=V2−V1
Substituting the values we found:
ΔV=60 liters−20 liters=40 liters
Final Answer
The change in volume is 40 liters.
---
Charles's Law states that at constant pressure, the volume of a gas is directly proportional to its absolute temperature. Mathematically, it can be expressed as:
V1T1=V2T2
where:
- V1 = initial volume
- T1 = initial temperature
- V2 = final volume
- T2 = final temperature
From the problem, we have:
- V1=20 liters
- T1=100 K
- T2=300 K
We can substitute the known values into the equation:
20100=V2300
Cross-multiply to solve for V2:
20×300=100×V2
6000=100V2
Now, divide both sides by 100:
V2=6000100=60 liters
The change in volume (ΔV) is calculated as:
ΔV=V2−V1
Substituting the values we found:
ΔV=60 liters−20 liters=40 liters
The change in volume is 40 liters.
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - STATES OF MATTEREXERCISE - PRACTICE EXERCISE-13videos
STATES OF MATTER
- BOOK - VMC MODULES ENGLISHCHAPTER - STATES OF MATTEREXERCISE - PRACTICE EXERCISE-23videos
STATES OF MATTER
- BOOK - VMC MODULES ENGLISHCHAPTER - STATES OF MATTEREXERCISE - ILLUSTRATION30videos
STATES OF MATTER
- BOOK - VMC MODULES ENGLISHCHAPTER - STATES OF MATTEREXERCISE - IMPECCABLE50videos
STATES OF MATTER
- BOOK - VMC MODULES ENGLISHCHAPTER - STOICHIOMETRY - IEXERCISE - JEE Advanced (Archive)31videos
STOICHIOMETRY - I
Similar Questions
The temperature of 20 L of nitrogen was increased from 10 K to 30 K at a constant pressure. Change in volume will be
View SolutionThe temperature of a given mass of a gas is increased from 19∘C to 20∘C at constant pressure. The volume V of the gas is
View SolutionTemperature of 4 moles of gas increases from 300 K to 500 K find Cv if ΔU=5000J.
View Solution300 ml of a gas at 300 K is cooled to 276 K at constant pressure. The final volume is
View SolutionWhat happens to the pressure of a sample of helium gas if the temperature is increased from 200 K to 800 K, with no change in volume?
View SolutionIf the temperature of a black body is raised from 300K to 600K by what factor the rate of emission shall increase ?
View SolutionThe rate of a certain reaction increases by 2.3 times when the temperature is raised form 300K to 310K. If k is the rate constant at 300K, then the rate constant at 310K will be equal to
View SolutionThe variation of pressure with volume of the gas at different temperatures can be graphically represented as shown in figure. On the basis of this graph answer the following question.
(i) How will the volume of a gas change if its pressure is increased at constant temperature ?
(ii) At a constant pressure, how will the volume of a gas change if the temperature is increased from 200 K to 400 K ?
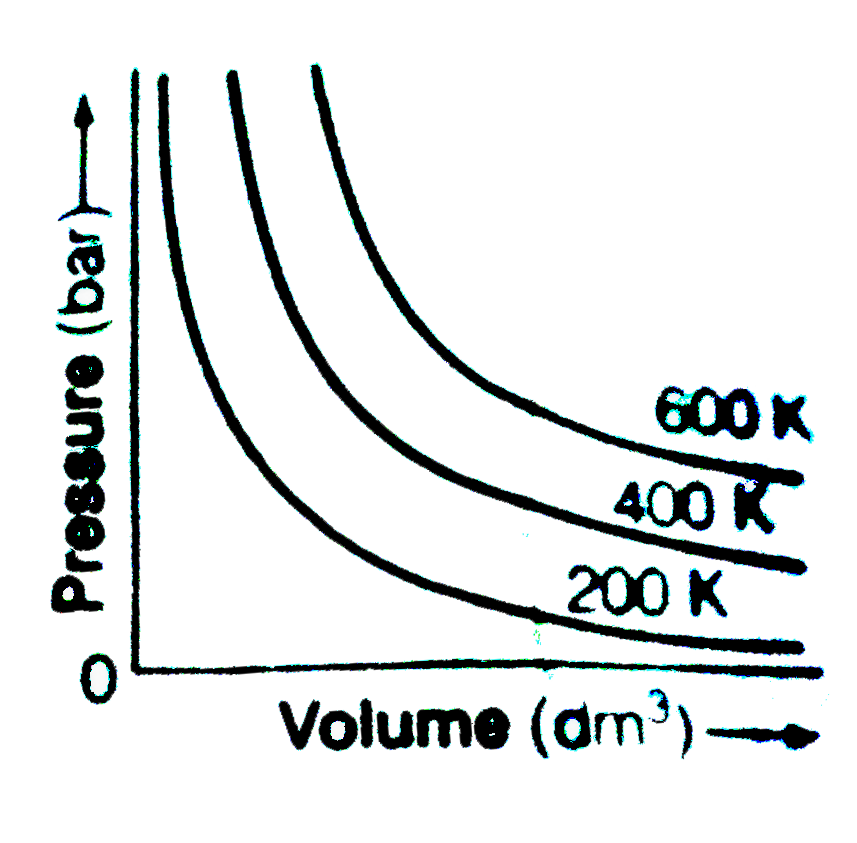 View Solution
View SolutionThe temperature of 5 moles of a gas at constant volume is changed from 100∘C to 120∘C. The change in internal energy is 80 J. The total heat capacity of the gas at constant volume will be in JK.
View SolutionWhen the pressure of 5 L of N2 is double and its temperature is raised from 300 K to 600 K, the final volume of the gas would be
View Solution

