The critical constants PC&TC for methane are 45 atm and 180 K. The correct statement is -
VC=2.4L
b=0.04L/mol
VC=0.8L
b=0.8L/mol
The correct Answer is:B
To solve the problem regarding the critical constants PC and TC for methane, we will follow these steps:
1. Identify the Given Values:
- Critical Pressure PC=45 atm
- Critical Temperature TC=180 K
2. Use the Critical Constants Formulas:
- The critical pressure is given by the formula:
PC=A27B2
- The critical temperature is given by:
TC=8A27RB
Here, A and B are constants specific to the substance, and R is the gas constant.
3. Set Up the Equations:
- From the first equation, we can express A:
A=27B2PC
- From the second equation, we can express A as well:
A=27RBTC8
4. Equate the Two Expressions for A:
27B2PC=27RBTC8
5. Simplify the Equation:
- Cancel 27B from both sides (assuming B≠0):
BPC=RTC8
6. Substitute the Known Values:
B⋅45=R⋅1808
7. Solve for B:
- Rearranging gives:
B=R⋅1808⋅45
- Simplifying further:
B=R⋅180360=R2
8. Determine the Value of R:
- The gas constant R can be taken as 0.0821L atm K−1mol−1.
- Thus:
B=0.08212=0.04105L/mol
9. Calculate the Critical Volume:
- The critical volume VC is given by:
VC=3B
- Therefore:
VC=3×0.04105=0.12315L
10. Conclusion:
- The correct statement is that the value of B is approximately 0.04L/mol.
- Critical Pressure PC=45 atm
- Critical Temperature TC=180 K
- The critical pressure is given by the formula:
PC=A27B2
- The critical temperature is given by:
TC=8A27RB
Here, A and B are constants specific to the substance, and R is the gas constant.
- From the first equation, we can express A:
A=27B2PC
- From the second equation, we can express A as well:
A=27RBTC8
27B2PC=27RBTC8
- Cancel 27B from both sides (assuming B≠0):
BPC=RTC8
B⋅45=R⋅1808
- Rearranging gives:
B=R⋅1808⋅45
- Simplifying further:
B=R⋅180360=R2
- The gas constant R can be taken as 0.0821L atm K−1mol−1.
- Thus:
B=0.08212=0.04105L/mol
- The critical volume VC is given by:
VC=3B
- Therefore:
VC=3×0.04105=0.12315L
- The correct statement is that the value of B is approximately 0.04L/mol.
Similar Questions
If a, b, c are in A.P. as well as in G.P. then correct statement is :
View SolutionFor a reaction A→P, the plots of [A] and [P] with time at temperatures T1 and T2 are given below.
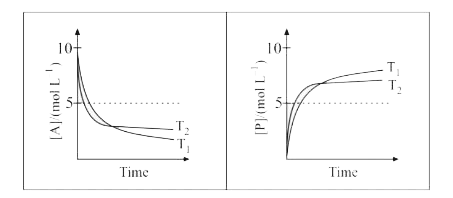
If T1>T2, the correct statements is (are):
(Assume ΔHΘ and ΔSΘ are independent of temperature and ratio of ln K at T1 to ln K at T2 is greater than T2T1. Here H,S,G and K are enthalpy, entropy, Gibbs energy and equilibrium constant, respectively).
View SolutionThe relationship between PC,VC and TC is
View SolutionFor A+B⇔C+D , the equilibrium constant is K1 and for C+D⇔A+B, the equilibrium constant is K2. The correct relation between K1 and K2 is
View SolutionThe temperature of 5 moles of a gas is decreased by 2K at constant pressure of 1 atm. Indicate the correct statement
View SolutionThe critical temperature (Tc) and pressure (Pc) of NO are 177K and 6.48MPa, respectively, and that of Cl4 are 550K and 4.56MPa, respectively. Which gas (a) has the smaller value for the van der Waals constant b, (b) has the smaller value for constant a, (c) has the larger critical volume, and (d) is most nearly ideal in behaviour at 300K and 1.013MPa.
View SolutionThe critical temperatures of carbon dioxide and methane are 31.1∘C and −81.9∘C, respectively. Which of them has stronger intermolecular forces and why?
View SolutionIf the critical constants for a hypothetical gas are Vc=150cm3mol−1.Pc=50atm and Tc=300K. then the radius of the molecule is: [Take R=112Ltratmmol−1K−1]
View SolutionTcandPc of a gas are 400 K and 41 atms respectively . The Vc is
View SolutionThe critical pressure PC and critical temperature TC for a gas obeying van der Waal's equation are 80 atm at 87∘C. molar mass of the gas is 130 g/mole. The compressibility factor for the above gas will be smaller than unity under the following condition:
View Solution
