If there are nine straight lines of which five are concurrent at a point and the other four are concurrent at another point and no two of these nine lines are parallel, then the number of points of intersection is equal to
20
22
36
38
The correct Answer is:B
Step by step video & image solution for If there are nine straight lines of which five are concurrent at a point and the other four are concurrent at another point and no two of these nine lines are parallel, then the number of points of intersection is equal to by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Prove that the line of centres of two intersecting circles subtends equal angles at the two points of intersection
View SolutionFind, which of the following sets are empty :
The set of points of intersection of two parallel lines.
View SolutionA line is parallel to YZ-plane, if all the points on the
line have equal
View SolutionFrom Fig., write concurrent lines and their points of concurrence.
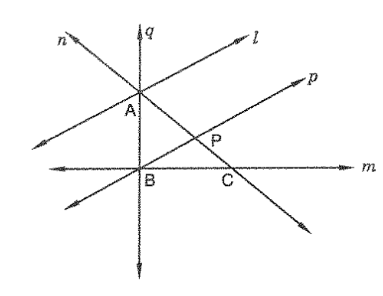
View SolutionIn a plane there are 37 straight lines, of which 13 passes through the point A and 11 passes through point B. Besides, no three lines passes through one point no line passes through both points A and B and no two are parallel, then find the number of points of intersection of the straight line.
View SolutionIn ;a plane, there are 5 straight lines which will pass through a given point, 6 others which all pass through another given point, and 7 others which all as through a third given point. Supposing no three lines intersect at any point and no two are parallel, find the number of triangles formed by the intersection of the straight line.
View SolutionIn a plane, there are 5 straight lines which will pass through a given point, 6 others which all pass through another given point, and 7 others which all as through a third given point. Supposing no three lines intersect at any point and no two are parallel, find the number of triangles formed by the intersection of the straight line.
View SolutionA line is parallel to XY- plane if all the points on the line have equal …………..
View SolutionAssertion: Number of triangles formed by n concurrent lines and a line which is parallel to one of the n concurrent lines is n−1C2. Reason: One and only one triangle is formed by three lines out of which 2 are concurrent and third line is not passing through the point of intersection of the concurrent lines and not parallel to any of the concurrent lines. (A) Both A and R are true and R is the correct explanation of A (B) Both A and R are true R is not the correct explanation of A (C) A is true but R is false. (D) A is false but R is true.
View SolutionSix straight lines are in a plane such that no two are parallel & no three are concurrent. The number of parts in which these lines divide the plane will be
View Solution
