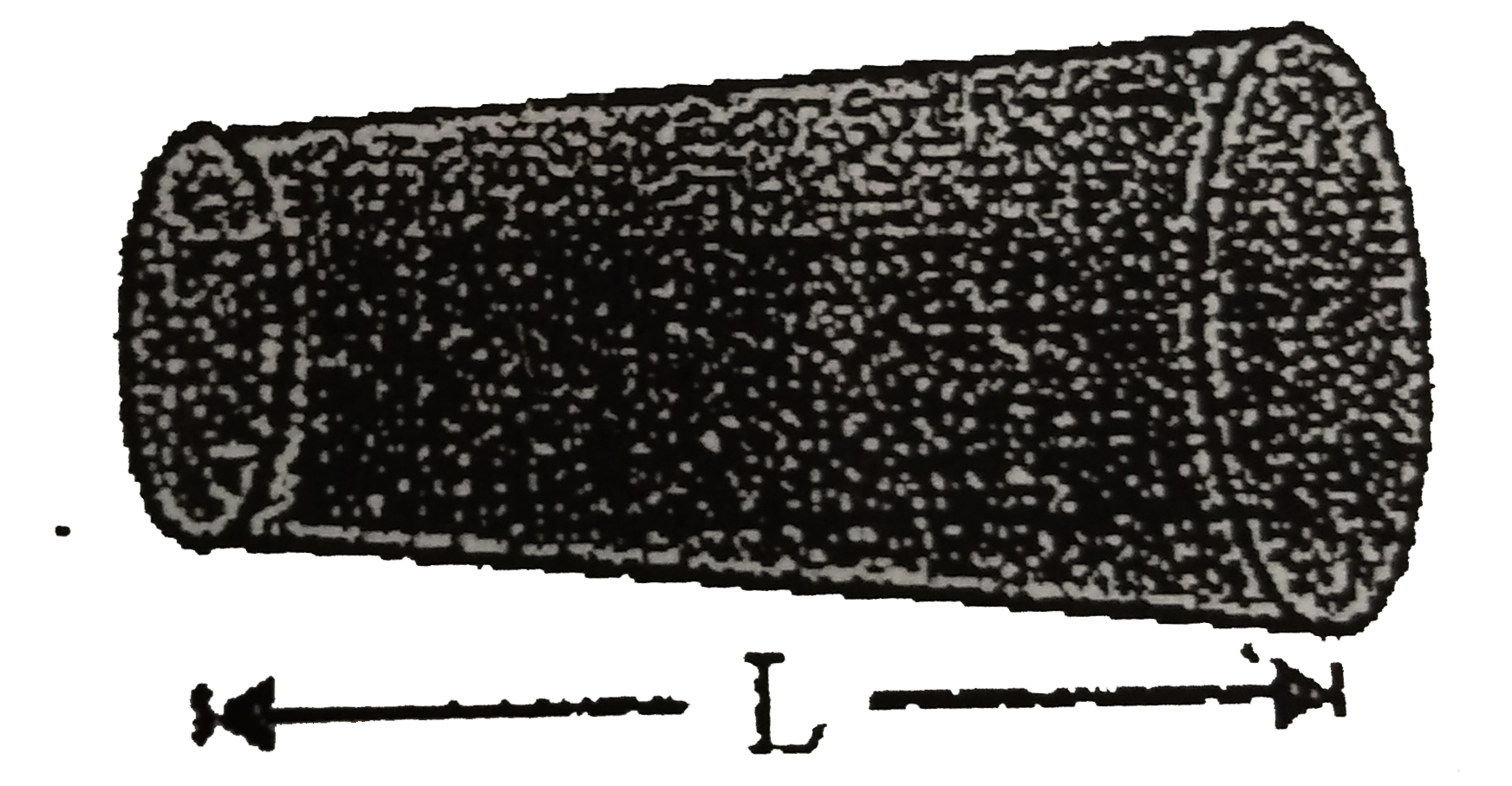
A thin bar of length L has a mass per unit length λ, that increases linerarly with distance from one end. If its total mass is M and its mass per unit length at the lighter end is λ0, then the distance of the centre of mass from the lighter end is
L3+λ0L28M
L3+λ0L24M
L2−λ0L24M
2L3−λ0L26M
The correct Answer is:D
To find the distance of the center of mass from the lighter end of a thin bar with a mass per unit length that increases linearly, we can follow these steps:
Step 1: Define the mass per unit length
The mass per unit length λ(x) of the bar increases linearly from λ0 at x=0 to some value at x=L. We can express this linear relationship as:
λ(x)=ax+λ0
where a is a constant that we need to determine.
Step 2: Determine the total mass
The total mass M of the bar can be calculated by integrating the mass per unit length over the length of the bar:
M=∫L0λ(x)dx=∫L0(ax+λ0)dx
Calculating this integral:
M=∫L0axdx+∫L0λ0dx=(ax22)L0+(λ0x)L0=aL22+λ0L
Step 3: Solve for a
Rearranging the equation for M:
M=aL22+λ0L
From this, we can solve for a:
a=2(M−λ0L)L2
Step 4: Find the center of mass
The x-coordinate of the center of mass xcm is given by:
xcm=1M∫L0x⋅λ(x)dx=1M∫L0x(ax+λ0)dx
Calculating this integral:
xcm=1M(∫L0ax2dx+∫L0λ0xdx)=1M((ax33)L0+(λ0x22)L0)
=1M(aL33+λ0L22)
Step 5: Substitute a into the center of mass equation
Substituting the expression for a:
xcm=1M(2(M−λ0L)L2⋅L33+λ0L22)
=1M(2L3(M−λ0L)+λ0L22)
Step 6: Simplify the expression
Now we simplify:
xcm=1M(2L3M−2λ0L23+λ0L22)
Finding a common denominator for the last two terms:
=1M(2L3M+(−4λ0L26+3λ0L26))
=1M(2L3M−λ0L26)
Final Result
Thus, the distance of the center of mass from the lighter end is:
xcm=2L3−λ0L26M
The mass per unit length λ(x) of the bar increases linearly from λ0 at x=0 to some value at x=L. We can express this linear relationship as:
λ(x)=ax+λ0
where a is a constant that we need to determine.
The total mass M of the bar can be calculated by integrating the mass per unit length over the length of the bar:
M=∫L0λ(x)dx=∫L0(ax+λ0)dx
Calculating this integral:
M=∫L0axdx+∫L0λ0dx=(ax22)L0+(λ0x)L0=aL22+λ0L
Rearranging the equation for M:
M=aL22+λ0L
From this, we can solve for a:
a=2(M−λ0L)L2
The x-coordinate of the center of mass xcm is given by:
xcm=1M∫L0x⋅λ(x)dx=1M∫L0x(ax+λ0)dx
Calculating this integral:
xcm=1M(∫L0ax2dx+∫L0λ0xdx)=1M((ax33)L0+(λ0x22)L0)
=1M(aL33+λ0L22)
Substituting the expression for a:
xcm=1M(2(M−λ0L)L2⋅L33+λ0L22)
=1M(2L3(M−λ0L)+λ0L22)
Now we simplify:
xcm=1M(2L3M−2λ0L23+λ0L22)
Finding a common denominator for the last two terms:
=1M(2L3M+(−4λ0L26+3λ0L26))
=1M(2L3M−λ0L26)
Thus, the distance of the center of mass from the lighter end is:
xcm=2L3−λ0L26M
Similar Questions
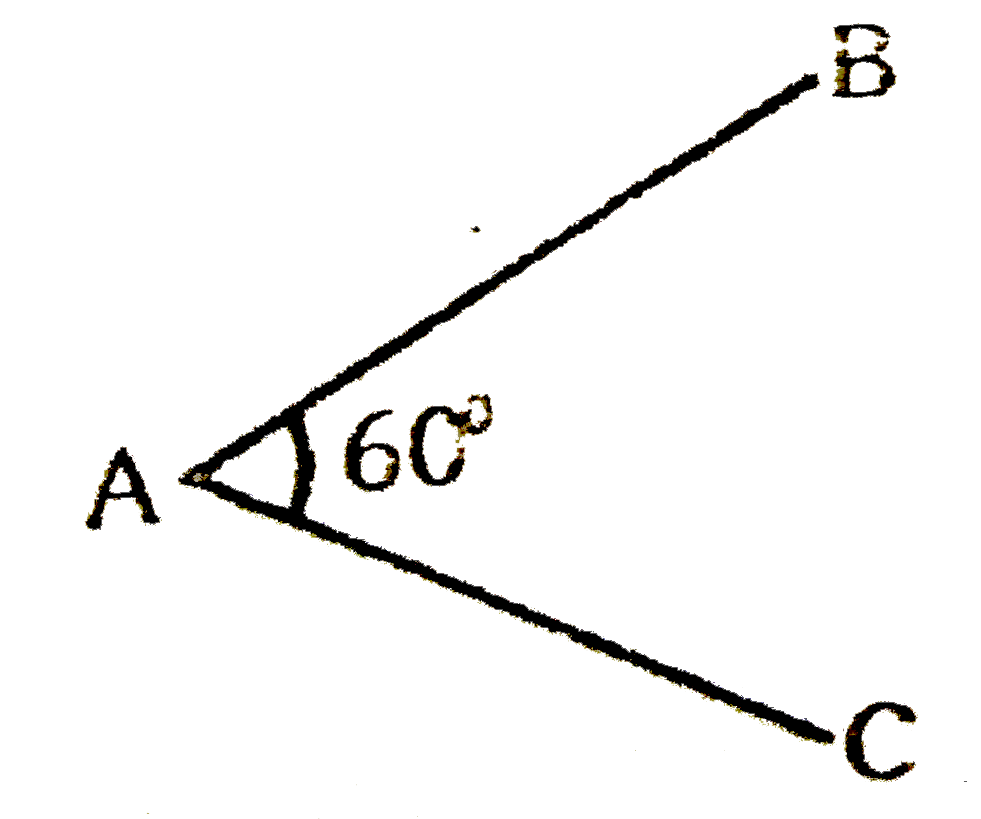
A uniform wire of length l is bent into the shape of 'V' as shown. The distance of its centre of mass from the vertex A is :-
View SolutionA particle placed
1.inside a uniform spherical shell of mass M, but not at the center
2.inside a uniform spherical shell of mass M, at the center
3.outside a uniform spherical shell of mass M, a distance r from the center
4.outside a uniform solid sphere of mass M, a distance 2r from the center
If F1,F2,F3 and F4 are gravitational forces acting on the particle in four cases.
View SolutionA rod of length L is of non uniform cross-section. Its mass per unit length varies linearly with distance from left end. Then its centre of mass from left end is at a distance. (Take linear density at left end=0).
View SolutionMass is non-uniformly distributed over the rod of length l, its linear mass density varies linearly with length as λ=kx2. The position of centre of mass (from lighter end) is given by-
View SolutionThe centre of mass of a non-uniform rod of length L whose mass per unit length λ is proportional to x2, where x is distance from one end
View SolutionA non–uniform thin rod of length L is placed along x-axis as such its one of ends at the origin. The linear mass density of rod is λ=λ0x. The distance of centre of mass of rod from the origin is :
View SolutionThere is a rod of length l , its density varies with length as distance of λ=ax , where x is distance from left end. Find center of mass from left end.
View SolutionA rod is of length 3 m and its mass acting per unit length is directly proportional to distance x from its one end. The centre of gravity of the rod from that end will be at
View SolutionThe linear density of a thin rod of length 1m lies as λ=(1+2x), where x is the distance from its one end. Find the distance of its center of mass from this end.
View SolutionFind coordinates of mass center of a non-uniform rod of length L whose linear mass density lambda varies as lambda=a+bx, where x is the distance from the lighter end.
View Solution
