A 70 kg man standing on ice throws a 3 kg body horizontally at 8m//s. The friction coefficient between the ice and his feet is 0.02. The distance, the man slip is
0.3m
2m
1m
∞
The correct Answer is:A
To solve the problem step by step, we will follow these steps:
Step 1: Identify the given data
- Mass of the man (M) = 70 kg
- Mass of the thrown body (m) = 3 kg
- Velocity of the thrown body (v) = 8 m/s
- Coefficient of friction (μ) = 0.02
Step 2: Calculate the normal force (N)
The normal force (N) acting on the man is equal to his weight, which can be calculated as:
N=M⋅g
where g is the acceleration due to gravity (approximately 9.8m/s2).
N=70kg⋅9.8m/s2=686N
Step 3: Calculate the frictional force (F_f)
The frictional force can be calculated using the formula:
Ff=μ⋅N
Ff=0.02⋅686N=13.72N
Step 4: Apply the conservation of momentum
Initially, both the man and the body are at rest, so the initial momentum is zero. After the man throws the body, the final momentum can be expressed as:
0=M⋅Vm+m⋅v
where Vm is the velocity of the man after throwing the body.
Rearranging gives:
Vm=−m⋅vM
Substituting the values:
Vm=−3kg⋅8m/s70kg=−2470≈−0.343m/s
Step 5: Calculate the acceleration (a) of the man
The acceleration of the man due to the frictional force can be calculated using Newton's second law:
a=FfM
a=13.72N70kg≈0.196m/s2
Step 6: Use the equations of motion to find the distance (s) slipped
Using the equation of motion:
V2=U2+2as
where:
- Final velocity V=0 (the man comes to rest)
- Initial velocity U=0.343m/s
- Acceleration a=−0.196m/s2 (deceleration)
Rearranging gives:
0=(0.343)2+2(−0.196)s
0.117649=0.392s
s=0.1176490.392≈0.3m
Final Answer
The distance the man slips is approximately 0.3 meters.
---
- Mass of the man (M) = 70 kg
- Mass of the thrown body (m) = 3 kg
- Velocity of the thrown body (v) = 8 m/s
- Coefficient of friction (μ) = 0.02
The normal force (N) acting on the man is equal to his weight, which can be calculated as:
N=M⋅g
where g is the acceleration due to gravity (approximately 9.8m/s2).
N=70kg⋅9.8m/s2=686N
The frictional force can be calculated using the formula:
Ff=μ⋅N
Ff=0.02⋅686N=13.72N
Initially, both the man and the body are at rest, so the initial momentum is zero. After the man throws the body, the final momentum can be expressed as:
0=M⋅Vm+m⋅v
where Vm is the velocity of the man after throwing the body.
Rearranging gives:
Vm=−m⋅vM
Substituting the values:
Vm=−3kg⋅8m/s70kg=−2470≈−0.343m/s
The acceleration of the man due to the frictional force can be calculated using Newton's second law:
a=FfM
a=13.72N70kg≈0.196m/s2
Using the equation of motion:
V2=U2+2as
where:
- Final velocity V=0 (the man comes to rest)
- Initial velocity U=0.343m/s
- Acceleration a=−0.196m/s2 (deceleration)
0=(0.343)2+2(−0.196)s
0.117649=0.392s
s=0.1176490.392≈0.3m
The distance the man slips is approximately 0.3 meters.
Similar Questions
A body of mass 2 kg is being dragged with uniform velocity of 2 m / s on a rough horizontal plane. The coefficient of friction between the body and the surface is 0.20. The amount of heat generated in 5 sec is
. (4.2joule/calandg=9.8m/s2)

View SolutionA constant horizontal force of 5N in magnitude is applied tangentially to the top most of a ring of mass 10 kg and radius 10 cm kept on a rough horizontal surface with coefficient of friction between surface and ring being 0.2. The friction force on the ring would be: (g=10m/s2)
View SolutionA man of mass 60 kg sitting on ice pushes a block of mass of 12kg on ice horizontally with a speed of 5 ms−1. The coefficient of friction between the man and ice and between block and ice is 0.2. If g =10 ms−2, the distances between man and the block, when they come to rest is
View Solution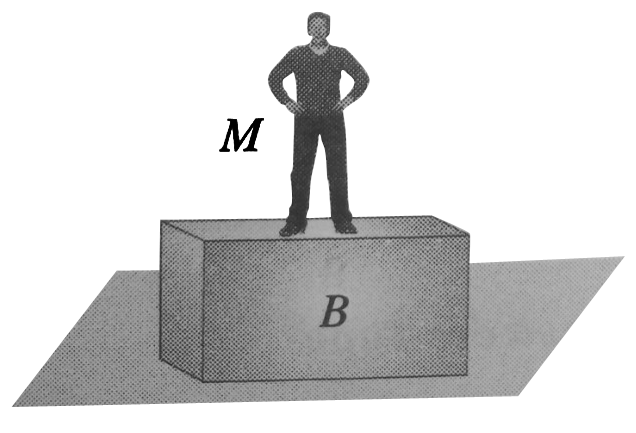
As shown in the figure, M is a man of mass 60 kg standing on a block of mass 40 kg kept on ground. The co-efficient of friction between the feet of the man and the block is 0.3 and that between B and the ground is 0.1. If the man accelerates with an acceleration 2ms2 in the forward direction, then,
View SolutionAs shown in figure, A is a man of mass 60kg standing on a block B of mass 40kg kept on ground. The coefficient of friction between the feet of the man and the block is 0.3 and that between B and the ground is 0.2 . If the person pulls the string with 125N force ,then

View SolutionA body of mass 5kg is under the action of 50N on the horizontal surface. If coefficient of friction in between the surfaces is one, the distance it travels in 3 s is
View SolutionA block A of mass 2kg rests on another block B of mass 8kg which rests on a horizontal floor. The coefficient of friction between A and B is 0.2 while that between B and floor is 0.5.When a horizontal floor F of 25N is applied on the block B the force of friction between A and B is
View SolutionA block of mass 10 kg slides down on an incline 5 m long and 3 m high. A man pushes up on the ice block parallel to the incline so that it slides down at constant speed. The coefficient of friction between the ice and the incline is 0.1. Find :
(a) the work done by the man on the block.
(b) the work done by gravity on the block.
(c) the work done by the surface on the block.
(d) the work done by the resultant forces on the block.
(e) the change in K.E. of the block.
View SolutionA body of mass 10kg is under the action of 138N on the horizontal surface. If coefficient of friction in between the surfaces is one, the distance it travels in 10s in metre is
View SolutionA 3 kg block is placed over a 10 kg block and both are palced on a smooth horizontal surface. The coefficient of friction between the block is 0.2 . If a horizontal force of 20 N is applied to 3 kg block, accelerations of the two block in ms−2 are (take, g=ms−2)
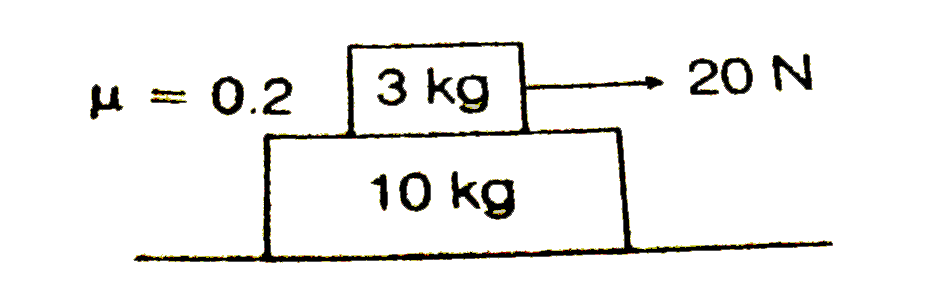
View Solution
