A man of mass 60 kg sitting on ice pushes a block of mass of 12kg on ice horizontally with a speed of 5 ms−1. The coefficient of friction between the man and ice and between block and ice is 0.2. If g =10 ms−2, the distances between man and the block, when they come to rest is
6 m
6.5 m
3 m
7 m
The correct Answer is:B
To solve the problem, we will follow these steps:
Step 1: Understand the problem
We have a man of mass 60 kg sitting on ice, and he pushes a block of mass 12 kg with an initial speed of 5 m/s. We need to find the distance between the man and the block when they both come to rest due to friction.
Step 2: Calculate the initial momentum
Using the conservation of linear momentum, we can set up the equation:
m1v1+m2v2=0
where:
- m1=60kg (mass of the man)
- v1 = final velocity of the man (to be determined)
- m2=12kg (mass of the block)
- v2=5m/s (initial speed of the block)
Setting up the equation:
60v1+12×(−5)=0
This simplifies to:
60v1−60=0
Thus,
v1=1m/s
Step 3: Calculate the distance traveled by the man (d1)
The man will come to rest due to friction. The frictional force can be calculated using:
f=μmg
where:
- μ=0.2
- g=10m/s2
So, the frictional force on the man is:
f1=0.2×60×10=120N
The deceleration a1 caused by this friction is:
a1=f1m1=12060=2m/s2
Using the equation of motion:
v2=u2+2as
where v=0 (final velocity), u=1m/s (initial velocity), and a=−2m/s2 (deceleration):
0=(1)2+2×(−2)×d1
This simplifies to:
1=4d1⟹d1=14m
Step 4: Calculate the distance traveled by the block (d2)
Similarly, for the block:
The frictional force on the block is:
f2=0.2×12×10=24N
The deceleration a2 for the block is:
a2=f2m2=2412=2m/s2
Using the same equation of motion:
0=(5)2+2×(−2)×d2
This simplifies to:
0=25−4d2⟹4d2=25⟹d2=254m
Step 5: Calculate the total distance between the man and the block
The total distance D between the man and the block when they come to rest is:
D=d1+d2=14+254=264=6.5m
Final Answer:
The distance between the man and the block when they come to rest is 6.5 meters.
---
We have a man of mass 60 kg sitting on ice, and he pushes a block of mass 12 kg with an initial speed of 5 m/s. We need to find the distance between the man and the block when they both come to rest due to friction.
Using the conservation of linear momentum, we can set up the equation:
m1v1+m2v2=0
where:
- m1=60kg (mass of the man)
- v1 = final velocity of the man (to be determined)
- m2=12kg (mass of the block)
- v2=5m/s (initial speed of the block)
60v1+12×(−5)=0
This simplifies to:
60v1−60=0
Thus,
v1=1m/s
The man will come to rest due to friction. The frictional force can be calculated using:
f=μmg
where:
- μ=0.2
- g=10m/s2
f1=0.2×60×10=120N
a1=f1m1=12060=2m/s2
v2=u2+2as
where v=0 (final velocity), u=1m/s (initial velocity), and a=−2m/s2 (deceleration):
0=(1)2+2×(−2)×d1
This simplifies to:
1=4d1⟹d1=14m
Similarly, for the block:
The frictional force on the block is:
f2=0.2×12×10=24N
a2=f2m2=2412=2m/s2
0=(5)2+2×(−2)×d2
This simplifies to:
0=25−4d2⟹4d2=25⟹d2=254m
The total distance D between the man and the block when they come to rest is:
D=d1+d2=14+254=264=6.5m
The distance between the man and the block when they come to rest is 6.5 meters.
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - AAKASH SERIESCHAPTER - LAWS OF MOTIONEXERCISE - EXERCISE - II
136 Videos
- GEOMETRICAL OPTICSBOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL-II PRACTICE SHEET (ADVANCED) INTEGER TYPE QUESTIONS)
10 Videos
- LOGIC GATESBOOK - AAKASH SERIESCHAPTER - LOGIC GATESEXERCISE - Exercise (Very Short Answer)
10 Videos
Similar Questions
A block of mass 2kg lying on ice when given a velocity of 6ms−1 is stopped by friction in 5s. The coefficient of friction between the block and ice is (g=10 ms−2)
View SolutionA man pulls a block of mass equal to himself with a light string .The coefficient of friction between the man and the floor is greater than that between the block and the floor
View SolutionA block of mass 50 kg slides over a horizontal distance of 1m. If the coefficient of friction between their surface is 0.2, then work done against friction is (take g=9.8m/s2) :
View SolutionA block of mass 8 kg is sliding on a surface inclined at an angle of 45∘ with the horizontal. Calculate the acceleration of the block. The coefficient of friction between the block and surface is 0.6. (Take g=10m/s2)
View SolutionA block of mass 1kg lies on a horizontal surface in a truck. The coefficeint of friction between the block and surface is 0.5. If the acceleration of the truck is 6ms−2 the acceleration of the block relative to ground is
View Solution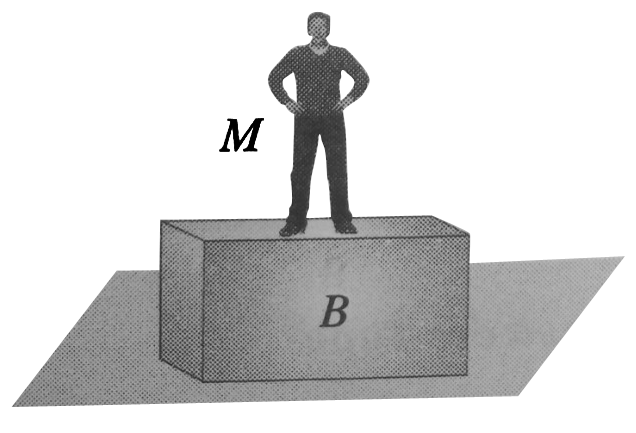
As shown in the figure, M is a man of mass 60 kg standing on a block of mass 40 kg kept on ground. The co-efficient of friction between the feet of the man and the block is 0.3 and that between B and the ground is 0.1. If the man accelerates with an acceleration 2ms2 in the forward direction, then,
View SolutionAs shown in figure, A is a man of mass 60kg standing on a block B of mass 40kg kept on ground. The coefficient of friction between the feet of the man and the block is 0.3 and that between B and the ground is 0.2 . If the person pulls the string with 125N force ,then

View SolutionA block of mass 8 kg is sliding on a surface inclined at an angle of 45∘ with the horizontal . Calculate the acceleration of the block . The coefficient of friction between the block and surface is 0⋅6 ( Take g = 10m/s2)
View SolutionA marble block of mass 2 kg lying on ice when given a velocity of 6m/s is stopped by friction in 10s. Then the coefficient of friction is
View SolutionA marble block of mass 2 kg lying on ice when given a velocity of 6m/s is stopped by friction in 10s. Then the coefficient of friction is
View Solution
