PQ and RS are two chords, of a circle. PQ =20 cm, RS =48 cm and PQ is parallel to RS. If the distance between PQ and RS is 34 cm, then what is the area (in cm2) of the circle?
PQ तथा RS एक वृत्त की 2 जीवाएं हैं। PQ=20 सेमी., RS =48 सेमी. तथा PQ,RS के समानान्तर है। यदि PQ तथा RS के मध्य दूरी 34 सेमी. है, तो वृत्त का क्षेत्रफल
(सेमी.2) क्या है?
A)729π
B)900π
C)676π
D)784π
To solve the problem, we will follow these steps:
Step 1: Understand the Geometry
We have a circle with two parallel chords PQ and RS. The lengths of the chords are given as PQ = 20 cm and RS = 48 cm. The distance between the two chords is 34 cm.
Step 2: Draw the Diagram
Draw a circle and label the center as O. Draw the chords PQ and RS parallel to each other. Let the perpendicular distances from the center O to the chords PQ and RS be OM and ON respectively.
Step 3: Divide the Chords
Since the perpendicular from the center to a chord bisects the chord, we can find the lengths of the segments:
- For chord PQ:
- PM = MQ = 20 cm / 2 = 10 cm
- For chord RS:
- RN = NS = 48 cm / 2 = 24 cm
Step 4: Use the Pythagorean Theorem
Now, we can apply the Pythagorean theorem in triangles OMP and ONR.
- For triangle OMP:
OP2=OM2+PM2
r2=OM2+102
- For triangle ONR:
OR2=ON2+RN2
r2=ON2+242
Step 5: Relate the Distances
The total distance between the two chords is given as:
OM+ON=34 cm
Let OM=x and ON=34−x.
Step 6: Set Up the Equations
From the triangles, we have:
1. r2=x2+102 (1)
2. r2=(34−x)2+242 (2)
Step 7: Equate the Two Expressions for r2
Set the two equations equal to each other:
x2+100=(34−x)2+576
Step 8: Expand and Simplify
Expanding the right side:
x2+100=1156−68x+x2+576
100=1156+576−68x
100=1732−68x
68x=1732−100
68x=1632
x=163268=24
Step 9: Find ON
Now, substitute x back to find ON:
ON=34−x=34−24=10
Step 10: Calculate the Radius
Now substitute x back into either equation to find r:
Using equation (1):
r2=242+102=576+100=676
r=√676=26 cm
Step 11: Calculate the Area of the Circle
Finally, the area A of the circle is given by:
A=πr2=π(262)=676π cm2
Final Answer
The area of the circle is 676π cm2.
---
We have a circle with two parallel chords PQ and RS. The lengths of the chords are given as PQ = 20 cm and RS = 48 cm. The distance between the two chords is 34 cm.
Draw a circle and label the center as O. Draw the chords PQ and RS parallel to each other. Let the perpendicular distances from the center O to the chords PQ and RS be OM and ON respectively.
Since the perpendicular from the center to a chord bisects the chord, we can find the lengths of the segments:
- For chord PQ:
- PM = MQ = 20 cm / 2 = 10 cm
- For chord RS:
- RN = NS = 48 cm / 2 = 24 cm
Now, we can apply the Pythagorean theorem in triangles OMP and ONR.
- For triangle OMP:
OP2=OM2+PM2
r2=OM2+102
- For triangle ONR:
OR2=ON2+RN2
r2=ON2+242
The total distance between the two chords is given as:
OM+ON=34 cm
Let OM=x and ON=34−x.
From the triangles, we have:
1. r2=x2+102 (1)
2. r2=(34−x)2+242 (2)
Set the two equations equal to each other:
x2+100=(34−x)2+576
Expanding the right side:
x2+100=1156−68x+x2+576
100=1156+576−68x
100=1732−68x
68x=1732−100
68x=1632
x=163268=24
Now, substitute x back to find ON:
ON=34−x=34−24=10
Now substitute x back into either equation to find r:
Using equation (1):
r2=242+102=576+100=676
r=√676=26 cm
Finally, the area A of the circle is given by:
A=πr2=π(262)=676π cm2
The area of the circle is 676π cm2.
Topper's Solved these Questions
Similar Questions
In the given figure, AP= 3 cm, AR= 6 cm and RS = 9 cm, then what is the value (in cm) of PQ?
View SolutionPQ and RS are two parallel chords of a circle whose centre is O and radius is 10 cm. If PQ =16cm and RS=12cm, find the distance between PQ and RS, If they lie on the same side of the Centre O.
View Solution
Knowledge Check
PQandRS are two parallel chords of a circle. If PQ=30 cm, RS=16 cm and distance between PQandRS is 23 cm, then find the radius of the circle.
AA)15BB)17CC)20DD)22PQRS is a rectangle, A, B, C and D are the mid points of sides PQ, QR, RS and PS respectively. If area of ΔPQR is 48 cm2, then what is the area (in cm2) of ΔBCD?
A24B6C16D12PQ and RS are two parallel chords of a circle with centre C such that PQ = 8 cm and RS = 16 cm. If the chords are on the same side of the centre and the distance between them is 4 cm, then the radius of the circle is :
A3√2 cmB3√5 cmC4√5 cmD5√5 cm
Triangle PQR is inscribed in the circle whose radius is 14 cm. If PQ is the diameter of the circle and PR = 10cm, then what is thearea of the triangle PQR?
त्रिभुज PQR वृत्त जिसकी भुजा 14 से.मी. है, में अंकित है। यदि PQ वृत्त का व्यास है तथा PR = 10 सेमी. हैं, तो त्रिभुज PQR का क्षेत्रफल क्या हैं?
View SolutionPQ and RS are two parallel chords of a circle whose centre is O and radius is 10cm. If PQ=16cm and RS=12cm, find the distance between PQ and RS, if they lie: on the same side of the centre O. on opposite side of the centre O.
View SolutionPQRSis a rectangle. Points A, B, C and D are the mid points of sides PQ,QR,RSandPSrespectively. If area of ΔPQR is 48cm2, then what is the area (in cm) of ΔBCD?
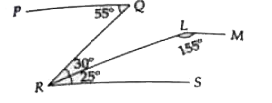
View SolutionIn the figure given below RS is parallel to PQ. what is angle between lines PQ and LM?
View SolutionPQRSis a rectangle in which side of PQ=24cm. and QR=16cm. T is a point on RS. What is the area (in cm.) of the triangle PTQ?
View Solution
