ABC is a triangle, PQ is line segment interseting AB in P and AC in Q and PQ||BC. The ratio of AP : BP = 3 : 5 and length of PQ is 18 cm. The length of BC is
28 cm
48 cm
84 cm
42 cm
Step by step video solution for ABC is a triangle, PQ is line segment interseting AB in P and AC in Q and PQ||BC. The ratio of AP : BP = 3 : 5 and length of PQ is 18 cm. The length of BC is by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
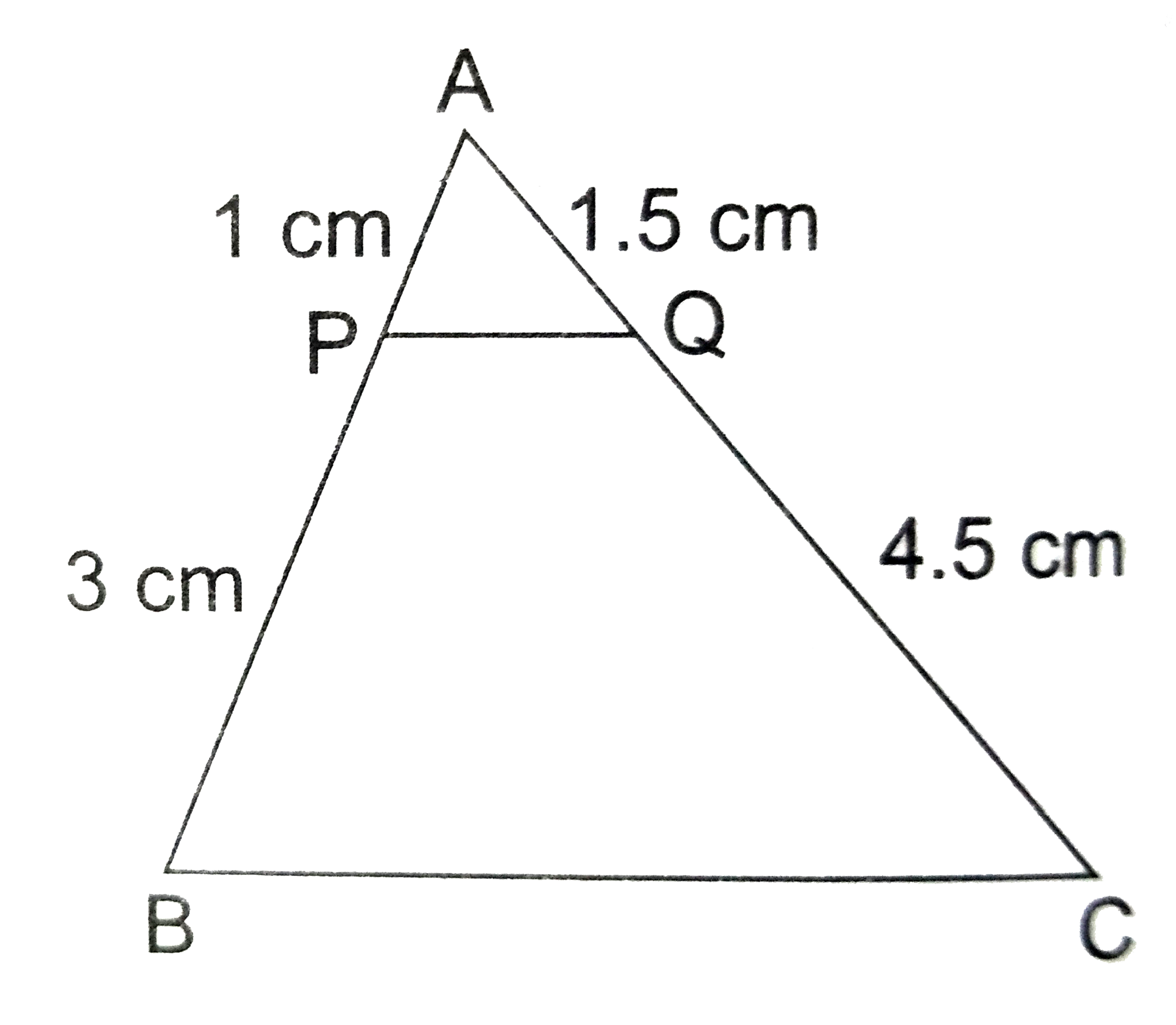
In the given figure, ABC is triangle and PQ is a stright line meeting AB in P and AC in Q. if AP=1cm,PB=3cm,AQ=1.5cm,QC=4.5cm prove that area of ΔAPQ is 116 of the area of ΔABC.
View SolutionIn ΔABC, PQ is a line segment which intersect AB on P and AC on Q so that PQ||BC and divides ΔABC in 2 equal parts of same area then find BPAB.
View Solution
Knowledge Check
△ABC is an isosceles triangle with AB=AC=15cm and altitude from A to BC is 12 cm. The length of sides BC is :
A9 cmB12 cmC18 cmD20 cmPoints P and Q lie on the sides AB and AC of triangle ABC respectivey such that line segment PQ is parallel to side BC. If the ratio of AP : PB is 1 : 4 and area of ΔAPQ is 4 square cm., what is the area of trapezium PQCB ?
A60 sq. cmB16 sq. cmC96 sq. cmD21 sq. cmIn ΔABC the straight line parallel to the side BCmeets ABandACat the points PandQ respectively. If AP=QC, the length of ABis 12 units and the length of AQis 2 units, then the length (in units) of CQ is
A4B6C8D10
Draw line segment PQ with the length 9cm.
View SolutionIn ABC , PQ is a line segment intersecting AB at P and AC at Q such that PQBC and PQ divides ABC into two parts equal in area. Find BPAB .
View SolutionB is a point on line segment AC such that AB: BC is 3 : 2. If AC = 40 cm, then length of BC is
View SolutionIn a △ABC P and Q are points on AB and AC respectively and PQ||BC. Prove that the median AD bisects PQ.
View SolutionInside a triangle ABC, a straight line parallel to BC intersects AB and AC at the point P and respectively. If AB=3PB, then PQ:BC is
View Solution
