ABCD is a trapezium with AD and BC parallel sides. E is a point on BC. The ratio of the area trapezium ABCD to that of triangle AED is-
ABCD एक AD और BC समांतर भुजाओं वाला समलंब चतुर्भुज है। E,BC पर एक बिंदु है। □ABCD और △AED के क्षेत्रफल का अनुपात क्या होगा?
¯¯¯¯¯¯AD¯¯¯¯¯¯BC
¯¯¯¯¯¯BE¯¯¯¯¯¯EC
¯¯¯¯¯¯AD+¯¯¯¯¯¯BE¯¯¯¯¯¯AD+¯¯¯¯¯¯CE
¯¯¯¯¯¯AD+¯¯¯¯¯¯BC¯¯¯¯¯¯AD
To find the ratio of the area of trapezium ABCD to that of triangle AED, we can follow these steps:
Step 1: Understand the Areas
1. Area of Trapezium ABCD: The area of a trapezium can be calculated using the formula:
Areatrapezium=12×(AD+BC)×h
where AD and BC are the lengths of the parallel sides, and h is the height of the trapezium.
2. Area of Triangle AED: The area of triangle AED can be calculated using the formula:
Area△AED=12×AD×h
where AD is the base of the triangle and h is the height from point E to line AD.
Step 2: Set Up the Ratio
Now, we need to set up the ratio of the area of trapezium ABCD to the area of triangle AED:
Ratio=AreatrapeziumArea△AED=12×(AD+BC)×h12×AD×h
Step 3: Simplify the Ratio
1. The 12 and h in both the numerator and denominator cancel out:
Ratio=(AD+BC)AD
Step 4: Final Expression
Thus, the ratio of the area of trapezium ABCD to that of triangle AED is:
Ratio=1+BCAD
Conclusion
The final answer is:
Ratio=AD+BCAD
1. Area of Trapezium ABCD: The area of a trapezium can be calculated using the formula:
Areatrapezium=12×(AD+BC)×h
where AD and BC are the lengths of the parallel sides, and h is the height of the trapezium.
Area△AED=12×AD×h
where AD is the base of the triangle and h is the height from point E to line AD.
Now, we need to set up the ratio of the area of trapezium ABCD to the area of triangle AED:
Ratio=AreatrapeziumArea△AED=12×(AD+BC)×h12×AD×h
1. The 12 and h in both the numerator and denominator cancel out:
Ratio=(AD+BC)AD
Thus, the ratio of the area of trapezium ABCD to that of triangle AED is:
Ratio=1+BCAD
The final answer is:
Ratio=AD+BCAD
Topper's Solved these Questions
Similar Questions
Knowledge Check
ABCD is a cyclic trapezium whose side AD and BC are parallel to each other. If ∠ABC=62∘ then the measure of the ∠BCD is :
A120∘B118∘C62∘D28∘ABCD is a cyclic trapezium whose sides AD and BC are parallel to each other. If ∠ABC=72∘, then the measure of the ∠BCD is
A162∘B18∘C108∘D72∘ABCD is a trapezium , in which AD∣∣BC, E and F are the mid-point of AB and CD respectively , then EF is :
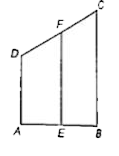 A(AD+BC)2B(AB+CD)2C(DF xx CF)/(AE xx BE)DAD+EF+BC2
A(AD+BC)2B(AB+CD)2C(DF xx CF)/(AE xx BE)DAD+EF+BC2
ABCD is a trapezium in which AB || DC, ABデ78 cm, CD = 52 cm. AD = 28 cm and BC =30cm . Find the area of the trapezium.
View SolutionABCD is a parallelogram, E and F are the mid-points of BC and CD. Find the ratio of area of parallelogram ABCD and ΔAEF
View SolutionABCD is a cyclic trapezium whose sides AD and BC are parallel to each other. If ∠ABC=75∘ then the measure of ∠BCD is:
View SolutionABCD is a trapezium in which AD∣∣BC and AB=DC=10m. then the distance of AD from BC is :
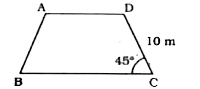
View SolutionABCD is a cyclic trapezium whose sides AD and BC are parallel to each other. If ∠ABC=72∘, then the measure of the ∠BCD is
View Solution
