Question

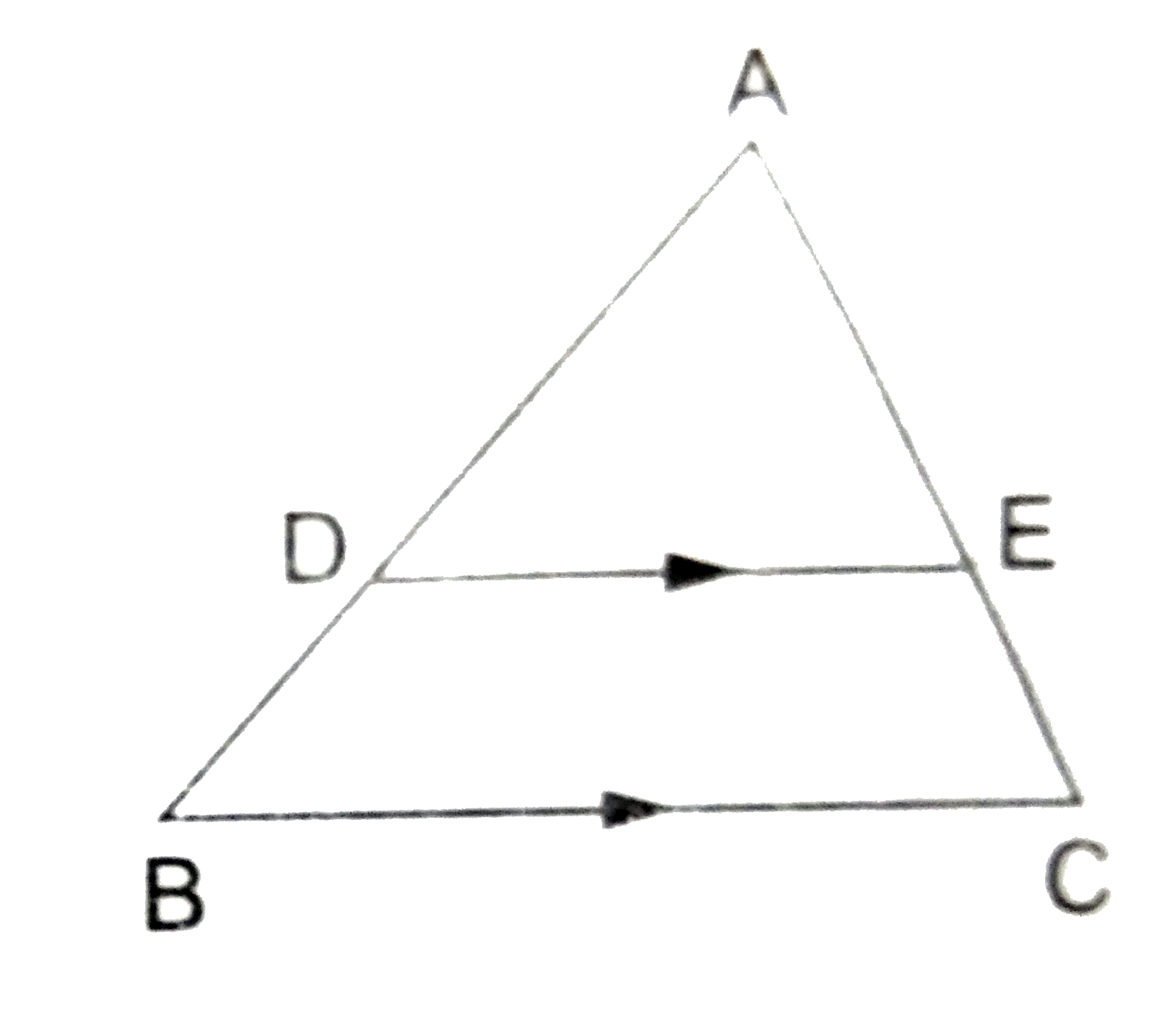
In triangle ABC a straight line parallel to BC intersects AB and AC at D and E respectively. If AB
= 2AD, then DE : BC is -

त्रिभुज ABC में BC के समांतर एक सरल रेखा AB और AC को क्रमशः D और E पर काटती है । यदि AB= 2AD, तो DE : BC कितना होगा ।

Options

A. `2:3`
B. `2:1`
C. `1:2`
D. `1:3`

Explanation

To solve the problem, we will use the properties of similar triangles and the concept of ratios.

1. Understanding the Triangle and the Line:
We have triangle ABC, and a line DE is drawn parallel to BC, intersecting AB at point D and AC at point E.

2. Given Information:
We know that \( AB = 2AD \). This implies that \( AD = \frac{1}{2} AB \).

3. Using Thales' Theorem:
According to Thales' theorem, if a line is drawn parallel to one side of a triangle, it divides the other two sides proportionally. Therefore, we can write:
\( \frac{AD}{AB} = \frac{DE}{BC} \)

4. Substituting the Known Values:
From the information given, we have:
\( AD = \frac{1}{2} AB \implies \frac{AD}{AB} = \frac{1/2 \cdot AB}{AB} = \frac{1}{2} \)

5. Setting Up the Ratio:
Now substituting this value back into the proportion from Thales' theorem:
\( \frac{1}{2} = \frac{DE}{BC} \)

6. Finding the Ratio DE : BC:
From the equation above, we can conclude:
\( DE : BC = 1 : 2 \)

Final Answer:
Thus, the ratio \( DE : BC \) is \( 1 : 2 \).

---