Question

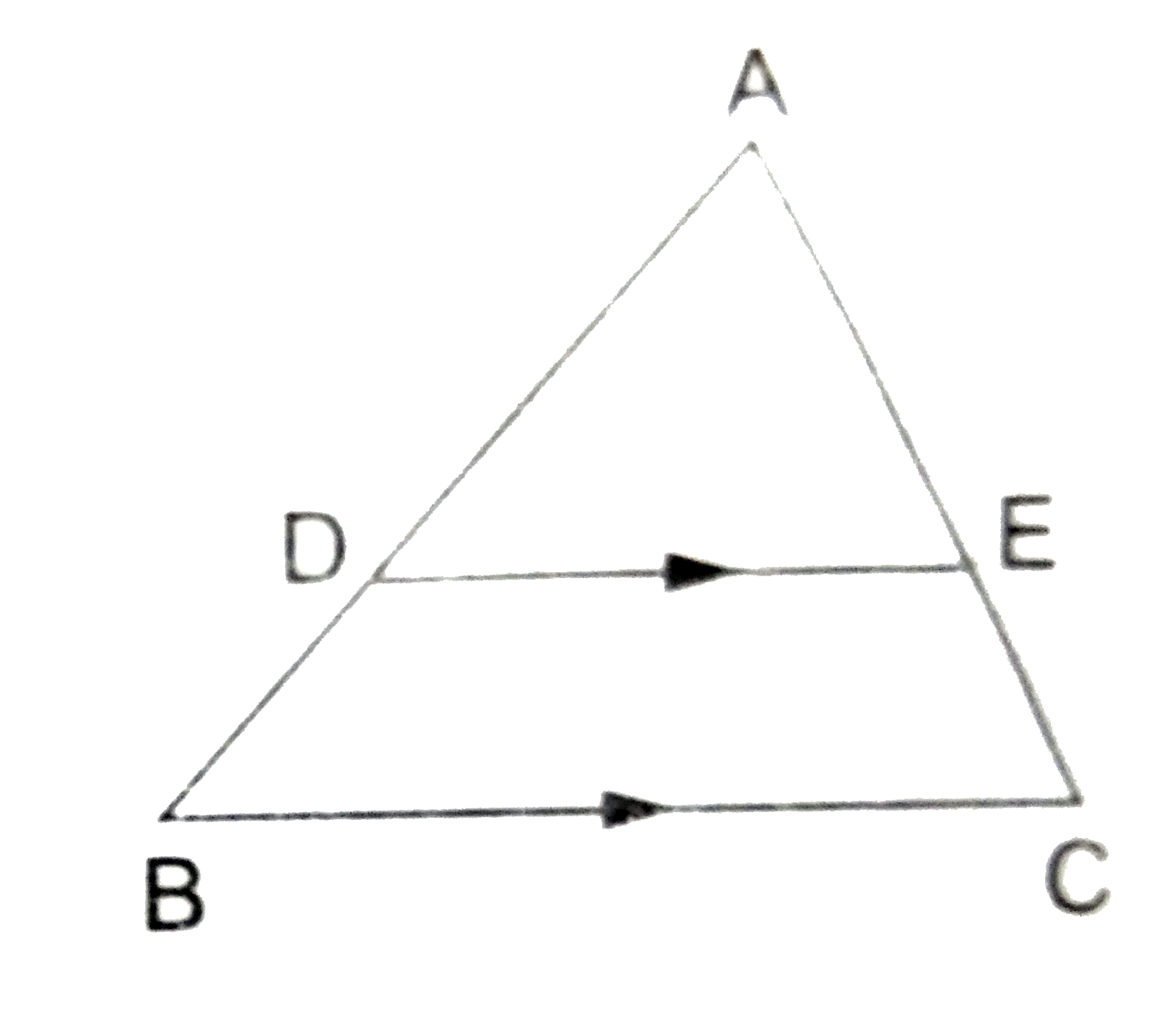
In a $△ABC$, DE is parallel to BC where D and E are the points on AB and AC,
respectively and AD = 4cm, DB = 8 cm, AE= 3 cm, Length of EC is:
triangle ABC में, DE, BC के समांतर है जहाँ D और E क्रमशः AB और AC पर बिंदु ह, और AD = 4 सेमी , DB= 8 सेमी , AE = 3 सेमी , तो EC की लम्बाई है ?

Options

A. 5 cm
B. 6 cm
C. 7 cm
D. 9 cm

Explanation

To find the length of EC in triangle ABC where DE is parallel to BC, we can use the properties of similar triangles. Here's the step-by-step solution:

Step 1: Understand the Given Information
We have triangle ABC with points D and E on sides AB and AC respectively. We know:
- AD = 4 cm
- DB = 8 cm
- AE = 3 cm
- We need to find the length of EC.

Step 2: Use the Property of Parallel Lines
Since DE is parallel to BC, triangles ADE and ABC are similar by the Basic Proportionality Theorem (also known as Thales' theorem). This means that the ratios of the lengths of corresponding sides are equal.

Step 3: Set Up the Ratio
From the similarity of triangles, we can set up the following ratio:
\( \frac{AD}{DB} = \frac{AE}{EC} \)
Substituting the known values:
\( \frac{4}{8} = \frac{3}{EC} \)

Step 4: Simplify the Ratio
The left side simplifies to:
\( \frac{1}{2} = \frac{3}{EC} \)

Step 5: Cross Multiply to Solve for EC
Cross-multiplying gives us:
\( 1 \cdot EC = 2 \cdot 3 \)
\( EC = 6 \text{ cm} \)

Conclusion
The length of EC is 6 cm.

---