In △ABC, XY || BC, AX : XB = 2 : 1 then trianl≥AXY : triangle ABC= …….Solution in Telugu
9:4
4:9
1:9
2:3
Step by step video & image solution for In triangle ABC, XY || BC, AX : XB = 2 : 1 then trianlge AXY : triangle ABC= ……. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
In △ABC, DE∣∣BC and AD=13BD.
If BC = 4.5cm, Find DE.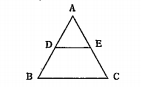
View SolutionWhich of the following are sets? Justify your answer.
The collection of all even integers.
View SolutionThe median of the data x/5, x, x/4, x/2 and x/8 is 8. Find the value of x.
View Solutionत्रिभुज ABC में Y क्रमशः AB और AC पर दो बिंदु है तथा XY।।BC और AX:XB=2:3, तो AY और YC का अनुपात क्या होगा?
View SolutionIn ΔABC,AB=BC then it is .......triangle .
View SolutionConstruct a triangle ABC with sides BC=3cm, AB=6cm and AC=4.5cm.Thenconstructa△whosessare4/3 of the corresponding sides of the trianlge ABC.
View SolutionΔABC లో XY || AC మరియు XY ల త్రిభుజాన్ని రెండు సమాన వైశాల్యాలు గల భాగాలుగా విభజించును. అయిన AX / XB నిష్పత్తిని కనుగొనండి.
View SolutionABC is a triangle. The straight line XY is drawn parallel to BC which meets AB and AC at points x and y respectively. If ar (DeltaAXY) : ar (squareBCCYX) = 4 : 5 Prove that AX: XB = 2: 1.
View SolutionIn ΔABC, XY || AC and XY divides the triangle into two parts of equal area. Find the ratio of AXXB.
View SolutionIn ΔABC, XY || AC and XY divides the triangle into two parts of equal area. Find the ratio of AXXB.
View SolutionIn ΔABC, XY∣∣BC, AYCY=12 and AX=4. Find BX.
View SolutionIn a ΔABC, XY∣∣BC and XY=12BC. If the area of ΔAXY=10cm2. Find the area of trapezium XYCB.
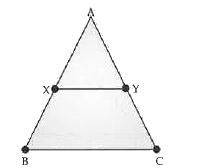
View SolutionIn ΔABC, XY∣∣BC and XY divides the triangle into two parts of equal area. Find BX:AB.
[Hint : ABC=2ΔAXY]
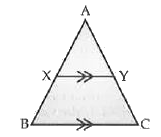
View SolutionA triangle ABC is placed so that the mid-points of the sides are on the x, y, z axes. Lengths of the intercept made by the plane containing the triangle on these axes are respectively α,β,γ. Coordinates of the centroid of the trianlge ABC are
View SolutionIn △ABC, points X and Y lie on AB and AC such that XY||BC, then which of the following statement is true? a)ABAC=AXAY b)AXXB=AYAC c)AXYC=AYXB d)ABYC=AVXB
View Solution
