In an isosceles triangle ABC,AB=AC,XY∣∣BC. If ∠A=30∘, then ∠BXY=?
75∘
30∘
150∘
105∘
The correct Answer is:D
To solve the problem, we need to find the measure of angle ∠BXY in the isosceles triangle ABC where AB=AC and XY is parallel to BC. Given that ∠A=30∘, we can follow these steps:
1. Identify the Angles in Triangle ABC:
- Since ABC is an isosceles triangle with AB=AC, the angles opposite these sides are equal. Therefore, we have:
∠B=∠C=θ
2. Use the Triangle Sum Property:
- The sum of the angles in any triangle is 180∘. Thus, we can write:
∠A+∠B+∠C=180∘
- Substituting the known values:
30∘+θ+θ=180∘
- This simplifies to:
30∘+2θ=180∘
3. Solve for θ:
- Rearranging the equation gives:
2θ=180∘−30∘
2θ=150∘
θ=150∘2=75∘
4. Determine the Angles ∠AXY and ∠BXY:
- Since XY is parallel to BC, the angles formed by the transversal AX are equal:
∠AXY=∠ABC=θ=75∘
- Similarly, since XY is parallel to BC:
∠CXY=∠ACB=θ=75∘
5. Use the Straight Angle Property:
- The angles along line AX must sum to 180∘:
∠AXY+∠BXY=180∘
- Substituting the known angle:
75∘+∠BXY=180∘
- Solving for ∠BXY:
∠BXY=180∘−75∘=105∘
Final Answer:
∠BXY=105∘
- Since ABC is an isosceles triangle with AB=AC, the angles opposite these sides are equal. Therefore, we have:
∠B=∠C=θ
- The sum of the angles in any triangle is 180∘. Thus, we can write:
∠A+∠B+∠C=180∘
- Substituting the known values:
30∘+θ+θ=180∘
- This simplifies to:
30∘+2θ=180∘
- Rearranging the equation gives:
2θ=180∘−30∘
2θ=150∘
θ=150∘2=75∘
- Since XY is parallel to BC, the angles formed by the transversal AX are equal:
∠AXY=∠ABC=θ=75∘
- Similarly, since XY is parallel to BC:
∠CXY=∠ACB=θ=75∘
- The angles along line AX must sum to 180∘:
∠AXY+∠BXY=180∘
- Substituting the known angle:
75∘+∠BXY=180∘
- Solving for ∠BXY:
∠BXY=180∘−75∘=105∘
∠BXY=105∘
Topper's Solved these Questions
- BOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-III)109videos
GEOMETRY
- BOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-IV)35videos
GEOMETRY
- BOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - TRY YOURSELF25videos
GEOMETRY
- BOOK - KIRAN PUBLICATIONCHAPTER - DISCOUNTEXERCISE - Test Yourself10videos
DISCOUNT
- BOOK - KIRAN PUBLICATIONCHAPTER - LCM AND HCF EXERCISE - Test Yourself18videos
LCM AND HCF
Similar Questions
Knowledge Check
- Question 1 - Select One
In an isosceles triangle ΔABC,AB=AC and ∠A=80∘. The bisector of ∠Band∠C meet at D. The ∠BDC is equal to the
A90∘B100∘C130∘D80∘ - Question 2 - Select One
A statements of Assertion (A) is followed by a statement of Reason (R ). Mark the correct choice as.
Assertion (A): ABC is an isoseles triangle right triangle , right angled at C. Then AB2=3AC2.
Reason (R ): In an isosceles triangle ABC if AC = BC and AB2=2AC2 then ∠C=90∘.ABoth A and R are true R is the correct explanation for A.BBoth A and R are true nad R is not correct explanation for A.CA is true but R is false.DA is false but R is true. - Question 3 - Select One
In an isosceles triangle ABC, AB=AC and ∠A=3∠B. Find ∠C.
A36∘B32∘C28∘D40∘
In ΔABC, if AB=AC and ∠A=50∘, then ∠C=
View SolutionIn a △ABC,AB = AC and AD⊥BC, then :
View SolutionIn an isosceles ΔABC, if AC=BC and AB2=2AC2 then ∠C=?
View Solution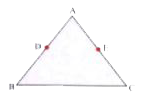
ABC is an isosceles triangle with AB = AC. If AD = AE then choose the correct alternatives.View SolutionIf the radius of the circum-circle of an isosceles triangle ABC is equal to AB(=AC), then angle A is
View Solution

