Consider curve y=f(x), then if PT = Length of tangent PN= Length of normal TM=Length of subtangent MN=Length of sub normal, then (a)TM.MN=f′(x) (b) PTPN=1f′(x) (c)CT.PN=constant (d) PT.TM=constant
To solve the problem, we need to analyze the relationships between the lengths of the tangent (PT), normal (PN), subtangent (TM), and subnormal (MN) for the curve y=f(x).
Step 1: Understand the Lengths
1. Length of Tangent (PT): The length of the tangent at a point (x,f(x)) on the curve is given by:
PT=|k|⋅√1+(dydx)2⋅1dydx
where k is a constant.
2. Length of Normal (PN): The length of the normal at the same point is given by:
PN=|k|⋅√1+(dydx)2
Step 2: Calculate the Ratio PTPN
Using the definitions above, we can find the ratio:
PTPN=|k|⋅√1+(dydx)2⋅1dydx|k|⋅√1+(dydx)2
This simplifies to:
PTPN=1dydx=1f′(x)
Conclusion for Option (b)
Thus, we have shown that:
PTPN=1f′(x)
This confirms that option (b) is correct.
Step 3: Analyze Other Options
Now we need to analyze the other options (a), (c), and (d).
Option (a): TM⋅MN=f′(x)
- Length of Subtangent (TM): The length of the subtangent is given by:
TM=f(x)f′(x)
- Length of Subnormal (MN): The length of the subnormal is given by:
MN=f′(x)⋅1f′(x)=1
Thus, TM⋅MN=f(x)f′(x)⋅1=f(x)f′(x), which is not equal to f′(x).
Option (c): CT⋅PN=constant
This option needs more context about what CT represents. Without additional information, we cannot confirm this option.
Option (d): PT⋅TM=constant
Using the definitions:
PT⋅TM=⎛⎜⎝|k|⋅√1+(dydx)2⋅1dydx⎞⎟⎠⋅(f(x)f′(x))
This expression does not simplify to a constant without specific conditions on f(x).
Final Summary
- The only confirmed option is (b): PTPN=1f′(x).
1. Length of Tangent (PT): The length of the tangent at a point (x,f(x)) on the curve is given by:
PT=|k|⋅√1+(dydx)2⋅1dydx
where k is a constant.
PN=|k|⋅√1+(dydx)2
Using the definitions above, we can find the ratio:
PTPN=|k|⋅√1+(dydx)2⋅1dydx|k|⋅√1+(dydx)2
This simplifies to:
PTPN=1dydx=1f′(x)
Thus, we have shown that:
PTPN=1f′(x)
This confirms that option (b) is correct.
Now we need to analyze the other options (a), (c), and (d).
- Length of Subtangent (TM): The length of the subtangent is given by:
TM=f(x)f′(x)
- Length of Subnormal (MN): The length of the subnormal is given by:
MN=f′(x)⋅1f′(x)=1
Thus, TM⋅MN=f(x)f′(x)⋅1=f(x)f′(x), which is not equal to f′(x).
This option needs more context about what CT represents. Without additional information, we cannot confirm this option.
Using the definitions:
PT⋅TM=⎛⎜⎝|k|⋅√1+(dydx)2⋅1dydx⎞⎟⎠⋅(f(x)f′(x))
This expression does not simplify to a constant without specific conditions on f(x).
- The only confirmed option is (b): PTPN=1f′(x).
Topper's Solved these Questions
Similar Questions
For the curve y=f(x) prove that (lenght of normal)^2/(lenght of tangent)^2 =length of sub-normal/length of sub-tangent
View SolutionIn the curve xm+n=am−ny2n , prove that the mth power of the sub-tangent varies as the nth power of the sub-normal.
View SolutionDetermine p such that the length of the sub-tangent and sub-normal is equal for the curve y=epx+px at the point (0,1).
View SolutionLet P (h,K) be any point on curve y=f(x). Let tangent drawn at point P meets x-axis at T & normal at point P meets x-axis at N (as shown in figure) and m =dydx](h,k)) = shope of tangent.
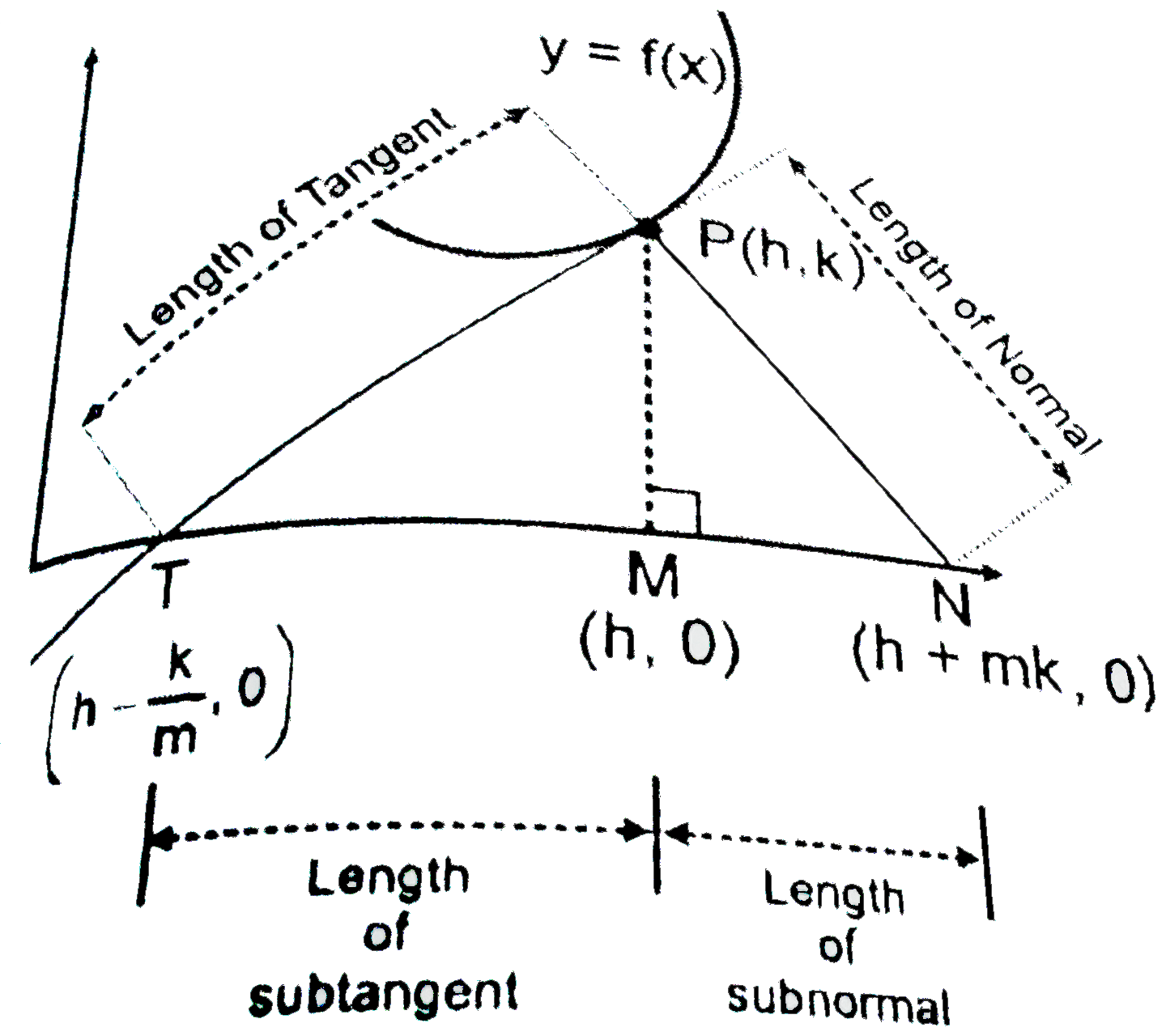
(i) Length of tangent =PT =|K|√1+1m2
(ii) Length of Normal =PN +|K|√1+m2
(iii) Length subtangent = Projection of segment PT on x-axis =TM=∣∣∣km∣∣∣
(iv) Length of subnormal =Projection of line segment PN on x-axis =MN =|Km|
Find the product of length of tangent and length of normal for the curve y=x3+3x2+4x−1 at point x=0
View SolutionThe length of the normal at t on the curve x=a(t+sint),y=a(1−cost), is
View SolutionIf f′(x)=√2x2−1andy=f(x2),thendydxatx=1 is (a)2 (b) 1 (c) −2 (d) none of these
View SolutionFor an A.P. show that tm+t2n+m=2tm+n
View SolutionIf x=f(t)cost−f′(t)sintandy=f(t)sint+f′(t)cost,then(dxdt)2+(dydt)2
View SolutionLet P (h,K) be any point on curve y=f(x). Let tangent drawn at point P meets x-axis at T & normal at point P meets x-axis at N (as shown in figure) and m =dydx](h,k)) = shope of tangent.

(i) Length of tangent =PT =|K|√1+1m2
(ii) Length of Normal =PN +|K|√1+m2
(iii) Length subtangent = Projection of segment PT on x-axis =TM=∣∣∣km∣∣∣
(iv) Length of subnormal =Projection of line segment PN on x-axis =MN =|Km|
Determine 'p' such that the length of the subtangent and subnormal is equal for the curve y=epx+px at the point (0,1)
View SolutionIf cos(x−y)cos(x+y)=mn, then write the value of tanx tany.
View Solution
