If A,B,C are three real numbers and p=[A+B+C] and q=[A]+[B]+[C]. (where [.] represents greatest integer function). Then maximum value of [p−q] is: 1 (b) 0 (c) 2 (d) 3
To solve the problem, we need to find the maximum value of (p−q), where p=(A+B+C) and q=(A)+(B)+(C). Here, (.) denotes the greatest integer function (also known as the floor function).
1. Understanding the Definitions:
- Let A=(A)+{A}, where {A} is the fractional part of A.
- Similarly, B=(B)+{B} and C=(C)+{C}.
2. Express p and q:
- We can express p as:
p=(A+B+C)=(((A)+{A})+((B)+{B})+((C)+{C}))=(((A)+(B)+(C))+({A}+{B}+{C}))
- Thus, we can write:
p=(q+({A}+{B}+{C}))
- Where q=(A)+(B)+(C).
3. Finding p−q:
- Now, we can express p−q:
p−q=(q+({A}+{B}+{C}))−q
- This simplifies to:
p−q=(q+({A}+{B}+{C}))−q
- Using the property of the greatest integer function, we have:
p−q=({A}+{B}+{C})
4. Analyzing the Fractional Parts:
- The fractional parts {A}, {B}, and {C} all lie in the range (0,1).
- Therefore, we can state:
0≤{A}+{B}+{C}<3
5. Finding the Maximum Value of (p−q):
- The maximum value of {A}+{B}+{C} can approach but never reach 3.
- The greatest integer function (x) gives the largest integer less than or equal to x.
- Hence, the maximum value of (p−q) can be:
({A}+{B}+{C})≤2
- Therefore, the maximum value of (p−q) is 2.
Conclusion:
The maximum value of (p−q) is 2, which corresponds to option (c).
- Let A=(A)+{A}, where {A} is the fractional part of A.
- Similarly, B=(B)+{B} and C=(C)+{C}.
- We can express p as:
p=(A+B+C)=(((A)+{A})+((B)+{B})+((C)+{C}))=(((A)+(B)+(C))+({A}+{B}+{C}))
- Thus, we can write:
p=(q+({A}+{B}+{C}))
- Where q=(A)+(B)+(C).
- Now, we can express p−q:
p−q=(q+({A}+{B}+{C}))−q
- This simplifies to:
p−q=(q+({A}+{B}+{C}))−q
- Using the property of the greatest integer function, we have:
p−q=({A}+{B}+{C})
- The fractional parts {A}, {B}, and {C} all lie in the range (0,1).
- Therefore, we can state:
0≤{A}+{B}+{C}<3
- The maximum value of {A}+{B}+{C} can approach but never reach 3.
- The greatest integer function (x) gives the largest integer less than or equal to x.
- Hence, the maximum value of (p−q) can be:
({A}+{B}+{C})≤2
- Therefore, the maximum value of (p−q) is 2.
The maximum value of (p−q) is 2, which corresponds to option (c).
Topper's Solved these Questions
Similar Questions
If a,b,c are three distinct real numbers in G.P. and a+b+c=xb, then prove that either x < -1 or x > 3
View SolutionIf a,b,c are three distinct real numbers in G.P. and a+b+c=xb, then prove that either x⟨−1orx⟩3.
View SolutionIf [.] denotes the greatest integer function, then (lim)x→0xa[bx] a. ba b. 0 c. ab d. does not exist
View SolutionIf αandβ are roots of the quadratic equation ax2+bx+c=0 and a+b+c>0,a−b+c>0&c<0,then[α]+[β] is equal to (where [.] denotes the greatest integer function) (a)10 (b) −3 (c) −1 (d) 0
View SolutionMatch the following lists (where [x] represents the greatest integer function) and then choose the correct code.
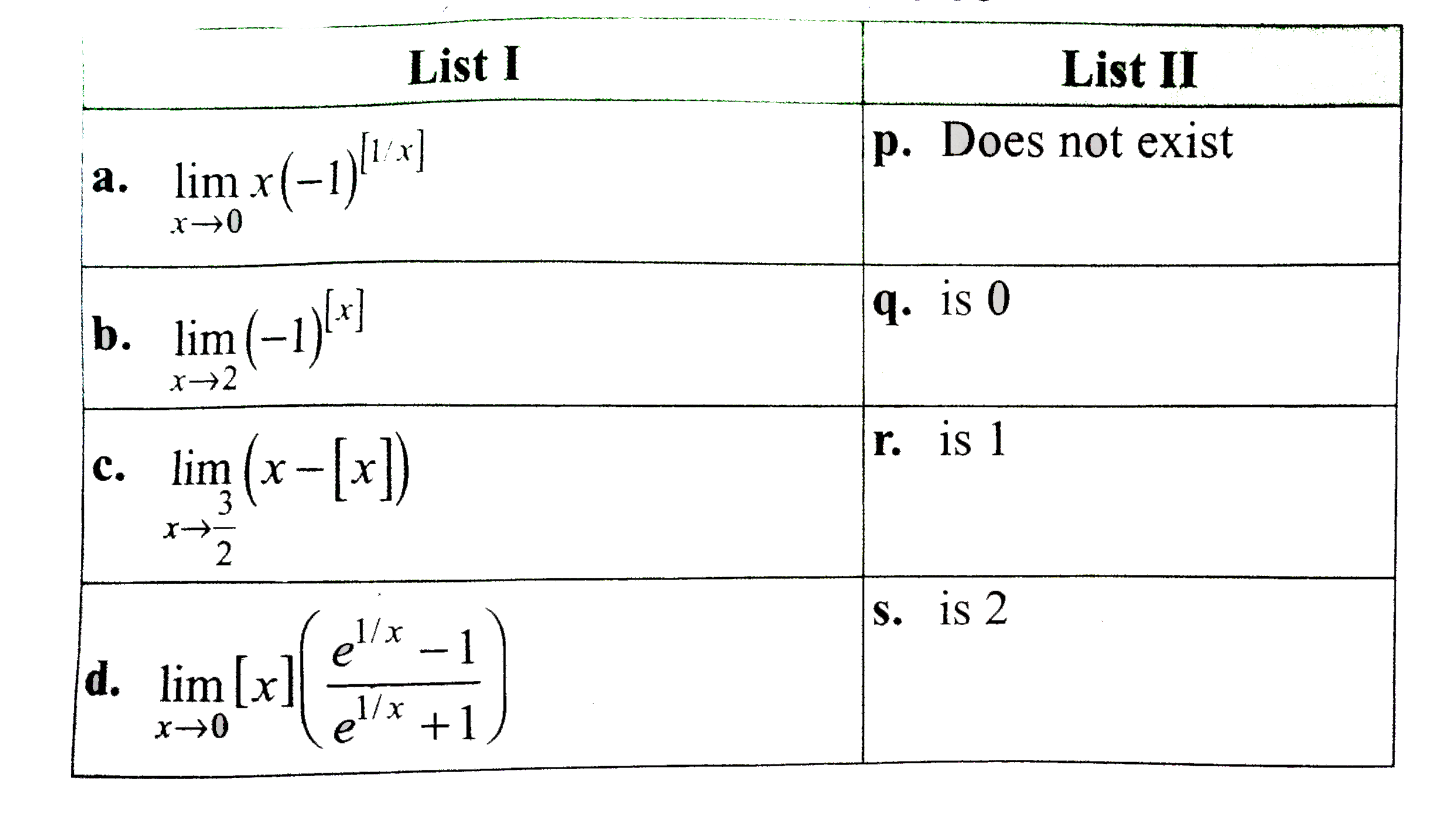
Codes :
a b c d(1)s r q p(2)q p s p(3)s r p q(4)p p q r
View SolutionLet a,bandc be real numbers such that a+2b+c=4 . Find the maximum value of (ab+bc+ca).
View SolutionIf a,b,c are three distinct positive real numbers in G.P., then prove that c2+2ab>3ac
View SolutionIf a,b,c are positive real numbers such that a + b +c=18, find the maximum value of a2b3c4
View SolutionThe function 'f' is defined by f(x)=xp(1−x)q for all x ∈R, where p, q are positive integers, has a maximum value, for x equal to : pqp+q (b) 1 (c) 0 (d) pp+q
View SolutionIf a, b, c are positive real numbers such that a+b+c=1, then the greatest value of (1-a)(1-b)(1-c), is
View Solution