If ABCD is a cyclic quadrilateral then find the value of sinA+sinB−sinC−sinD
To solve the problem, we need to find the value of sinA+sinB−sinC−sinD for a cyclic quadrilateral ABCD.
1. Understanding Cyclic Quadrilateral Properties:
A cyclic quadrilateral is one where all vertices lie on a circle. A key property of cyclic quadrilaterals is that the sum of opposite angles is 180 degrees. Therefore, we have:
A+C=180∘andB+D=180∘
2. Expressing Angles C and D:
From the properties above, we can express angles C and D in terms of angles A and B:
C=180∘−AandD=180∘−B
3. Substituting C and D into the Expression:
We substitute the expressions for C and D into our original expression:
sinA+sinB−sinC−sinD=sinA+sinB−sin(180∘−A)−sin(180∘−B)
4. Using the Sine Function Property:
We use the property of the sine function that states sin(180∘−θ)=sinθ. Therefore:
sin(180∘−A)=sinAandsin(180∘−B)=sinB
5. Simplifying the Expression:
Substituting these values back into our expression gives:
sinA+sinB−sinA−sinB
This simplifies to:
0
Final Answer:
Thus, the value of sinA+sinB−sinC−sinD is:
0
A cyclic quadrilateral is one where all vertices lie on a circle. A key property of cyclic quadrilaterals is that the sum of opposite angles is 180 degrees. Therefore, we have:
A+C=180∘andB+D=180∘
From the properties above, we can express angles C and D in terms of angles A and B:
C=180∘−AandD=180∘−B
We substitute the expressions for C and D into our original expression:
sinA+sinB−sinC−sinD=sinA+sinB−sin(180∘−A)−sin(180∘−B)
We use the property of the sine function that states sin(180∘−θ)=sinθ. Therefore:
sin(180∘−A)=sinAandsin(180∘−B)=sinB
Substituting these values back into our expression gives:
sinA+sinB−sinA−sinB
This simplifies to:
0
Thus, the value of sinA+sinB−sinC−sinD is:
0
Topper's Solved these Questions
Similar Questions
If ABC D is a cyclic quadrilateral, then find the value of sinA+sinB−sinC−sinD
If A+B+C=π2, then find the value of tanAtanB+tanBtanC+tanCtanA
View SolutionIf ABCD is a cyclic quadrilateral, then the value of cosA-cosB+cosC-cosD is equal to
View SolutionIn the adjoining figure, if ABCD is a cyclic quadrilateral, find the value of x.
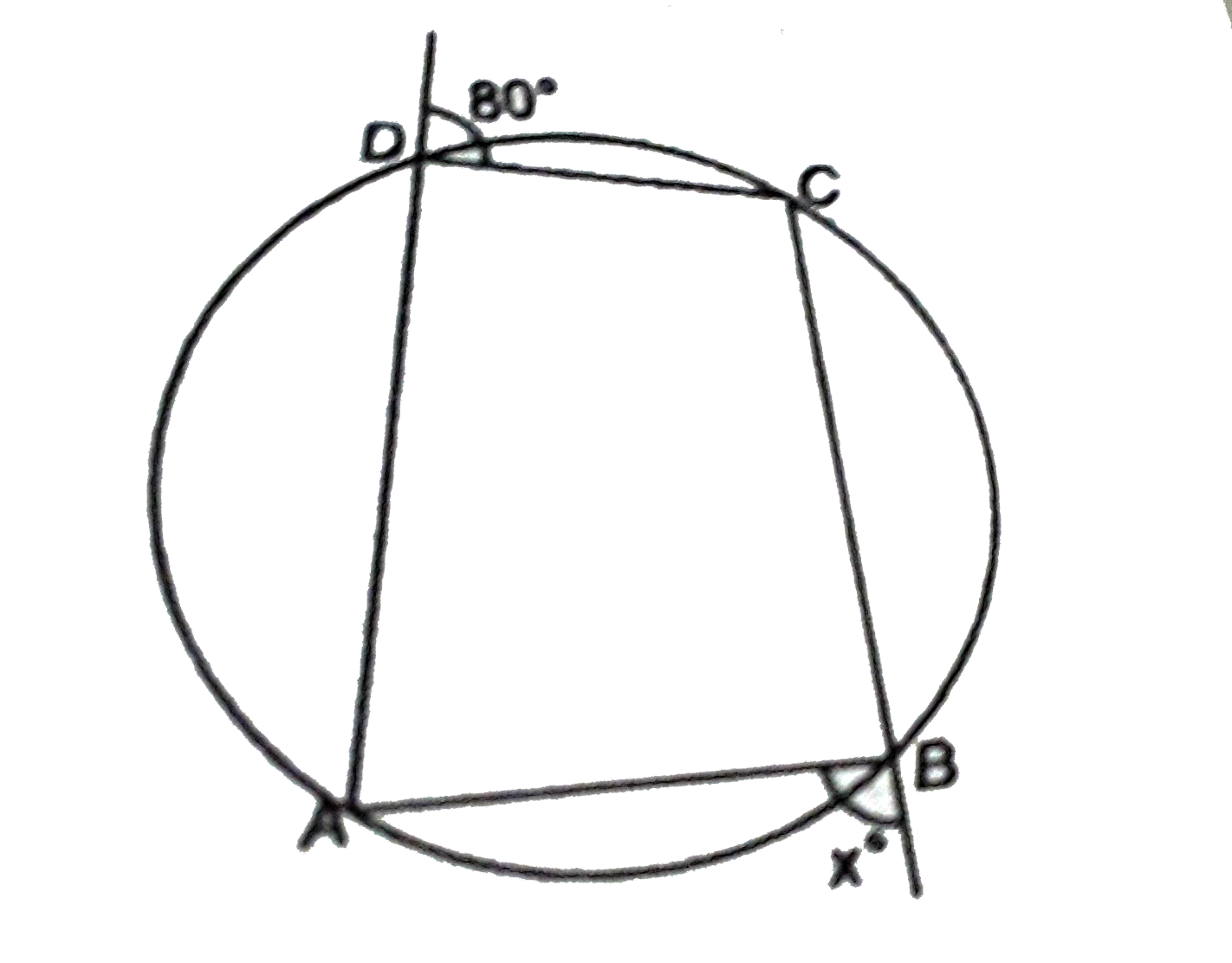
View SolutionLet ABCD be a cyclic quadrilateral then
Statement:1 sinA + sinB+ sinC + sinD=0
and Statement:2 cosA + cosB + cosC + cosD=0
View SolutionIn Figure, ABCD is a cyclic quadrilateral. Find the value of a and b.
View SolutionIn a cyclic quadrilateral ABCD, the value of 2+∑cosAcosB, is
View SolutionIf ABCD is a cyclic quadrilateral, then prove that AC.BD=AB.CD+BC.AD
View SolutionABCD is a cyclic quadrilateral (see Figure). Find the angles of the cyclic quadrilateral.
View SolutionIn the adjoining figure, ABCD is a cyclic quadrilateral whose side AB is the diameter of the circle . If ∠ADC=140∘ , then find the value of ∠BAC.

View SolutionIn the adjoining figure, ABCD is a cyclic quadrilateral and AB is the diameter of the circle. If ∠APC=120∘, then find the value of ∠CAB.
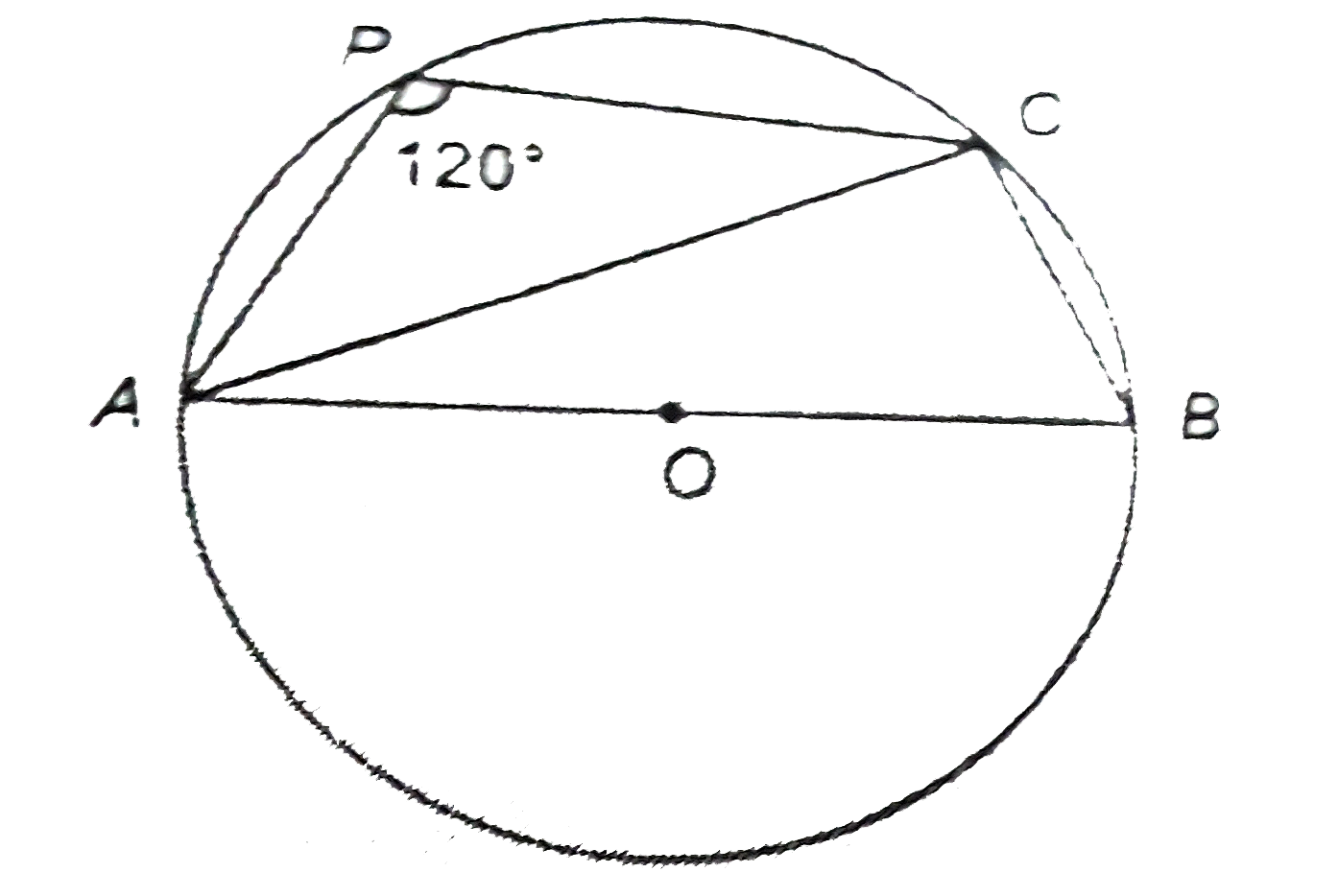
View Solution