A(-4,0) and B (-1,4) are two given points. Cand D are points which are symmetric to the given points A and B respectively with respect to y-axis. Calculate the perimeter of the trapezium ABDC.
To find the perimeter of trapezium ABDC formed by points A(-4,0), B(-1,4), C(4,0), and D(1,4), we will follow these steps:
Step 1: Identify the coordinates of points A, B, C, and D
- Given points:
- A = (-4, 0)
- B = (-1, 4)
- The points C and D are symmetric to A and B with respect to the y-axis:
- C = (4, 0) (symmetric to A)
- D = (1, 4) (symmetric to B)
Step 2: Calculate the lengths of the sides of the trapezium
1. Length of AB:
- Use the distance formula:
AB=√(x2−x1)2+(y2−y1)2
- Here, A(−4,0) and B(−1,4):
AB=√((−1)−(−4))2+(4−0)2=√(3)2+(4)2=√9+16=√25=5
2. Length of CD:
- Similarly, for points C(4, 0) and D(1, 4):
CD=√(1−4)2+(4−0)2=√(−3)2+(4)2=√9+16=√25=5
3. Length of AD:
- For points A(-4, 0) and D(1, 4):
AD=√(1−(−4))2+(4−0)2=√(5)2+(4)2=√25+16=√41
4. Length of BC:
- For points B(-1, 4) and C(4, 0):
BC=√(4−(−1))2+(0−4)2=√(5)2+(−4)2=√25+16=√41
Step 3: Calculate the perimeter of trapezium ABDC
- The perimeter P of trapezium ABDC is given by:
P=AB+BC+CD+AD
- Substituting the lengths:
P=5+√41+5+√41=10+2√41
Final Answer:
The perimeter of trapezium ABDC is 10+2√41.
---
- Given points:
- A = (-4, 0)
- B = (-1, 4)
- The points C and D are symmetric to A and B with respect to the y-axis:
- C = (4, 0) (symmetric to A)
- D = (1, 4) (symmetric to B)
1. Length of AB:
- Use the distance formula:
AB=√(x2−x1)2+(y2−y1)2
- Here, A(−4,0) and B(−1,4):
AB=√((−1)−(−4))2+(4−0)2=√(3)2+(4)2=√9+16=√25=5
- Similarly, for points C(4, 0) and D(1, 4):
CD=√(1−4)2+(4−0)2=√(−3)2+(4)2=√9+16=√25=5
- For points A(-4, 0) and D(1, 4):
AD=√(1−(−4))2+(4−0)2=√(5)2+(4)2=√25+16=√41
- For points B(-1, 4) and C(4, 0):
BC=√(4−(−1))2+(0−4)2=√(5)2+(−4)2=√25+16=√41
- The perimeter P of trapezium ABDC is given by:
P=AB+BC+CD+AD
- Substituting the lengths:
P=5+√41+5+√41=10+2√41
The perimeter of trapezium ABDC is 10+2√41.
Similar Questions
Let A≡(−4,0),B≡(−1,4).CandD are points which are symmetric to points AandB respectively with respect to y-axis, then area of the quadrilateral ABCD is (A) 8 sq units (B) 12 sq. units (C) 20 sq. units (D) none of these
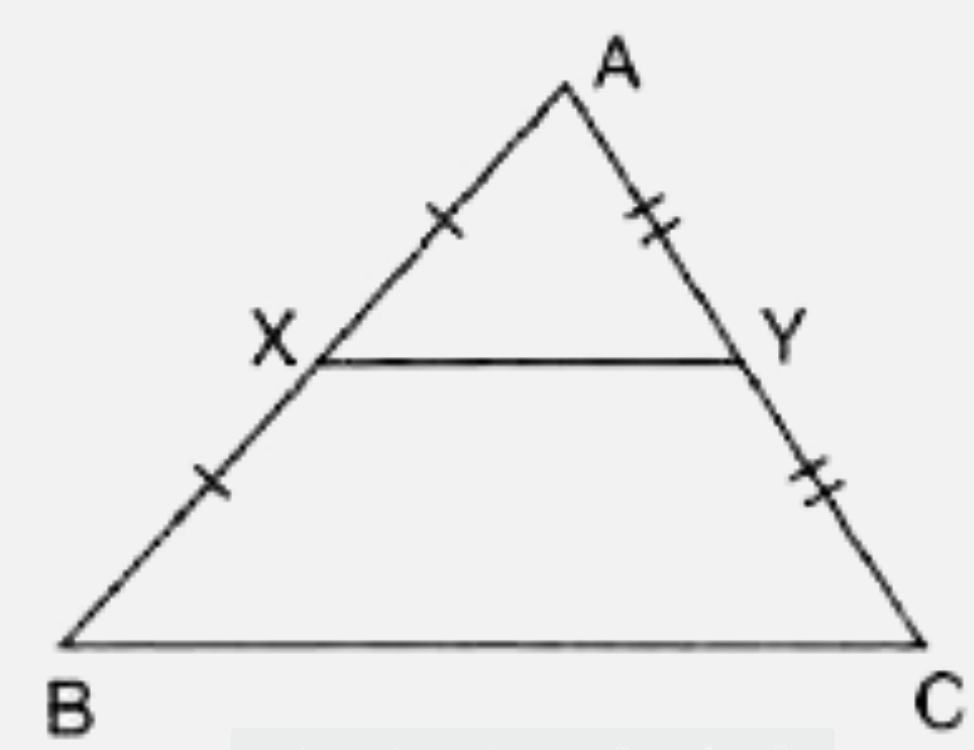
View SolutionIn the figure, given below X and Y are the mid-points of AB and AC respectively. Given that BC = 6 cm, AB = 5-4 cm and AC = 5-0 cm, calculate the perimeter of trapezium YXBC.
View SolutionIf the point A is symmetric to the point B(4,−1) with respect to the bisector of the first quadrant then AB is
View SolutionA(2, 0) and B(4, 0) are two given points. A point P moves so that PA2+PB2=10. Find the locus of P.
View SolutionIf the point A is symmetric to the point B(4,−1) with respect to the bisector of the first quadrant, then the length of AB is:
View SolutionFind the point on y-axis which is equidistant from the points A(-4,3)and B(5,2)
View SolutionThe inverse point of (1, -1) with respect to x2+y2=4, is
View SolutionPoint charges +4q,−q and +4q are kept on the x-axis at points x=0,x=a and x=2a respectively, then
View SolutionThe line xa+yb=1 meets the axis of and y at A and B respectively and C is middle point of AB then
View SolutionIn the given circuits calculate the resstance between points A and B.

View Solution
