If a quadrilateral can be insribed in one circle and circumsceribed about another circle, prove that its area is √abcd and the radius of the latter circle is √abcda+b+c+d
Step by step video & image solution for If a quadrilateral can be insribed in one circle and circumsceribed about another circle, prove that its area is sqrt(abcd) and the radius of the latter circle is (sqrt(abcd))/(a+b+c+d) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
The area of a circle is 616 cm2. Find the radius of the circle.
View SolutionThe area of a circle is 154 cm2. Find the radius of the circle.
View SolutionIf the radius of a circle is 3 times that of another circle, find the ratio of their areas.
View Solutionin the adjoining figure ABCD is a square of side 14 cm. Find the radius of the circle
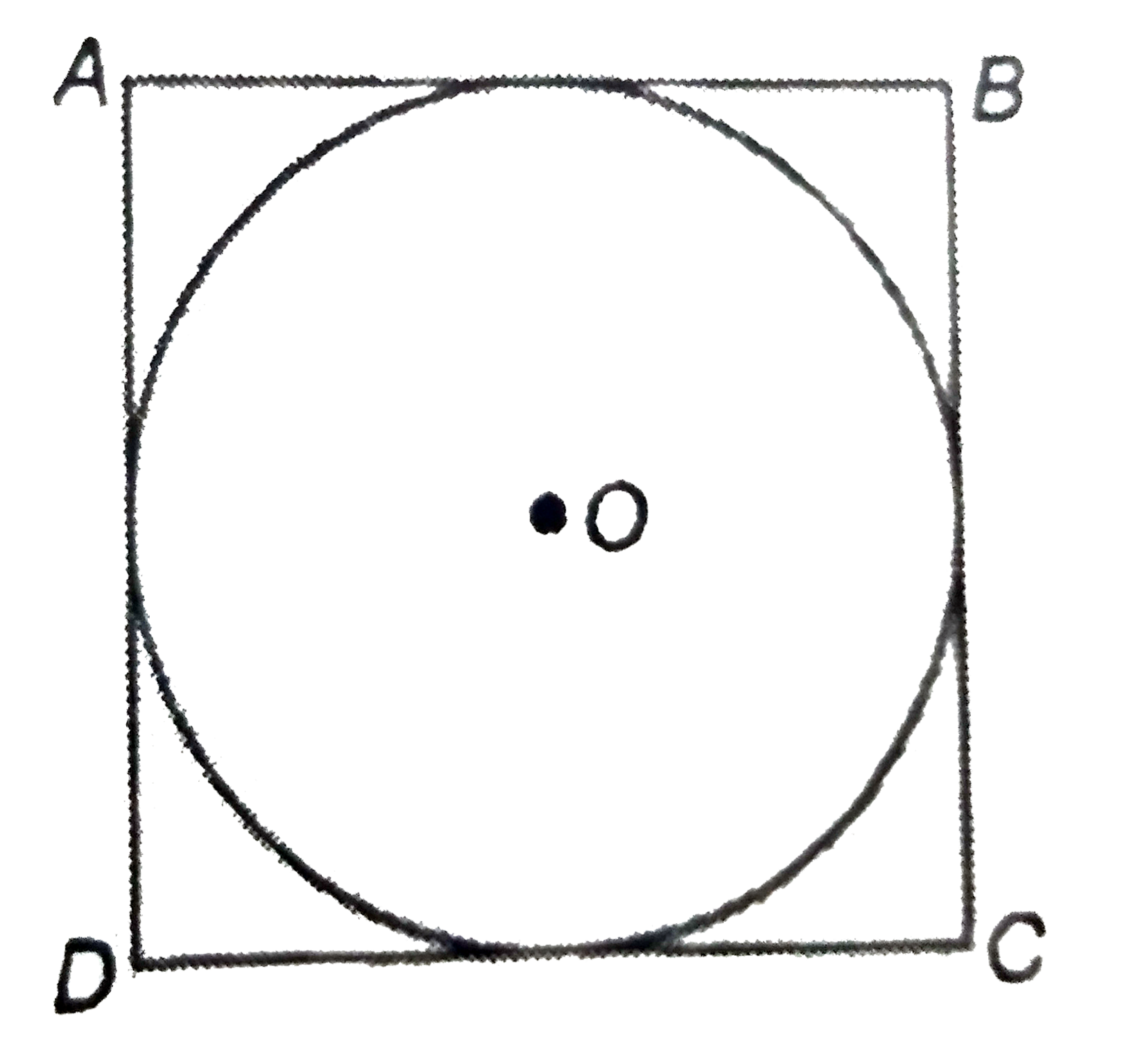
View SolutionA quadrilateral ABCD is drawn to circumscribe a circle. Prove that AB+CD=AD+BC
View SolutionA quadrilateral ABCD is drawn to circumscribe a circle. Prove that AB+CD=AD+BC
View SolutionA quadrilateral ABCD is drawn to circumscribe a circle. Prove that AB+CD=AD+BC
View SolutionIf bisectors of opposite angles of a cyclic quadrilateral ABCD intersect the circle, circumscribing it at the points P and Q, prove that PQ is a diameter of the circle.
View SolutionThe area of a square is equal to the area of a circle. The ratio between the side of the square and the radius of the circle is (a) √π:1 (b) 1:√π (c) 1:π (d) π:1
View SolutionThe centres of the circles are (a, c) and (b, c) and their radical axis is y-axis. The radius of one of the circles is r. The radius of the other circle is
View Solution
