. The velocity of a boat relative to water is represented by and that of water relative to the earth by . What is the velocity of the boat relative to the earth, if represent velocities of 1 km/hour east and north respectively .
To find the velocity of the boat relative to the earth, we can use the concept of relative velocity. The velocity of the boat relative to the water and the velocity of the water relative to the earth are given. We can express this mathematically and solve for the velocity of the boat relative to the earth.
1. Identify the Given Velocities:
- Velocity of the boat relative to water: →Vbw=3^i+4^j (in km/h)
- Velocity of water relative to earth: →Vwe=^i−3^j (in km/h)
2. Use the Relative Velocity Formula:
The relationship between the velocities can be expressed as:
→Vbw=→Vb−→Vw
where →Vb is the velocity of the boat relative to the earth and →Vw is the velocity of the water relative to the earth.
3. Rearranging the Equation:
Rearranging the equation gives:
→Vb=→Vbw+→Vw
4. Substituting the Known Values:
Substitute →Vbw and →Vwe:
→Vb=(3^i+4^j)+(^i−3^j)
5. Combine the Vectors:
Now, combine the components:
→Vb=(3^i+^i)+(4^j−3^j)=4^i+1^j
6. Final Result:
Therefore, the velocity of the boat relative to the earth is:
→Vb=4^i+1^j km/h
- Velocity of the boat relative to water: →Vbw=3^i+4^j (in km/h)
- Velocity of water relative to earth: →Vwe=^i−3^j (in km/h)
The relationship between the velocities can be expressed as:
→Vbw=→Vb−→Vw
where →Vb is the velocity of the boat relative to the earth and →Vw is the velocity of the water relative to the earth.
Rearranging the equation gives:
→Vb=→Vbw+→Vw
Substitute →Vbw and →Vwe:
→Vb=(3^i+4^j)+(^i−3^j)
Now, combine the components:
→Vb=(3^i+^i)+(4^j−3^j)=4^i+1^j
Therefore, the velocity of the boat relative to the earth is:
→Vb=4^i+1^j km/h
Similar Questions
(a) What physical quantity is measured in bar ?
(b) How is the unit bar related to the S.I. unit pascal ?View SolutionAn aeroplane is flying with a velocity 800kmph relative to air wind is belong from west to east with a velocity 15ms−1. Find velocity of aeroplane relative to the earth to fly south ward
View Solution
Knowledge Check
- Question 1 - Select One
A motor boat is racing towards North at 25km/h and the water current in that region is 10km/h in the direction of 60∘ East of South. Find the resultant velocity of the boat.
A11 km h−1B22 km h−1C33 km h−1D44 km h−1 - Question 2 - Select One
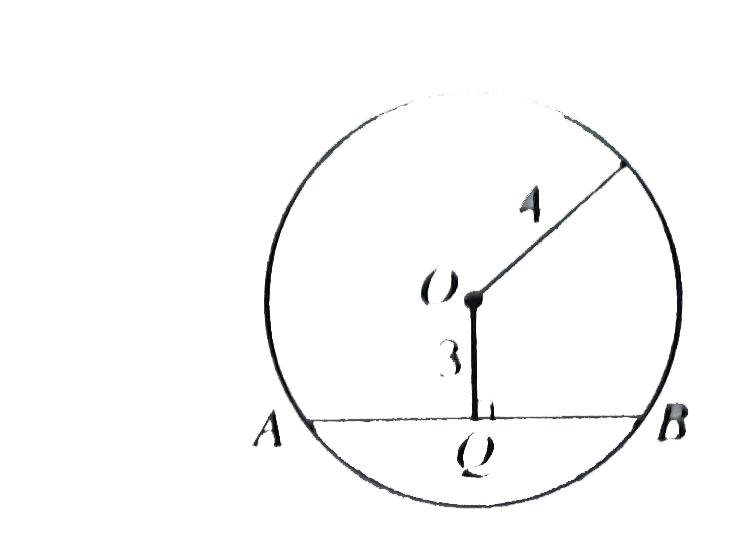
A chord ¯¯¯¯¯¯AB is 3 cm. from the center of a circle with radius 4. What is the length of chord ¯¯¯¯¯¯AB ?A√7B2√7C5D10
The vector difference between the absolute velocities of two bodies is called their relative velocity. The concept of relative velocity enables us to treat one body as being at rest while the other is in motion with the relative velocity. This jormalism greatly simplifies many problems. If →vAis the absolute velocity of a body A and →–––(B)that of another body B then the relative velocity of A in relation to B is →–––(AD)=→VA−→vB and →vBA=→vB-→vA.
The principle follows here is that the relative velocity of two bodies remains unchaged if the same additional velocity is imparted to both the bodies. A simple way of carrying out this operation is to be represent the velocities in magnitude and direction from a common point. Then the line joining the tips of the vectors represents the relative velocity
Q A train A moves to the east with velocity of 40 km/hr and a train B moves due north with velocity of 30 km/hr. The relative velocity of A w.rt. B is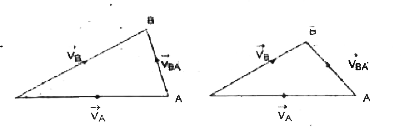 View Solution
View SolutionA boat moves relative to water with a velocity v which is n times less than the river flow velocity u. At what angle to the stream direction must the boat move to minimize drifting ?
View SolutionA boat moves relative to water with a velocity v which is n times less than the river flow velocity u. At what angle to the stream direction must the boat move to minimize drifting ?
View SolutionA boat is travelling with a speed of 27 km h−1 due east. An observer is situated at 30 m south of the line of travel. The angular velocity of the boat relative to be the observer in the position shown will be
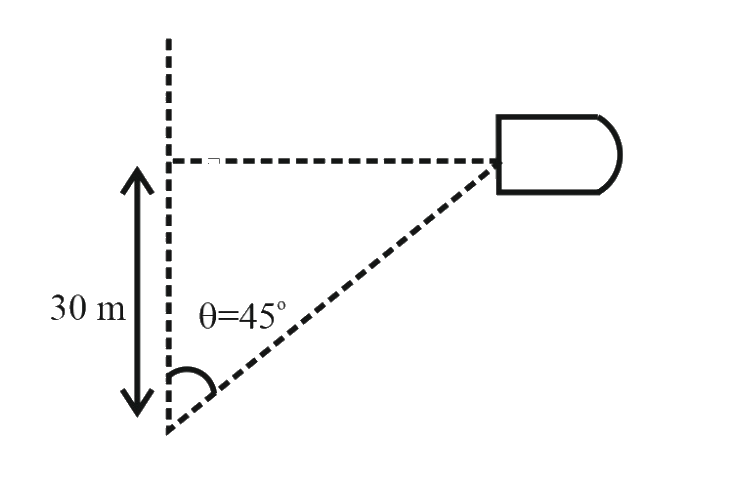 View Solution
View SolutionA motor boat is racing towards North at 25km/h and the water current in that region is 10km/h in the direction of 6000 East of South. Find the resultant velocity of the boat.
View SolutionA boat is moving with a velocity 3ˆi+4ˆj with respect to ground. The water in the river is moving with a velocity −3ˆi−4ˆj with respect to ground. The relative velocity of the boat with respect to water is.
View Solution

