Let O be the circumcenter, H be the orthocentre, I be the incenter, and I1,I2,I3 be the excenters of acute-angled ABC. Column I Column II Angle subtended by OI at vertex A p. |B−C| Angle subtended by HI at vertex A q. ∣∣∣B−C2∣∣∣ Angle subtended by OH at vertex A r. B+C2 Angle subtended by I2I3atI1 A q. B2−CSolution in Tamil
Step by step video & image solution for Let O be the circumcenter, H be the orthocentre, I be the incenter, and I_1, I_2,I_3 be the excenters of acute-angled A B Cdot Column I Column II Angle subtended by O I at vertex A p. |B-C| Angle subtended by H I at vertex A q. |(B-C)/2| Angle subtended by O H at vertex A r. (B+C)/2 Angle subtended by I_2I_3a tI_1 A q. B/2-C by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
If normal at point P on the parabola y2=4ax,(a>0), meets it again at Q in such a way that OQ is of minimum length, where O is the vertex of parabola, then OPQ is
(a)a right angled triangle (b)an obtuse angled triangle (c)an acute angle triangle (d)none of these
View SolutionIn ABC,AB=AC, and the bisectors of angles B and C intersect at point O . Prove that BO=CO and the ray AO is the bisector of angles BAC.
View SolutionThe equation to the locus of P such that the join of (a, b) and (b, a) subtend a right angle at P, is
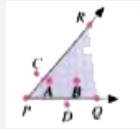
View SolutionWhich of these statements is not true ?
(a) Point A and B lie in the interior of the angle
(b) Points C and D lie in the exterior of the angle.
( c )Point P is the vertex of the angle .
(d) Point R is the vertex of the angle.
View SolutionIf the chord y=mx+c subtends a right angle at the vertex of the parabola y2=4ax, thenthe value of c is
View SolutionLet ABC be a triangle having OandI as its circumcenter and incentre, respectively. If Randr are the circumradius and the inradius, respectively, then prove that (OI)2=R2−2Rr. Further show that the triangle BIO is a right angled triangle if and only if b is arithmetic mean of aandc.
View SolutionColumn I (condition) Column II (Type of ABC) cotA2=b+ca p. always right angled atanA+btanB=(a+b)tan(A+B2) q. always isosceles acosA−bcosB r. may be right angled cosA=sinB2sinC s. may be right angled isosceles
View Solution
