Question

In triangle ${\displaystyle ABC,\angle A={60}^0,\angle B={40}^0,and\angle C={80}^0\stackrel{.}{}}$
If ${\displaystyle P}$
is the center of the circumcircle of triangle ${\displaystyle ABC}$
with radius unity, then the radius of the circumcircle of triangle ${\displaystyle BPC}$
is
1 (b) ${\displaystyle \sqrt{3}}$
(c) 2 (d) ${\displaystyle \sqrt{3}}$
${\displaystyle 2}$

Explanation

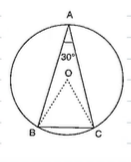
With the given details, we can create a diagram.

Please refer to video for the diagram.

Here, circumradius of `Delta ABC` = `sqrt16 = 4`

`:. a = 2*4sinA =>a = 8sinA->(1)`

Let `R_1` is the circumradius of the `Delta HBC`.

Then,

`a = 2R_1sin/_BHC`

`=2R_1sin(B+C)`

`=2R_1sin(pi-A)`

`:. a=2R_1sinA->(2)`

From (1) and (2),

`2R_1sinA = 8sinA`

`=>2R_1 = 8`

`:.` Circumdiameter of `Delta HBC = 2R_1 = 8.`