From the graph of y=x2−4, draw the graph of y=1x2−4.Solution in Tamil
y=f(x)=x2−4 is an upward parabola having vertex at (0, -4).
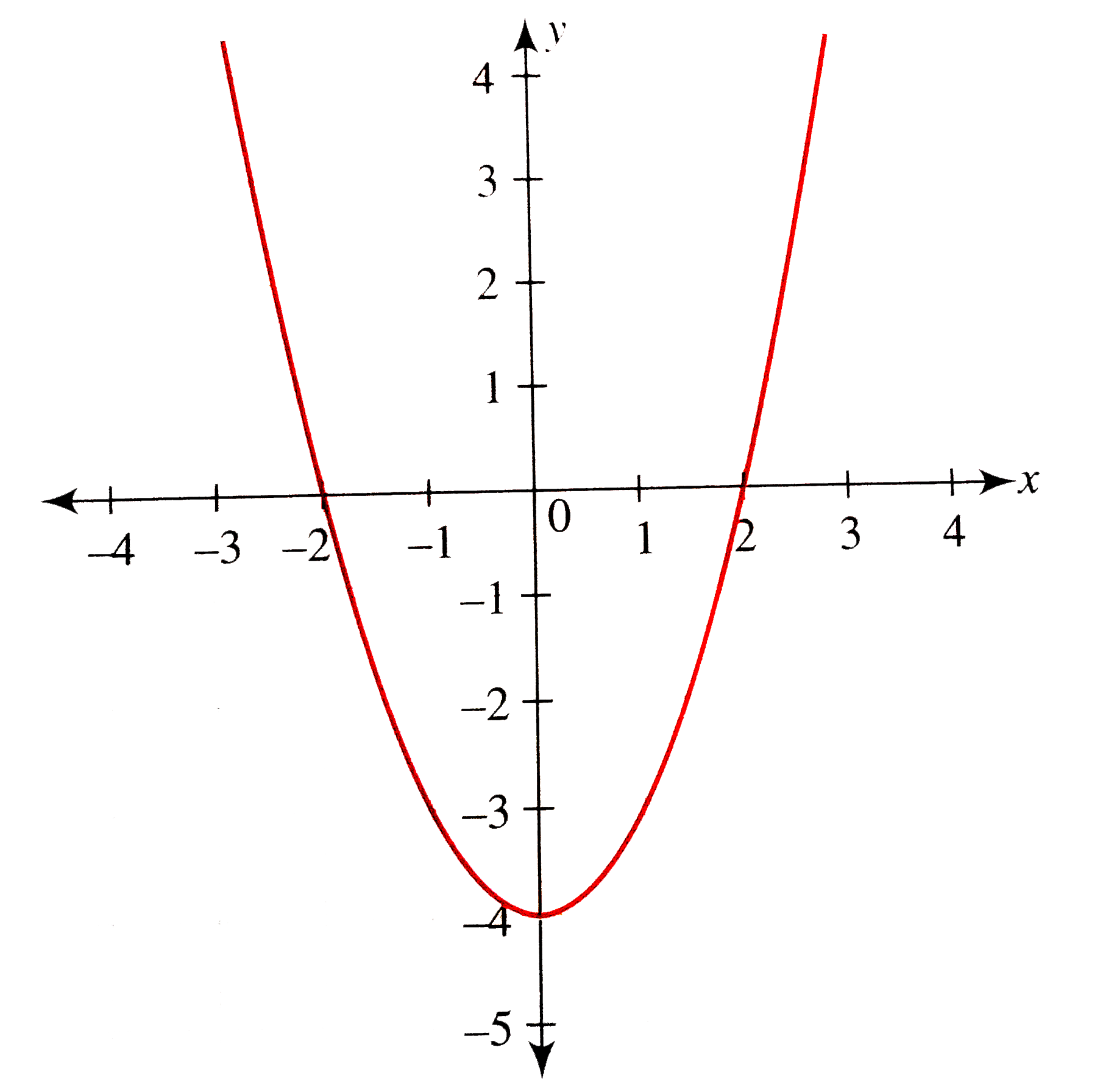
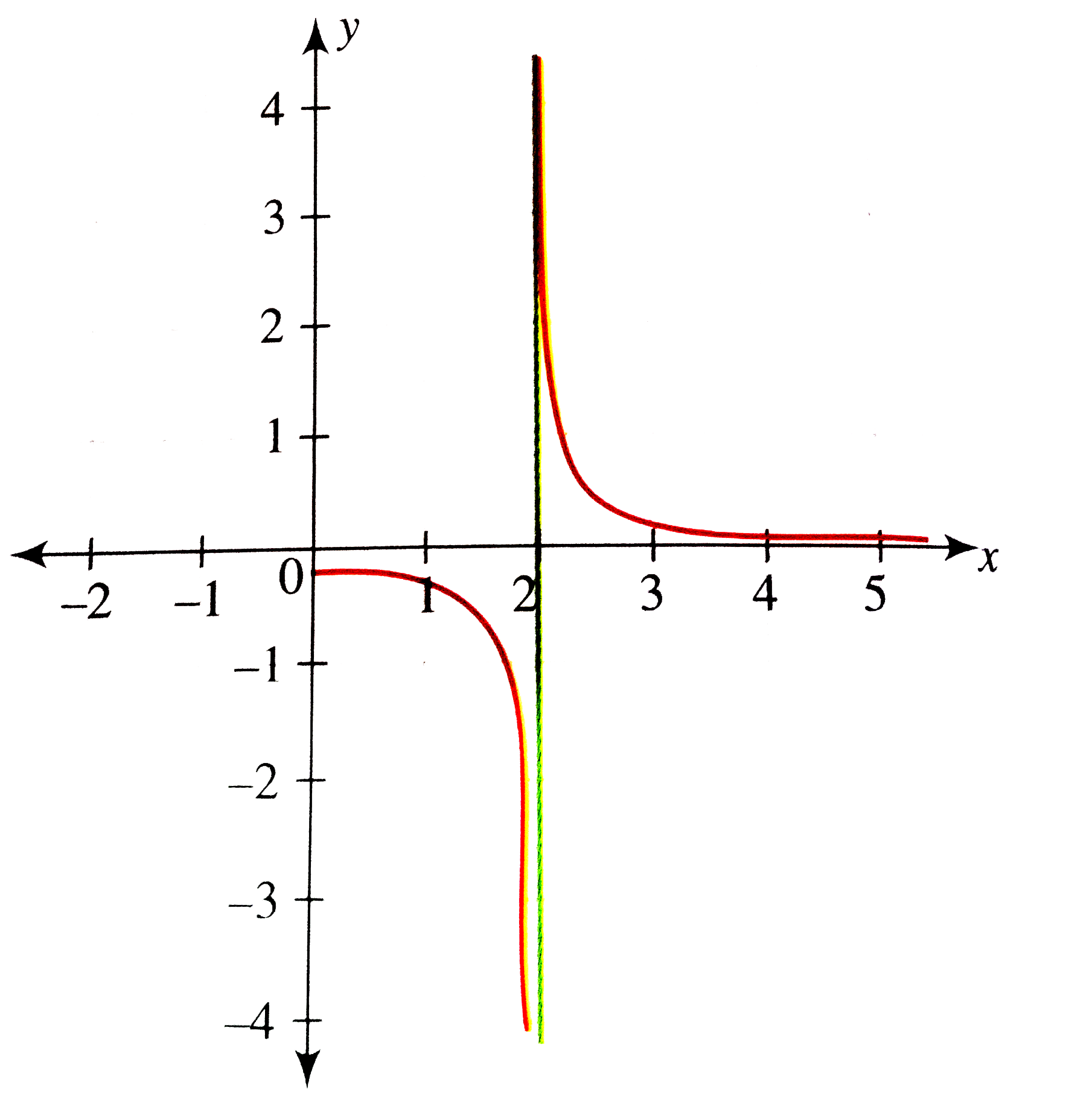
Since the function is even, let us draw the graph of y=f(x) for x∈[0,∞)−{2}.
For [0,2),x2−4 increases from -4 to 0.
So 1x2−4 decreases from -1/4 to −∞.
For (2,∞),x2−4 increases from 0 to ∞.
So 1x2−4 decreases from ∞ to 0.
So the graph of y=f(x) for x∈[0,∞)−{2} is as shown in the following figure.
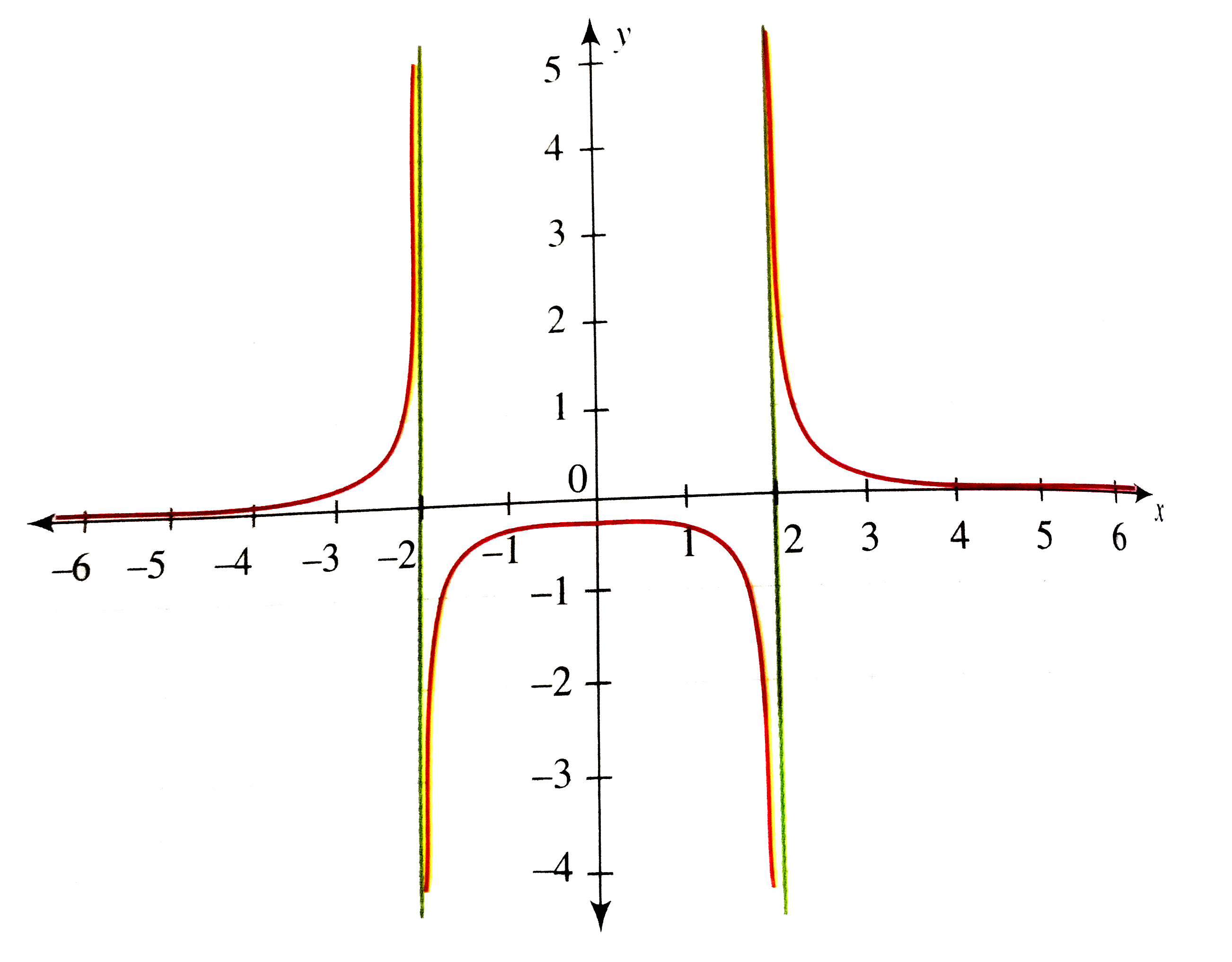
Since y=g(x) is an even function, the graph for R-{-2,2} can be drawn as shown in the following figure.
Topper's Solved these Questions
- GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSEXERCISE - Exercise
13 Videos
- GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSEXERCISE - Exercises
4 Videos
- GRAPHICAL TRANSFORMATIONSBOOK - CENGAGECHAPTER - GRAPHICAL TRANSFORMATIONSEXERCISE - Exercise
14 Videos
- GRAPHS OF ELEMENTARY FUNCTIONS BOOK - CENGAGECHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - Exercise
34 Videos
Similar Questions
Draw the graph of y=(x−1)(x2−x+1).
View SolutionSketch the graph of an example of a rational function f that satisfies all the given conditions.
(i) f(0)=0,f(1),limx→∞f(x)=0,f is odd
(ii) limx→0+f(x)=∞,limx→0−f(x)=−∞,limx→∞f(x)=1,limx→−∞f(x)=1
(iii) limx→−2f(x)=∞,limx→−∞f(x)=3,limx→∞f(x)=3,f(0)=0
View SolutionDraw the graph of y=tan−1x−cot−1x.
View SolutionDraw the graph of y=1(1/x).
View SolutionDraw the graph : x+y=4
View SolutionDraw the graph of y=x−1x2 and hence the graph of y=|x−1|x2.
View SolutionFrom the graph of y=x2−4, draw the graph of y=1x2−4.
View SolutionDraw graph of y=1x2−x.
View SolutionDraw the graph of y=x−1x−2.
View SolutionDraw the graph for |y|=(x−1)(x−2).
View SolutionDraw the graph of y=x−1x2 and hence the graph of y=|x−1|x2.
View SolutionFrom the graph of y=x2−4, draw the graph of y=1x2−4.
View SolutionDraw graph of y=1x2−x.
View SolutionDraw the graph of y=x−1x−2.
View SolutionDraw the graph of the function y=1x for x:-4≤x≤4
View Solution
