ABCD is a square whose vertices are A(0,0),B(2,0),C(2,2), and D(0,2) . The square is rotated in the XY−plane through an angle 300 in the anticlockwise sense about an axis passing though A perpendicular to the XY−plane . Find the equation of the diagonal BD of this rotated square.
We have
B≡(2cos30∘,2sin30∘)=(√3,1)
D≡(−2sin30∘,2cos30∘)=(−1,√3)
Hence, the equation of BD is
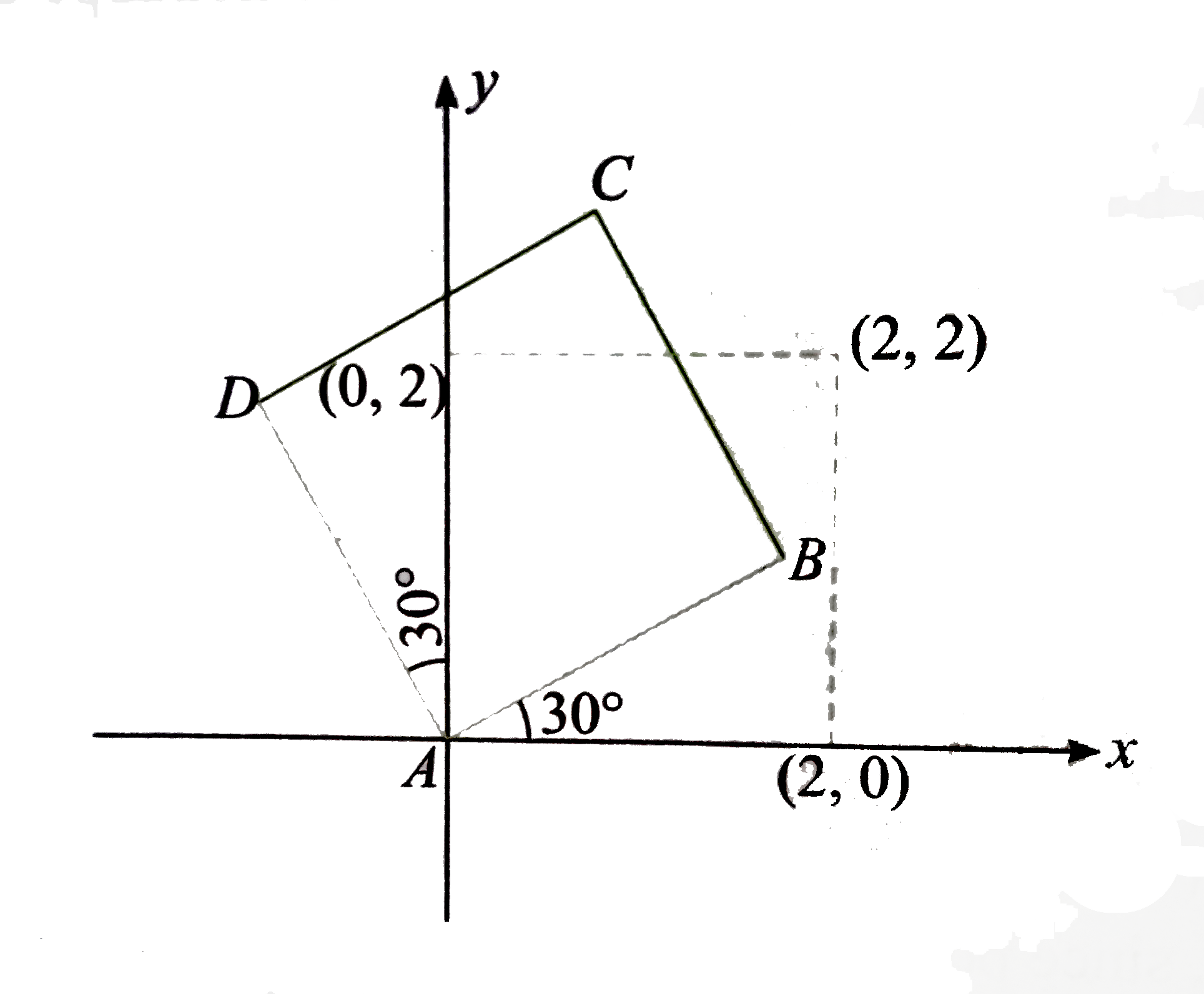
y−1=√3−1−(√3+1)(x−√3)
=(√3−2)(x−√3)
i.e., (2−√3)x+y=2√3−2
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise 2.123videos
STRAIGHT LINES
- BOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise 2.24videos
STRAIGHT LINES
- BOOK - CENGAGECHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type8videos
STRAIGHT LINE
- BOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - JEE Advanced Previous Year9videos
THEORY OF EQUATIONS
Similar Questions
The line segment joining A(3,0)andB(5,2) is rotated about A in the anticlockwise direction through an angle of 450 so that B goes to C. If D is the reflection of C in y-axis, find the coordinates of D.
View SolutionThe line segment joining A(3,0)andB(5,2) is rotated about A in the anticlockwise direction through an angle of 450 so that B goes to C. If D is the reflection of C in y-axis, find the coordinates of D.
View SolutionThe line PQ whose equation is x−y=2 cuts the x-axis at P ,and Q is (4,2). The line PQ is rotated about P through 45∘ in the anticlockwise direction. The equation of the line PQ in the new position is (A) y=−√2 (B) y=2 (C) x=2 (D) x=−2
View SolutionThe line segment joining A(3,0),B(5,2) is rotated about a point A in anti clcokwise sense through an angle π4 and B move to C. if a point D be the reflection of C in y-axis, then D=
View SolutionABC is an equilateral triangle with A(0,0) and B(a,0) , (a>0). L, M and N are the foot of the perpendiculars drawn from a point P to the side AB,BC,andCA , respectively. If P lies inside the triangle and satisfies the condition PL2=PM.PN, then find the locus of P.
View SolutionABC is an equilateral triangle with A(0,0) and B(a,0) , (a>0). L, M and N are the foot of the perpendiculars drawn from a point P to the side AB,BC,andCA , respectively. If P lies inside the triangle and satisfies the condition PL2=PM.PN, then find the locus of P.
View SolutionThe medians AD and BE of a triangle with vertices A(0,b), B(0,0) and C(a,0) are perpendicular to each other, if a=b2 b. b=a2 c. ab=1 d. a=±√2b
View SolutionThe medians AD and BE of a triangle with vertices A(0,b), B(0,0) and C(a,0) are perpendicular to each other, if a=b2 b. b=a2 c. ab=1 d. a=±√2b
View SolutionIf A(0,4,1),B(a,b,c),C(4,5,0),D(2,6,2) are the consecutive vertices of a square,and the distance BD is l then l√2
View SolutionIf A(0,4,1),B(a,b,c),C(4,5,0),D(2,6,2) are the consecutive vertices of a square,and the distance BD is l then l√2=______
View Solution

