Two adjacent vertices of a square are (1, 2) and (−2,6) Find the other vertices.
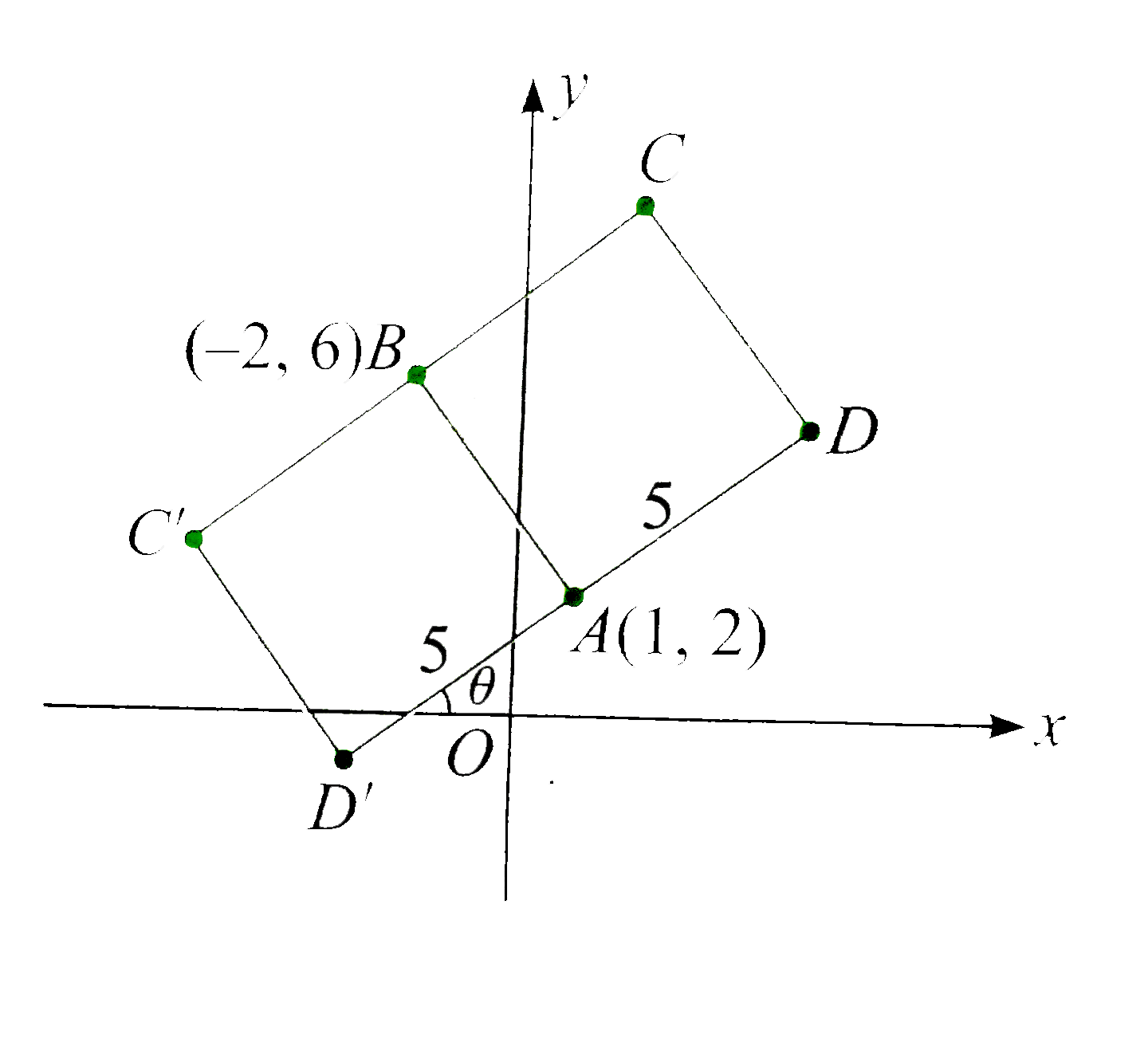
Given two adjacent vertices are A (1,2) and B(-2,6). Then,
AB = 5
Also, the slope of AB is
mAB=6−2−2−1=−43
Slope of AD=34=tan θ
The coordinates of D and D' are
(1±5cosθ,2±5sinθ)≡(1±545,2±535)
or D(5,5) and D'(-3-1)
Similarly, the coordinates of C and C' are
(−2±5cosθ,6±5sinθ)≡(−2±545,6±535)
or C(2,9) and C'(-6,3)
Topper's Solved these Questions
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise 2.1
23 Videos
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise 2.2
4 Videos
- STRAIGHT LINEBOOK - CENGAGECHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
- THEORY OF EQUATIONSBOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - JEE Advanced Previous Year
9 Videos
Similar Questions
The vertices of a diagonal of a square are (−2,4) and (−2,−2) Find the other vertices
View SolutionThe vertices of a diagonal of a square are (−2,4) and (−2,−2) Find the other vertices
View SolutionTwo opposite vertices of a square are (−1, 2) and (3, 2) . Find the coordinates of other two vertices.
View SolutionTwo opposite vertices of a square are (0,−2) (2,6) . Find the coordinates of the other vertices.
View SolutionThe two opposite vertices of a square are (−1,2) and (3,2). Find the coordinates of the other two vertices.
View SolutionThe two opposite vertices of a square are (1, 2) and (3, 2). Find the coordinates of the other two vertices.
View SolutionTwo opposite vertices of a square are (−1, 2) and (3, 2) . Find the coordinates of other two vertices.
View SolutionIf two adjacent vertices of a parallelogram are (3, 2) and (-1, 0) and the diagonals intersect at (2, -5), then find the coordinates of the other two vertices.
View SolutionIf two adjacent vertices of a parallelogram are (3, 2) and (-1, 0) and the diagonals intersect at (2, -5), then find the coordinates of the other two vertices.
View SolutionIf two adjacent vertices of a parallelogram are (3, 2) and (-1, 0) and the diagonals intersect at (2, -5), then find the coordinates of the other two vertices.
View Solution
