The equation of straight line passing through (-2,-7) and having an intercept of length 3 between the straight lines : 4x + 3y = 12 , 4x + 3y = 3 are : (A) 7x + 24y + 182 = 0 (B) 7x + 24y + 18 = 0 (C) x + 2 = 0 (D) x - 2 = 0

Text Solution

Verified by Experts

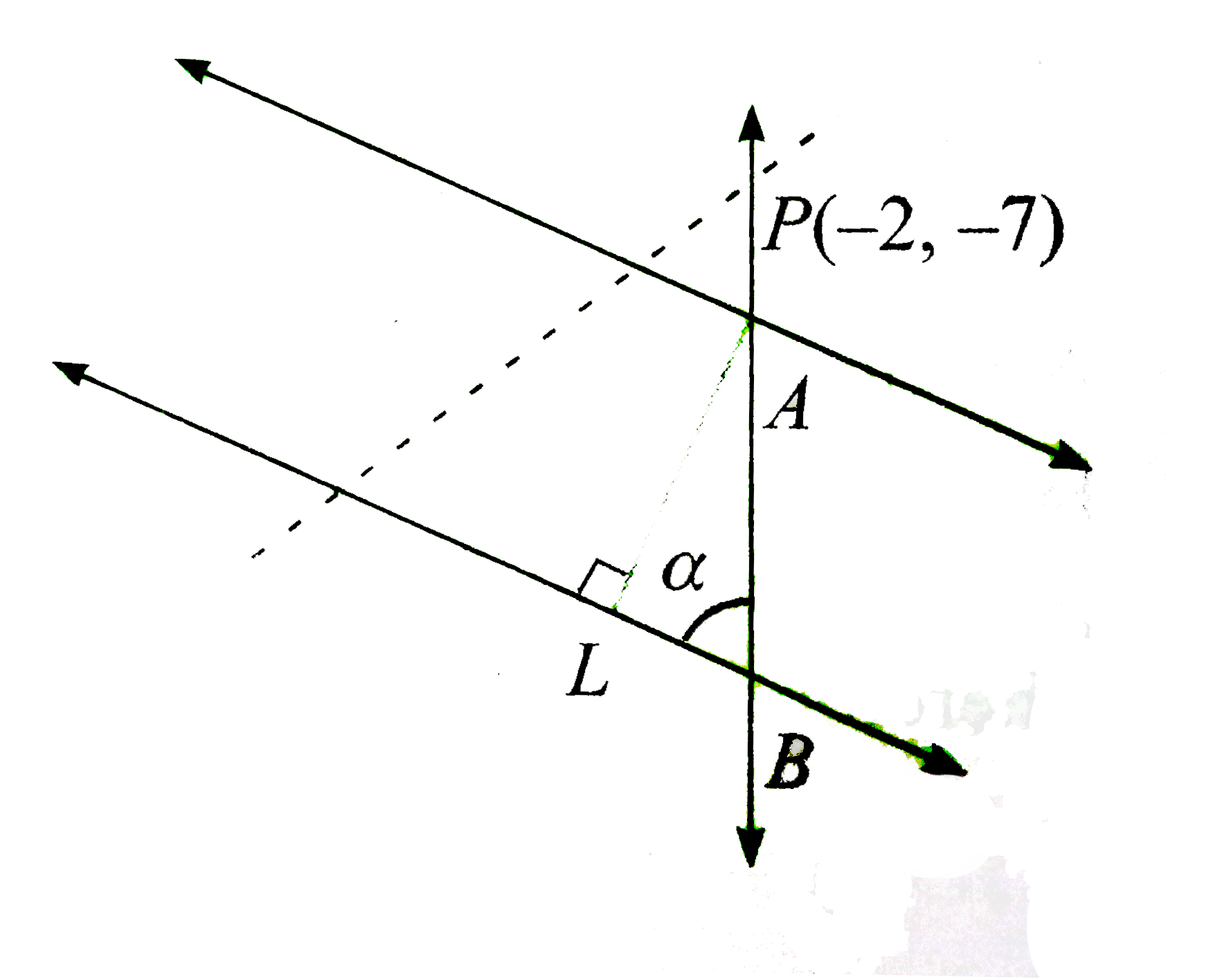
Given lines are
$4 x + 3 y - 12 = 0 (1)$
$and 4 x + 3 y - 3 = 0 (2)$
Given AB=3.
The distance between parallel lines (1) and (2),
$A L = \frac{| - 12 + 3 |}{\sqrt{4^{2} + 3^{2}}} = \frac{9}{5}$
$From Δ ALB, we get$
$L B^{2} = A B^{2} - A L^{2}$
$= 3^{2} - \frac{81}{25} = 9 \times \frac{16}{25}$
$∴ L B = \frac{12}{5}$
$\tan α = \frac{A L}{L B} = \frac{3}{4}$
$Also, tan θ = Slope of line (1) = - \frac{4}{3}$
Let the slope of PB be m. Now,
$\tan α = \frac{3}{4} = | \frac{m - (- 4 / 3)}{1 + m (- 4 / 3)} | = | \frac{3 m + 4}{3 - 4 m} |$
$or m = \infty or m = - \frac{7}{24}$
Therefore, the equation of the line is
$x + 2 = 0 and y + 7 = - \frac{7}{24} (x + 2)$
$or x + 2 = 0 and 7 x + 24 y + 182 = 0$

Updated on:21/07/2023