Doubtnut Promotions Sticky

Sketch the origin in which the points satisfying the following inequality lie.
(i)2x−3y−5>0 (ii) −3x+4y+7>0
(iii)x>2 (iv) y>−3

Text Solution
Verified by Experts

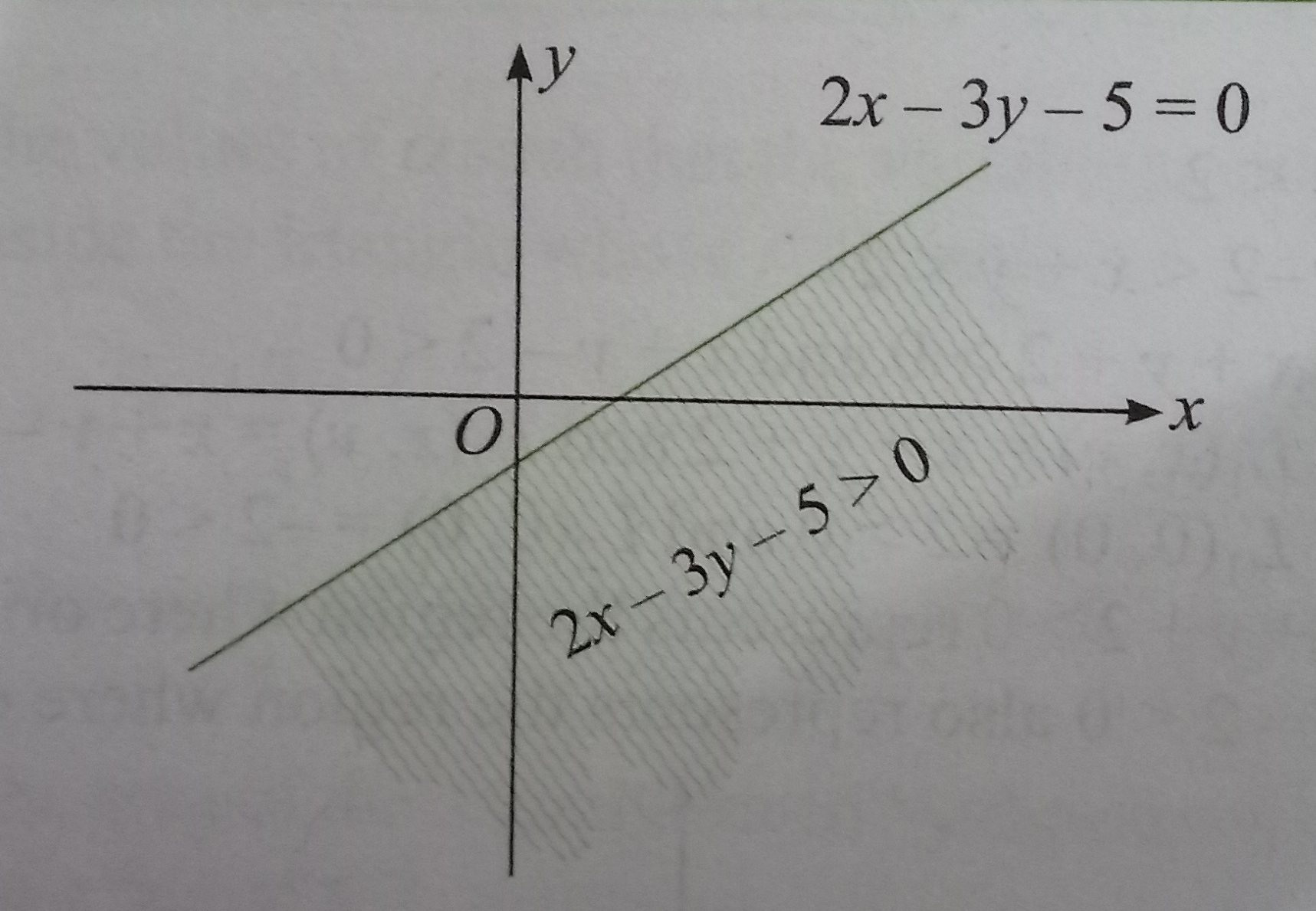
(i)2x−3y−5>0
Let L(x,y)=2x−3y−5
L(0,0)=−5<0
So,2x−3y−5>0 represents the half origin of the plane where origin does not lie, i.e., below line 2x-3y-5=0.
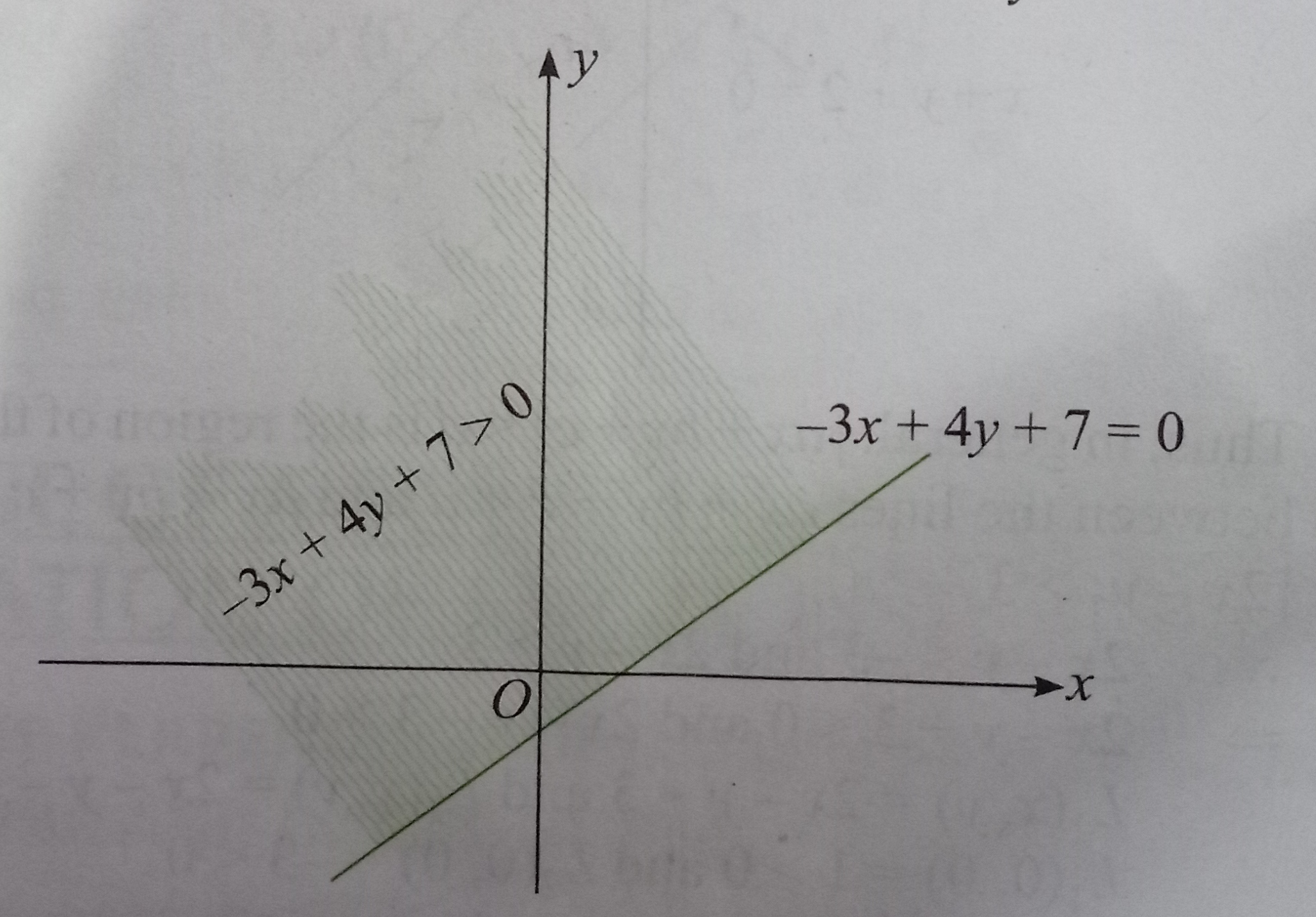
ocr_image
(ii)−3x+4y+7>0
Let L(x,y) = -3x+4y+7
L(0,0)=7>0
SO, −3x+4y+7>0 represents the half region of the plane where origin lies, i.e., above line -3x+4y+7=0.
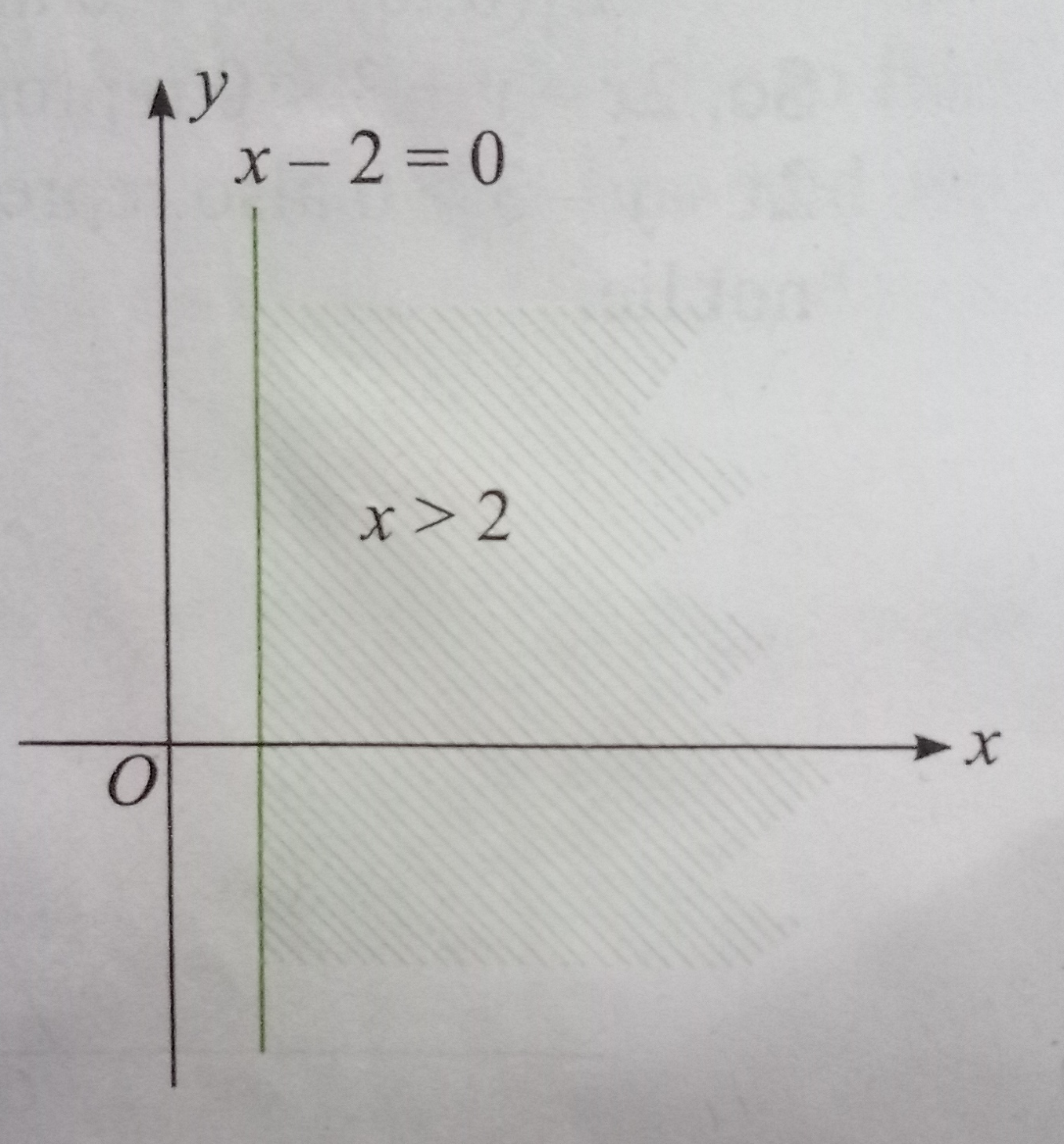
ocr_image
ocr_image
(iii)x>2orx−2>0
Let L(x,y) = x-2
L(0,0)=0−2<0
So, x−2>0 represents the half region of the plane where origin does not lie, i.e., the right of the line x=2.
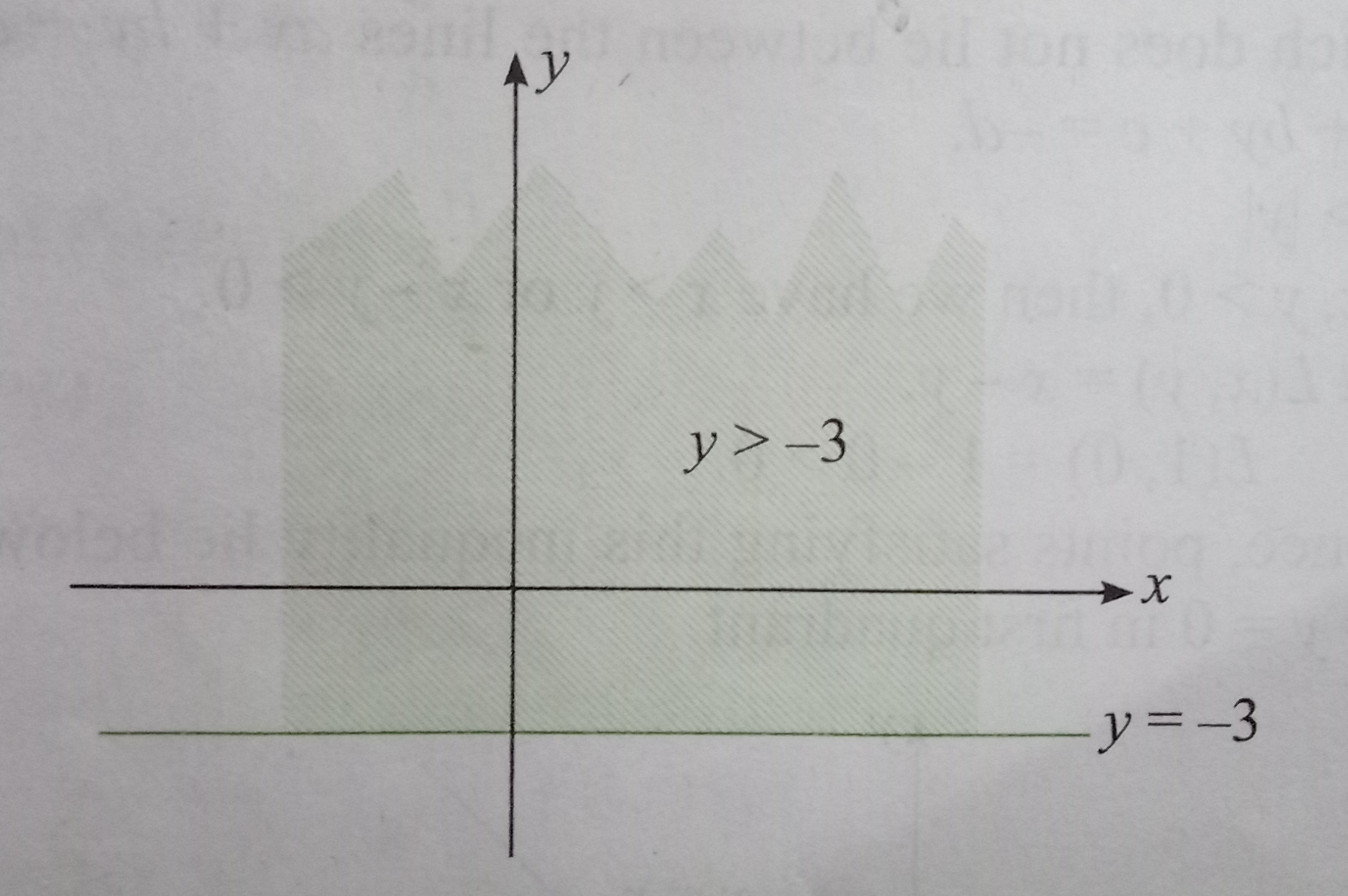
(iv)y>−3ory+3>0
Let L(x,y) = y+3
L(0,0)=0+3>0
So,y+3>0 represents the half region of the plane where origin lies, i.e., above the line y+3=0.
ocr_image

Doubtnut Promotions Banner
|
Updated on:21/07/2023
Class 12MATHSSTRAIGHT LINES

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One

    The ordered pairs (3,-1) satisfies which of the following inequalities?
    I. x+3y>0
    II. 2x+3y>2
    III. x+y<0

    AI only
    BII only
    CI and III only
    DII and III only
  • Question 2 - Select One

    Is x−y>0 ?
    I. x>y
    II. x2>y2

    AIf statement (I) alone is sufficient to answer the question.
    BIf statement (II) alone is sufficient to answer the question.
    CIf either (I) or (II) alone is sufficient to answer the question.
    DIf both statements (I) and (II) taken together are sufficient to answer the question.
  • Question 3 - Select One

    Which of the following is a corner point of the feasible region of system of linear inequations 2x + 3y ≤6,x+4y≤4 and x, y ≥0 ?

    A(1,0)
    B(1,1)
    C(0,3)
    D(125,25)