The vertices BandC of a triangle ABC lie on the lines 3y=4xandy=0 , respectively, and the side BC passes through the point (23,23) . If ABOC is a rhombus lying in the first quadrant, O being the origin, find the equation of the line BC.
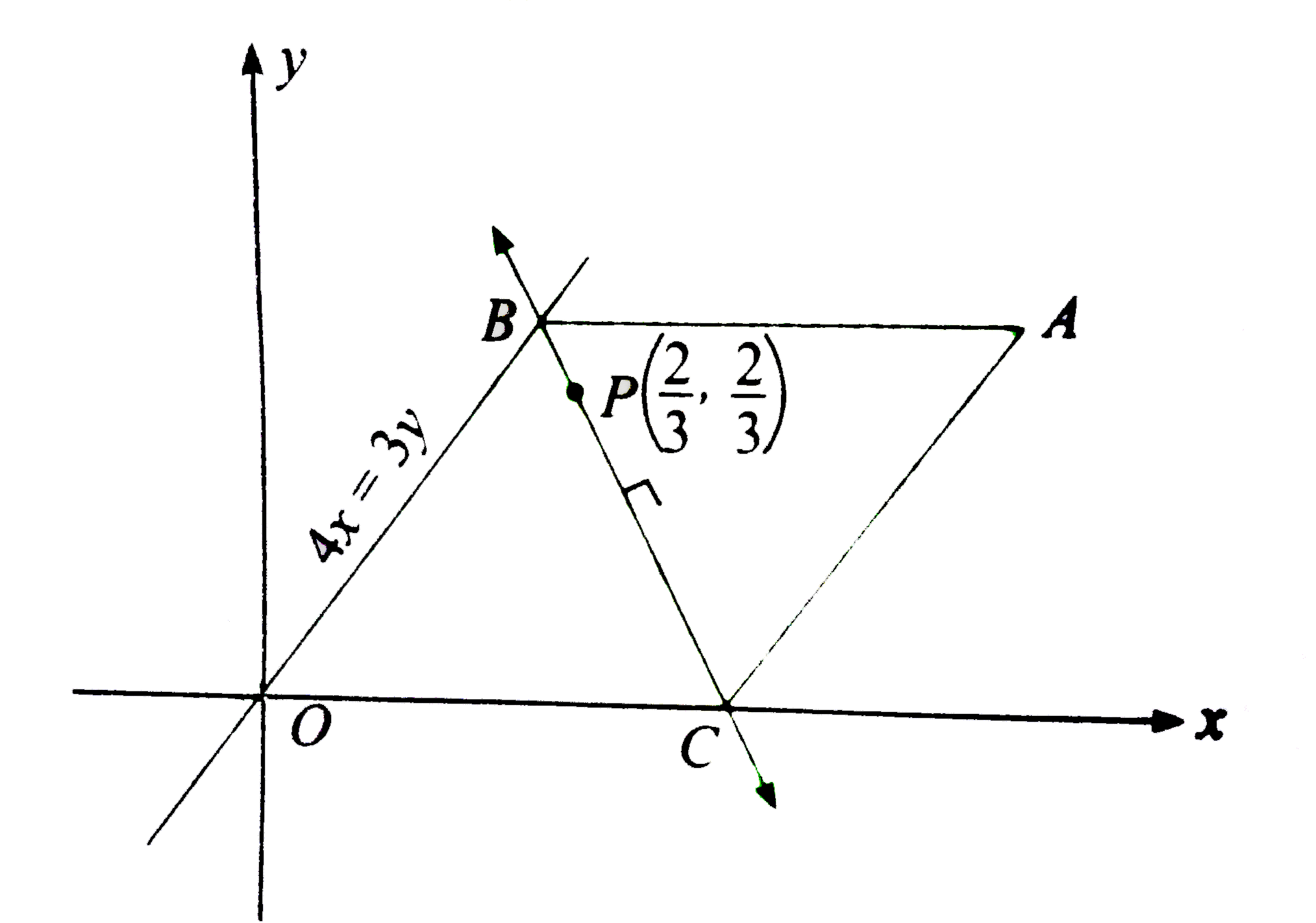
Diagonals of rhombus are equally inclined to the sides.
So, diagonals are parallel to or coincident with the angle bisectors of sides.
Equations of bisectors of sides OC and OB of rhombus are
4x−3y5=±y1
or x-2y=0 and 2x+y=0
Clearly, diagonal BC is parallel to the bisector 2x+y=0.
So, equation BC is
y−23=−2[x−23]
or 2x+y-2=0
Topper's Solved these Questions
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise 2.1
23 Videos
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise 2.2
4 Videos
- STRAIGHT LINEBOOK - CENGAGECHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
- THEORY OF EQUATIONSBOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - JEE Advanced Previous Year
9 Videos
Similar Questions
The vertices BandC of a triangle ABC lie on the lines 3y=4xandy=0 , respectively, and the side BC passes through the point (23,23) . If ABOC is a rhombus lying in the first quadrant, O being the origin, find the equation of the line BC.
View SolutionThe vertices BandC of a triangle ABC lie on the lines 3y=4xandy=0 , respectively, and the side BC passes through the point (23,23) . If ABOC is a rhombus lying in the first quadrant, O being the origin, find the equation of the line BC.
View Solution
Knowledge Check
A straight line passing through the point A(−2,−3) cuts lines x+3y=9 and x+y+1=0 at B and C, respectively. If AB.AC=20, then equation of the possible line is
Ax−y=1Bx−y+1=0C3x−y+3=0D3x−y=3
The vertices BandC of a triangle ABC lie on the lines 3y=4xandy=0 , respectively, and the side BC passes through the point (23,23) . If ABOC is a rhombus lying in the first quadrant, O being the origin, find the equation of the line BC.
View SolutionThe vertices B and C of a triangle ABC lie on the lines 3y=4x and y=0 respectively and the side BC passes through the point (23,23)If ABOC is a rhombus, O being the origin. If co-ordinates of vertex A is (α,β) , then find the value of 52(α+β).
View SolutionThe vertices B,C of a triangle ABC lie on the lines 4y=3xandy=0 respectively and the side BC passes through thepoint P(0,5). If ABOC is a rhombus, where O is the origin and the point P is inside the rhombus, then find the coordinates of A'.
View SolutionThe vertices B,C of a triangle ABC lie on the lines 4y=3xandy=0 respectively and the side BC passes through thepoint P(0,5). If ABOC is a rhombus, where O is the origin and the point P is inside the rhombus, then find the coordinates of A\'.
View SolutionThe sides ABandAC of a triangle ABC are respectively 2x+3y=29andx+2y=16 respectively. If the mid-point of BCis(5,6) then find the equation of BC.
View SolutionThree sides AB,ACandCA of triangle ABC are5x−3y+2=0,x−3y−2=0andx+y−6=0 respectively. Find the equation of the altitude through the vertex A.
View SolutionA straight line through the point A(−2,−3) cuts the line x+3y=9 and x+y+1=0 at BandC respectively. Find the equation of the line if AB.AC=20.
View Solution
