Let ABCDEFGHIJKL be a regular dodecagon. Then the value of ABAF+AFAB is equal to ____Solution in Tamil
The correct Answer is:4
AM=Rsin.π12orABsin.π12
Similarly, AN=Rsin.5π12⇒AF=2Rsin.5π12

∴ABAF+AFAB=sin.π12sin.5π12+sin.5π12sin.π12
=tan.π12+cot.π12
=(2−√3)+(2+√3)=4
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - JEE Main Previous Year2videos
PROPERTIES AND SOLUTIONS OF TRIANGLE
- BOOK - CENGAGECHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - JEE Advanced Previous Year11videos
PROPERTIES AND SOLUTIONS OF TRIANGLE
- BOOK - CENGAGECHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Exercise (Matrix)6videos
PROPERTIES AND SOLUTIONS OF TRIANGLE
- BOOK - CENGAGECHAPTER - PROGRESSION AND SERIESEXERCISE - ARCHIVES (MATRIX MATCH TYPE )1videos
PROGRESSION AND SERIES
- BOOK - CENGAGECHAPTER - PROPERTIES OF TRIANGLE, HEIGHT AND DISTANCEEXERCISE - Question Bank15videos
PROPERTIES OF TRIANGLE, HEIGHT AND DISTANCE
Similar Questions
For a regular polygon, let r and R be the radii of the inscribed and the circumscribed circles. A false statement among the following is There is a regular polygon with rR=1√2 (17) There is a regular polygon with rR=23 (30) There is a regular polygon with rR=√32 (47) There is a regular polygon with rR=12 (60)
03:26View SolutionLet ABC be a triangle such that ∠ACB=π6 and let a,bandc denote the lengths of the side opposite to A,B,andC respectively. The value(s) of x for which a=x2+x+1,b=x2−1,andc=2x+1 is(are) −(2+√3) (b) 1+√3 2+√3 (d) 4√3
02:40View SolutionFind the derivative of y=sin−1(2–x4).
View SolutionIt is given that in a group of 4 students, the probability of 2 students not having the same birthday is 0.893. What is the probability that the 2 students have the same birthday?
03:19View SolutionThe area of a right triangle is 6864 sq. units. If the ratio of its legs is 143:24, then the value of r is ______
05:54View SolutionIf ABCDEF is a regular hexagon inscribed in a ( circle with centre O, then the value of )/(AB)+bar(AC)+bar(AD)+( then the value of )/(AF) equals
03:11View SolutionIn a regular hexagon ABCDEF, prove that −−→AB+−−→AC+−−→AD+−−→AE+−−→AF=3−−→AD
04:32View SolutionLet ABCDEFGHIJKL be a regular dodecagon. Then the value of ABAF+AFAB is equal to ____
02:34View SolutionIf ABCDEF is a regular hexagon , then
¯¯¯¯¯¯AB+¯¯¯¯¯¯AC+¯¯¯¯¯¯AE+¯¯¯¯¯¯AF =03:43View SolutionIf ABCDEF is a regular hexagon, then ¯¯¯¯¯¯AB+¯¯¯¯¯¯AC+¯¯¯¯¯¯AE+¯¯¯¯¯¯AF=
01:48View SolutionIf ABCDEF is a regular hexagon and AB+AC+AD+AE+AF=λAD then λ is equal to
02:53View SolutionIf ABCDEF is a regular hexagon of side a, then ¯¯¯¯¯¯AB.¯¯¯¯¯¯AF=
05:17View SolutionIn the given figure, AB|| CD. Find the value af x.
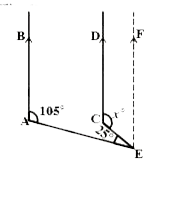 06:20View Solution
06:20View SolutionIn the given figure, AB|| CD. Find the value af x.
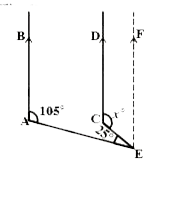 03:03View Solution
03:03View SolutionIn the given figure, AB|| CD. Find the value af x.
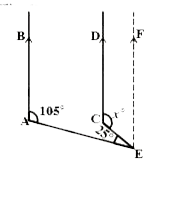 07:54View Solution
07:54View SolutionIn the given figure, AB|| CD. Find the value af x.
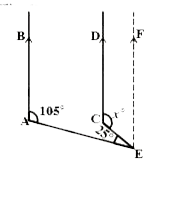 04:19View Solution
04:19View Solution

