Complex numbers z1 and z2 satisfy |z1|=2 and |z2|=3. If the included angle of their corresponding vectors is 60∘, then the value of 19∣∣∣z1−z2z1+z2∣∣∣2 isSolution in Tamil
5
6
7
8
The correct Answer is:C
(c)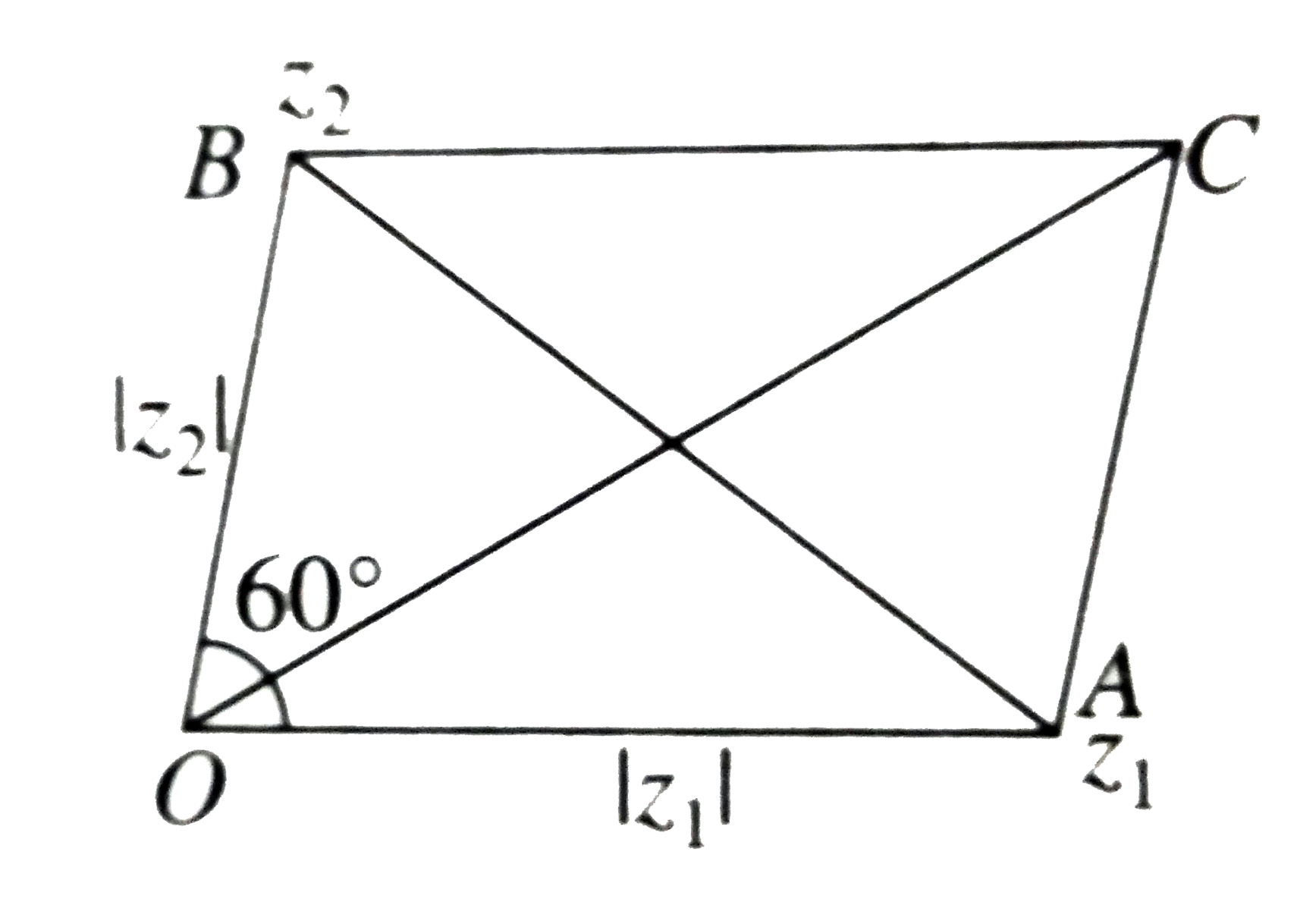 
OC=|z1+z2| and AB=|z1−z2|
|z1+z2|=√|z1|2+|z2|2−2|z1||z2|cos120∘
=√4+9+2.3=√19
and |z1−z2|=√|z1|2+|z2|2−2|z1||z2|cos60∘
=√4+9−6=√7
∴∣∣∣z1−z2z1+z2∣∣∣=√719
∴19∣∣∣z1−z2z1+z2∣∣∣2=7
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - COMPLEX NUMBERSEXERCISE - Multiple Correct Answer11videos
COMPLEX NUMBERS
- BOOK - CENGAGECHAPTER - COMPLEX NUMBERSEXERCISE - Matching Column1videos
COMPLEX NUMBERS
- BOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank16videos
CIRCLES
- BOOK - CENGAGECHAPTER - CONIC SECTIONSEXERCISE - Solved Examples And Exercises87videos
CONIC SECTIONS
Similar Questions
Complex numbers whose real and imaginary parts x and y are integers and satisfy the equation 3x2−|xy|−2y2+7=0
02:25View Solutionz1, z2, z3 are vertices of a triangle. Match the condition in List I with type of triangle in List II.
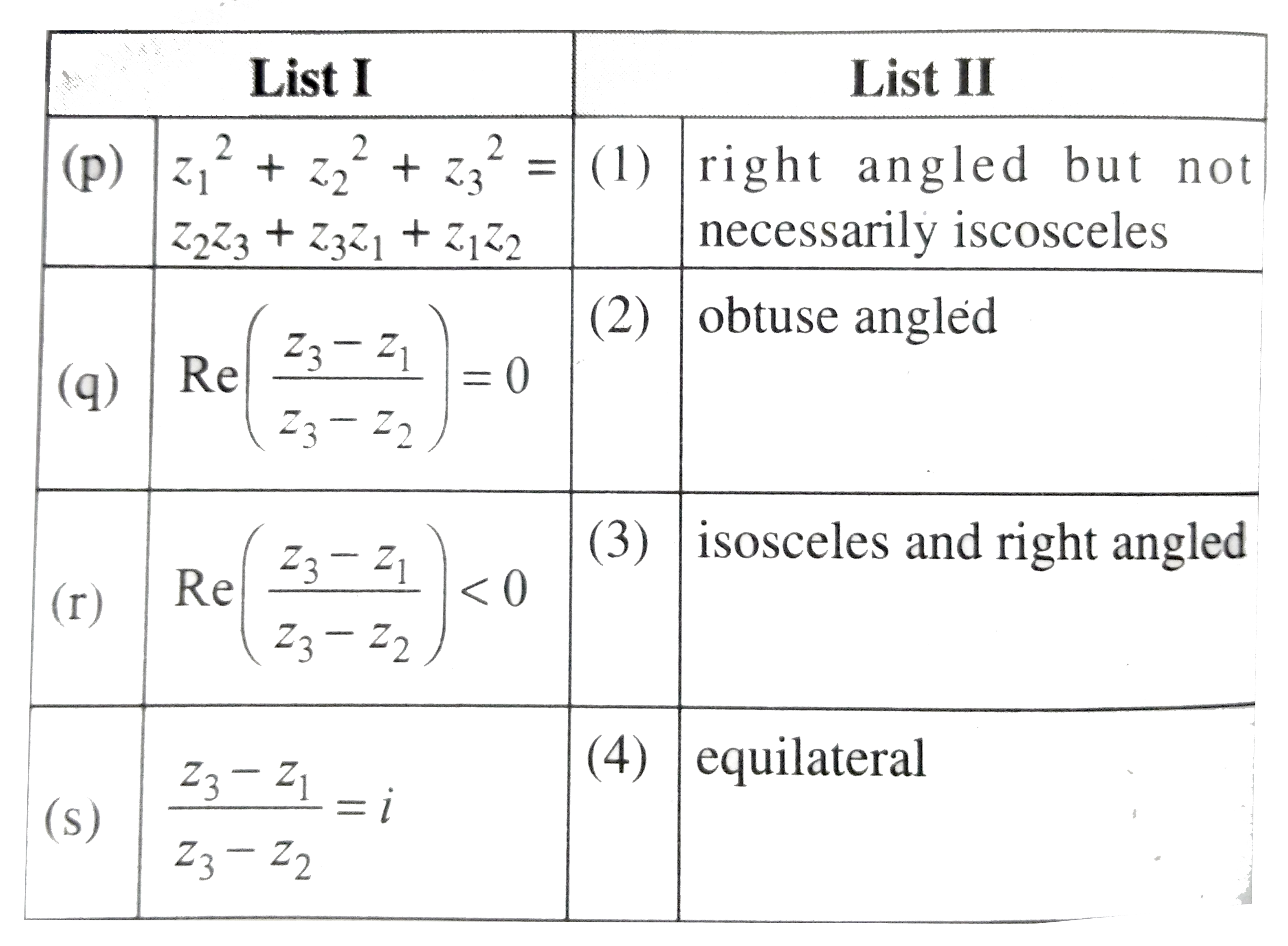
Codes03:46View SolutionIf ax+by=10 is the chord of minimum length of the circle (x−10)2+(y−20)2=729 and the chord passes through (5,15) then the value of (4a+2b) is
05:43View SolutionGiven that A(1,1) and B(2,−3) are two points and D is a point on AB produced such that AD=3AB. Find the coordinates of D.
01:40View SolutionIf z_(1) and z_(2), are two complex numbers such that |z_(1)|=|z_(2)|+|z_(1)-z_(2)|,|z_(1)|>|z_(2)|, then
05:51View SolutionComplex numbers z1 and z2 satisfy |z1|=2 and |z2|=3. If the included angle of their corresponding vectors is 60∘, then the value of 19∣∣∣z1−z2z1+z2∣∣∣2 is
View SolutionIt is given the complex numbers z1 and z2, |z1|=2 and |z2|=3. If the included angle of their corresponding vectors is 60∘ , then find value of ∣∣∣z1+z2z1−z2∣∣∣
04:41View SolutionConsider the complex numbers z1 and z2 Satisfying the relation |z1+z2|2=|z1|+|z2|2
Complex number z1/z2 is04:22View SolutionIf z1,z2 and z3 be unimodular complex numbers, then the maximum value of |z1−z2|2+|z2−z3|2+|z3−z1|2, is
05:56View SolutionStatement-1, For any two complex numbers z1 and z2
∣∣z1+√z21−z22∣∣+∣∣z1−√z21−z22∣∣=|z1+z2|+|z1−z2|
Statement-2: For any two complex numbers z1 and z2
|z1+z2|2+|z1−z2|2=2(|z1|2+|z2|2)03:53View SolutionIf z1,z2,andz3 are three complex numbers such that |z1|=1, |z2|=2, |z3|=3and|z1+z2+z3|=1, show that |9z1z2+4z1z3+z2z3|=6.
03:51View SolutionComplex numbers z1 and z2 satisfy |z1|=2 and |z2|=3. If the included angle of their corresponding vectors is 60∘, then the value of 19∣∣∣z1−z2z1+z2∣∣∣2 is
03:20View SolutionIt is given the complex numbers z1 and z2, |z1|=2 and |z2|=3. If the included angle of their corresponding vectors is 60∘ , then find value of ∣∣∣z1+z2z1−z2∣∣∣
02:24View SolutionConsider the complex numbers z1 and z2 Satisfying the relation |z1+z2|2=|z1|2+|z2|2 Complex number z1z2 is
View SolutionConsider the complex numbers z1 and z2 Satisfying the relation |z1+z2|2=|z1|+|z2|2
Complex number z1/z2 is05:13View Solution