Doubtnut Promotions Sticky

The range of the function f(x)=|x−1|+|x−2|,−1≤x≤3, is

A

[1, 3]

B

[1, 5]

C

[3, 5]

D

None of these

Text Solution
Verified by Experts

The correct Answer is:B

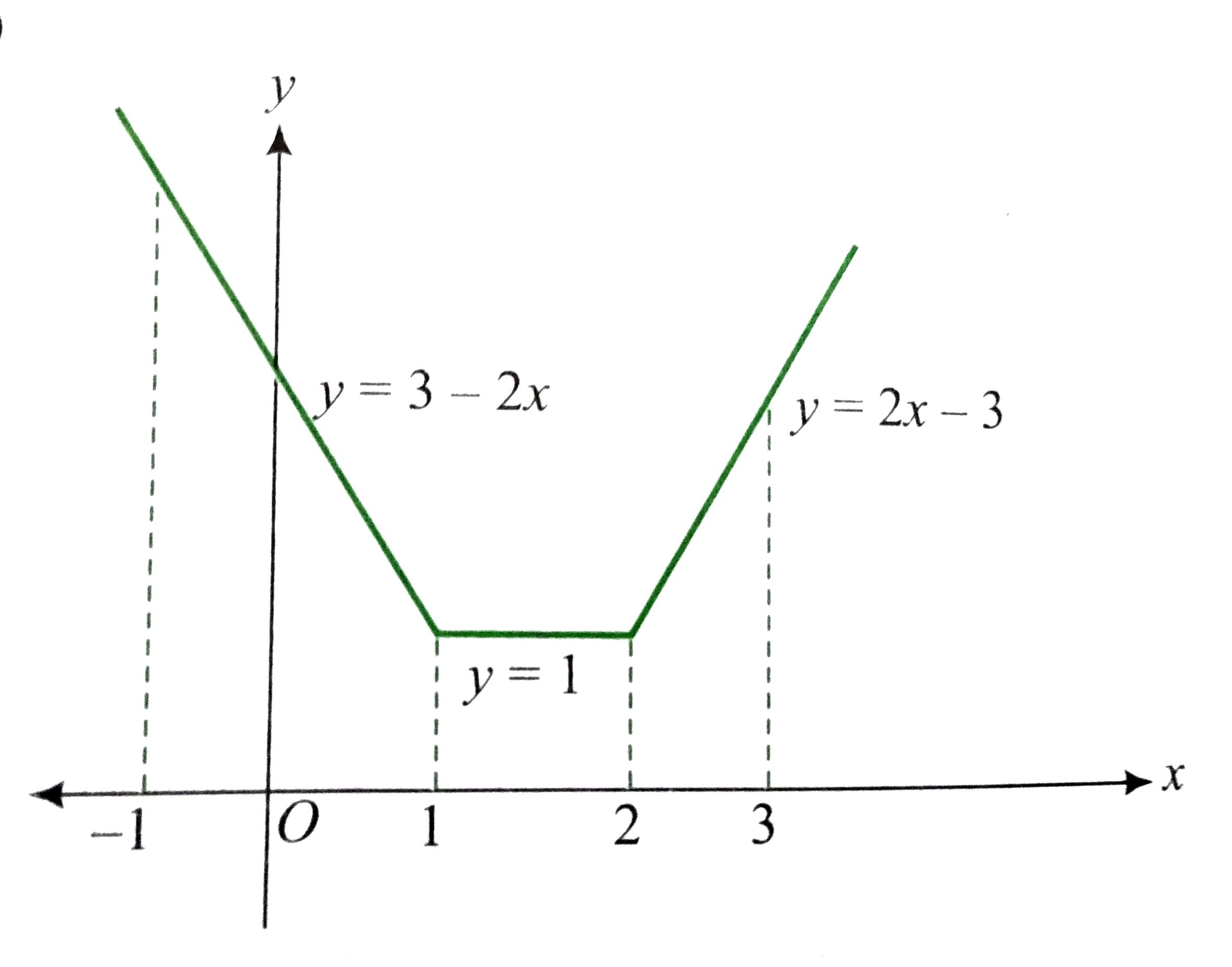
ocr_image
Clearly, from the graph, the range is [1,f(−1)]≡[1,5].
If x<1,f(x)=−(x−1)−(x−2)=−2x+3.
In this interval, f(x) is decreasing.
If 1≤x<2,f(x)=x−1−(x−2)=1.
In this interval, f(x) is constant.
If 2≤x≤3,f(x)=x−1+x−2=2x−3.
In this interval, f(x) is increasing .
∴maxf(x)=the greatest among f(−1) and f(3)=5,
minf(x)=f(1)=1
So, range=[1,5]

Doubtnut Promotions Banner
|
Updated on:21/07/2023
Class 12MATHSRELATIONS AND FUNCTIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One

    If function f(x)=x−∣∣x−x2∣∣,−1≤x≤1 then f is

    Acontinous at x=0
    Bcontinous at x=1
    Ccontinous at x=-1
    Deverywhere continous
  • Question 2 - Select One

    Find the range of the function (i) F(x)=2x2+5, where −3≤x≤2

    ARF={y∈R∣−5≤y≤23}.
    BRF={y∈R∣5≤y≤23}.
    CRF={y∈R∣5≤y≤13}.
    DNone of these
  • Question 3 - Select One

    The function f(x)=x−∣∣x−x2∣∣,−1≤x≤1 is continuous on the interval

    A[−1,1]
    B(−1,1)
    C{−1,1]−{0}
    D(−1,1)−{0}