Let f(x)=x+2|x+1|+x−1∣.Iff(x)=k has exactly one real solution, then the value of k is3 (b) 0 (c) 1(d) 2
3
0
1
2
The correct Answer is:A
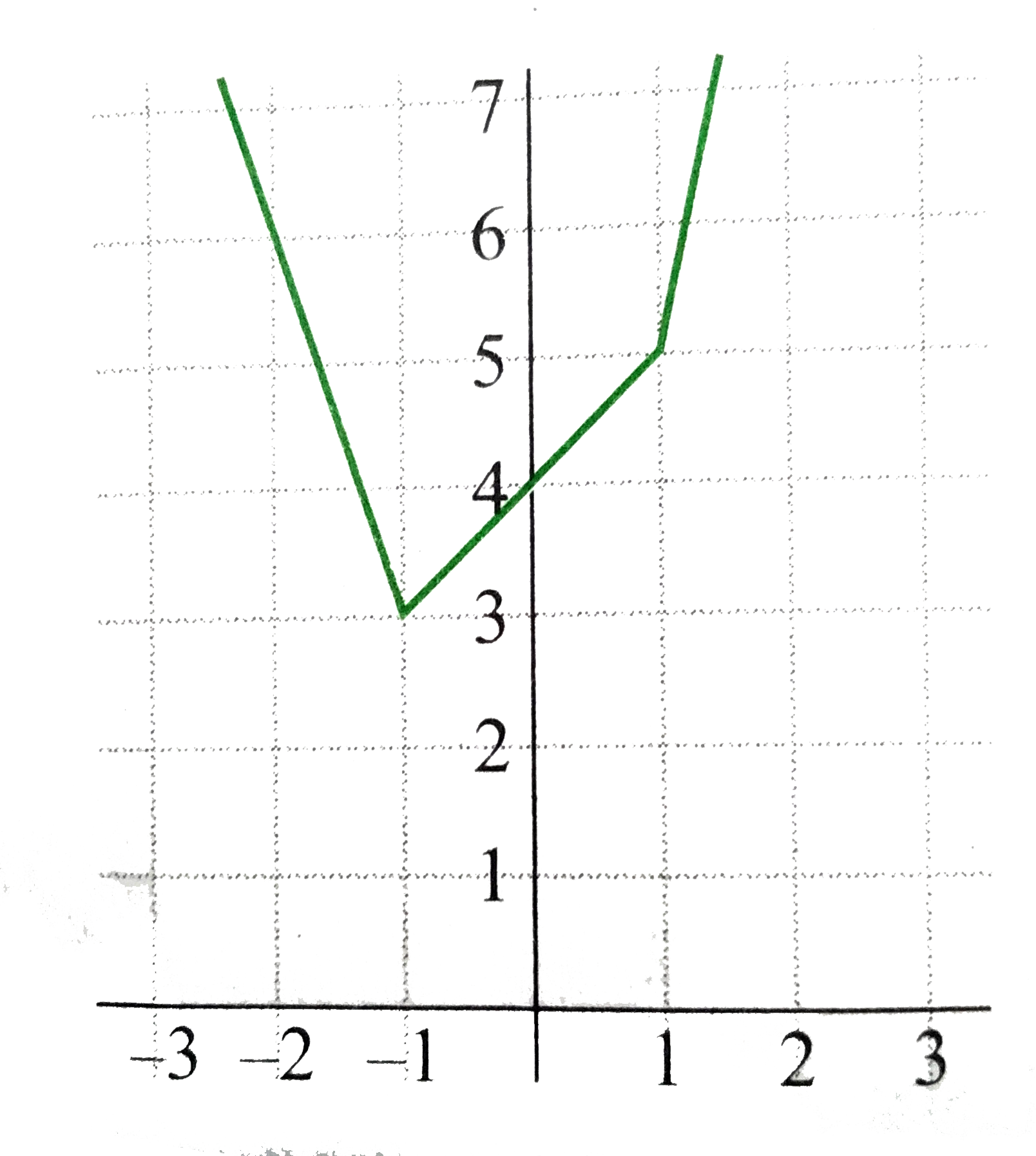
Let f(x)=x+2|x+1|+2|x−1|
=⎧⎪⎨⎪⎩x−2(x+1)−2(x−1),x<−1x+2(x+1)−2(x−1),−1≤x≤1x+2(x+1)+2(x−1),x>1
=⎧⎪⎨⎪⎩−3x,x<−1x+4,−1≤x≤15x,x>1
Topper's Solved these Questions
- RELATIONS AND FUNCTIONSBook:CENGAGEChapter:RELATIONS AND FUNCTIONSExercise:Exercise (Multiple)
Explore 27 Videos
- RELATIONS AND FUNCTIONSBook:CENGAGEChapter:RELATIONS AND FUNCTIONSExercise:Exercise (Comprehension)
Explore 32 Videos
- RELATIONS AND FUNCTIONSBook:CENGAGEChapter:RELATIONS AND FUNCTIONSExercise:Exercise 1.15
Explore 8 Videos
- Quadratic Equations, Inequalities, Modulus and LogarithmsBook:CENGAGEChapter:Quadratic Equations, Inequalities, Modulus and LogarithmsExercise:Question Bank
Explore 28 Videos
- SCALER TRIPLE PRODUCTS Book:CENGAGEChapter:SCALER TRIPLE PRODUCTS Exercise:DPP 2.3
Explore 11 Videos
Similar Questions
Let f(x)=x+2|x+1|+x−1∣.Iff(x)=k has exactly one real solution, then the value of k is 3 (b) 0 (c) 1 (d) 2
View SolutionLet f(x)=x+2|x+1|+2|x−1|. If f(x)=k has exactly one real solution, then the value of k is (a)3 (b) 0 (c)1 (d) 2
View SolutionLet f(x)=x+2|x+1|+2|x−1|. If f(x)=k has exactly one real solution, then the value of k is (a)3 (b) 0 (c)1 (d) 2
View SolutionLet f(x)=||x−1|+a|−4,iff(x)=0 has three real solution, then the values of lies in
View SolutionLet f(x)=x+2|x+1|+2|x−1|. Find the values of k if f(x)=k
(i) has exactly one real solution,
(ii) has two negative solutions,
(iii) has two solutions of opposite sign.
View SolutionLet f(x)=x+2|x+1|+2|x−1|. Find the values of k if f(x)=k
(i) has exactly one real solution,
(ii) has two negative solutions,
(iii) has two solutions of opposite sign.
View SolutionLet f(x)=2+x,x≥0 and f(x)=4−x,x<0 if f(f(x))=k has at least one solution, then smallest value of k is
View SolutionLet f(x)=exx2 x∈R−{0}. If f(x) = k has two distinct real roots, then the range of k, is
View SolutionLet f(x)=x3−3x2+2x . If the equation f(x)=k has exactly one positive and one negative solution then the value of k equals. −2√39 (b) −29 23√3 (d) 13√3
View SolutionLetf(x)=min{ex,32,1+e−x} if f(x)=k has at least 2 solutions then k can not be
View Solution
