Question

Locus of the intersection of the two straight lines passing through $(1,0)\text{and}(-1,0)$ respectively and including an angle of ${45}^{\circ }$ can be a circle with

Options

A. curve `(1,0)` and radius `sqrt(2)`
B. centre `(1,0)` and radius 2
C. centre `(0,1)` and radius `sqrt(2)`
D. centre `(0,-1)` and radius `sqrt(2)`

Answer

C::D

Explanation

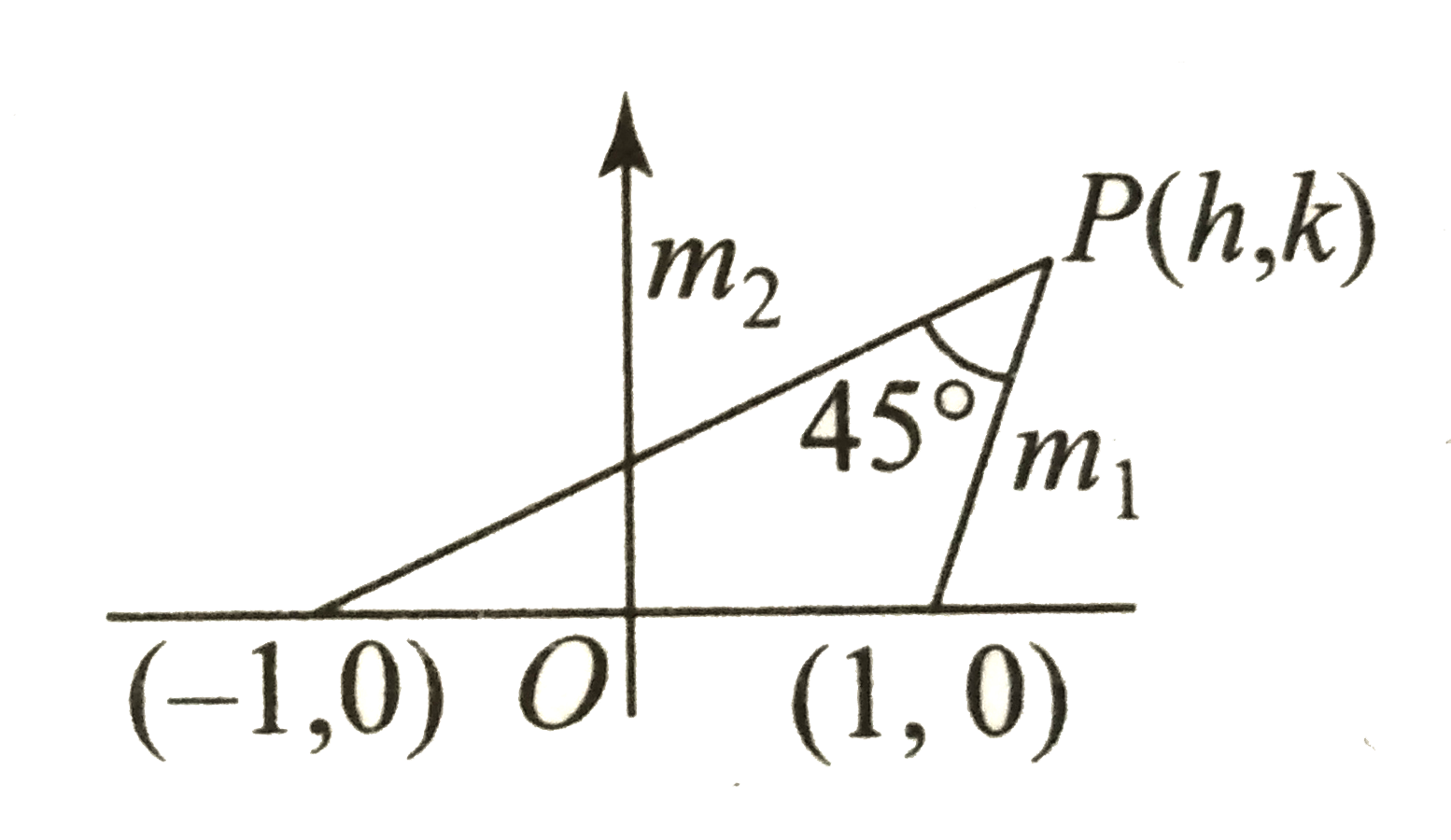
Clearly, `m_(1) = (k)/(h-1)` and `m_(2) =(k)/(h+1)`
`:. tan 45^(@) =|((k)/(h-1)-(k)/(h+1))/(1+(k^(2))/(h^(2)-1))|=|(k(h+1-h+1))/(h^(2)-1+k^(2))|`
`rArr h^(2) + k^(2) - 1 = +-2 k`
Locus is: `x^(2) + y^(2) +- 2y -1 =0`
or `x^(2) + (+-1)^(2) =2`
Thus, centre is (0,1) or `(0,-1)` and radius is `sqrt(2)`.