The line 3x+6y=k intersects the curve 2x2+3y2=1 at points AandB . The circle on AB as diameter passes through the origin. Then the value of k2 is__________Solution in Tamil
3
4
−4
−3
The correct Answer is:A, D
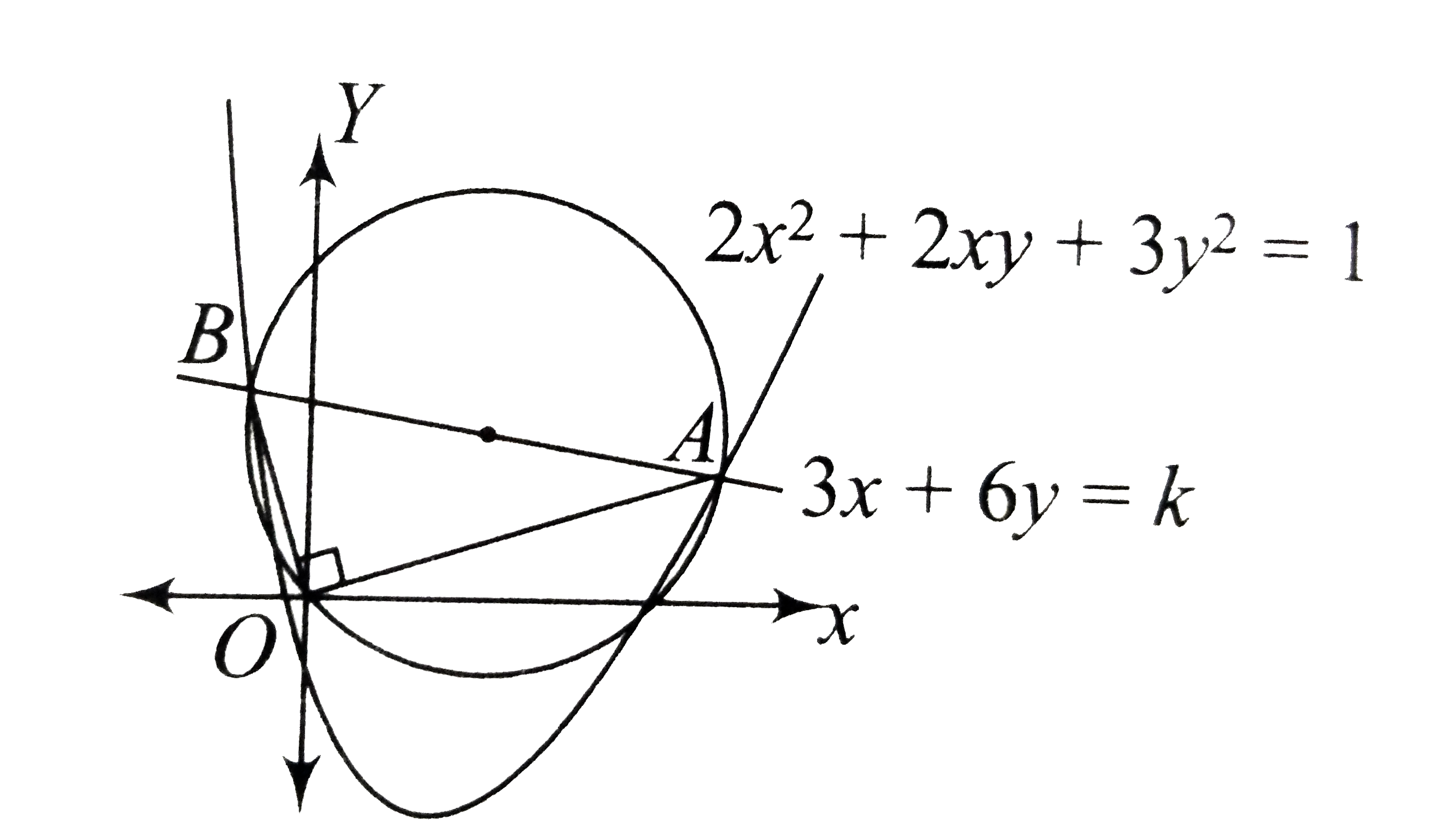
We have 3x+6yk=1 (1)
2x2+2xy+3y2−1=0 (2)
Now homogenising (2) with the help of (1), we get
⇒2x2+2xy+3y2−(3x+6yk)2=0
⇒k2(2x2+3x+3y2)−(3x+6y)2=0
Now, coefficient of x2+ coefficient of y2=0
⇒(2k2−9)+(3k2−36)=0
⇒5k2=45⇒k2=9⇒k=3 or −3
Topper's Solved these Questions
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Comprehension Type
8 Videos
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank
16 Videos
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank
16 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - MATRIX MATCH TYPE
6 Videos
- COMPLEX NUMBERSBOOK - CENGAGECHAPTER - COMPLEX NUMBERSEXERCISE - JEE Advanced Previous Year
14 Videos
Similar Questions
In the diagram as shown, a circle is drawn with centre C(1, 1) and radius I and a line L. The line Lis tangential to the circle at Q. Further L meet the y-axis at R and the x-axis at Pis such a way that the angle OPQ equals θ where 0<θ<π2 The coordinates of Q are
View SolutionIf ax+by=10 is the chord of minimum length of the circle (x−10)2+(y−20)2=729 and the chord passes through (5,15) then the value of (4a+2b) is
View SolutionIf ax+by=10 is the chord of minimum length of the circle (x−10)2+(y−20)2=729 and the chord passes through (5,15) then the value of (4a+2b) is
View SolutionFind the derivative of y=ln(cosx2).
View SolutionLet z=x+iy be a complex number where xandy are integers. Then, the area of the rectangle whose vertices are the roots of the equation zz3+zz3=350 is 48 (b) 32 (c) 40 (d) 80
View SolutionThe lines joining the origin to the points of intersection of the line 3x-2y -1 and the curve 3x2+5xy−3y2+2x+3y=0, are
View SolutionThe line 3x+6y=k intersects the curve 2x2+3y2=1 at points AandB . The circle on AB as diameter passes through the origin. Then the value of k2 is__________
View SolutionIf the straight lines joining the origin to the points of intersection of 3x^(2)-xy+3y^(2)+2x-3y+4=0 and 2x+3y=k,(k!=0) are at right angles,then find the value of 5k-6k^(2)-49
View SolutionThe radius of the circle x^(2)+y^(2)-2x+3y+k=0 is 2(1)/(2). Find the value of k .Find also the equation of diameter of the circle which passes through the point (5,2(1)/(2))
View SolutionLine 3x + by = k intersect the curve 2x^2 + 3y^2 + 2xy=1 at points A and B. Thecircle on AB as diameter passes through origin,then sum of all possible values of 'k' is
View SolutionThe line (a−1)x+ay=1 intersects the curve 3x2+4xy+2y2=1 at points A and B. The circle on AB as diameter passes through the origin, then find the sum of all possible value of 'a'.
View SolutionThe line y+2x=bis perpendicular to a line that passes through the origin. If the two lines intersect at the point (k+2,2k), what is the value of k?
View SolutionSuppose the ine x+2y=k intersects the conic 2x2+5xy+3y2=1 in two points A and B. If the circle with AB as diameter passes through the origin, then value of 14k2 is
View SolutionThe line 3x+6y=k intersects the curve 2x2+3y2=1 at points AandB . The circle on AB as diameter passes through the origin. Then the value of k2 is__________
View SolutionLine 3x+6y=k intersect the curve 2x2+3y2+2xy=1 at points A and B. Thecircle on AB as diameter passes through origin,then sum of all possible values of 'k' is
View SolutionThe radius of the circle x2+y2−2x+3y+k=0 is 2 12 Find the value of k. Find also the equation of the diameter of the circle, which passes through the point (5,212)
View Solution
