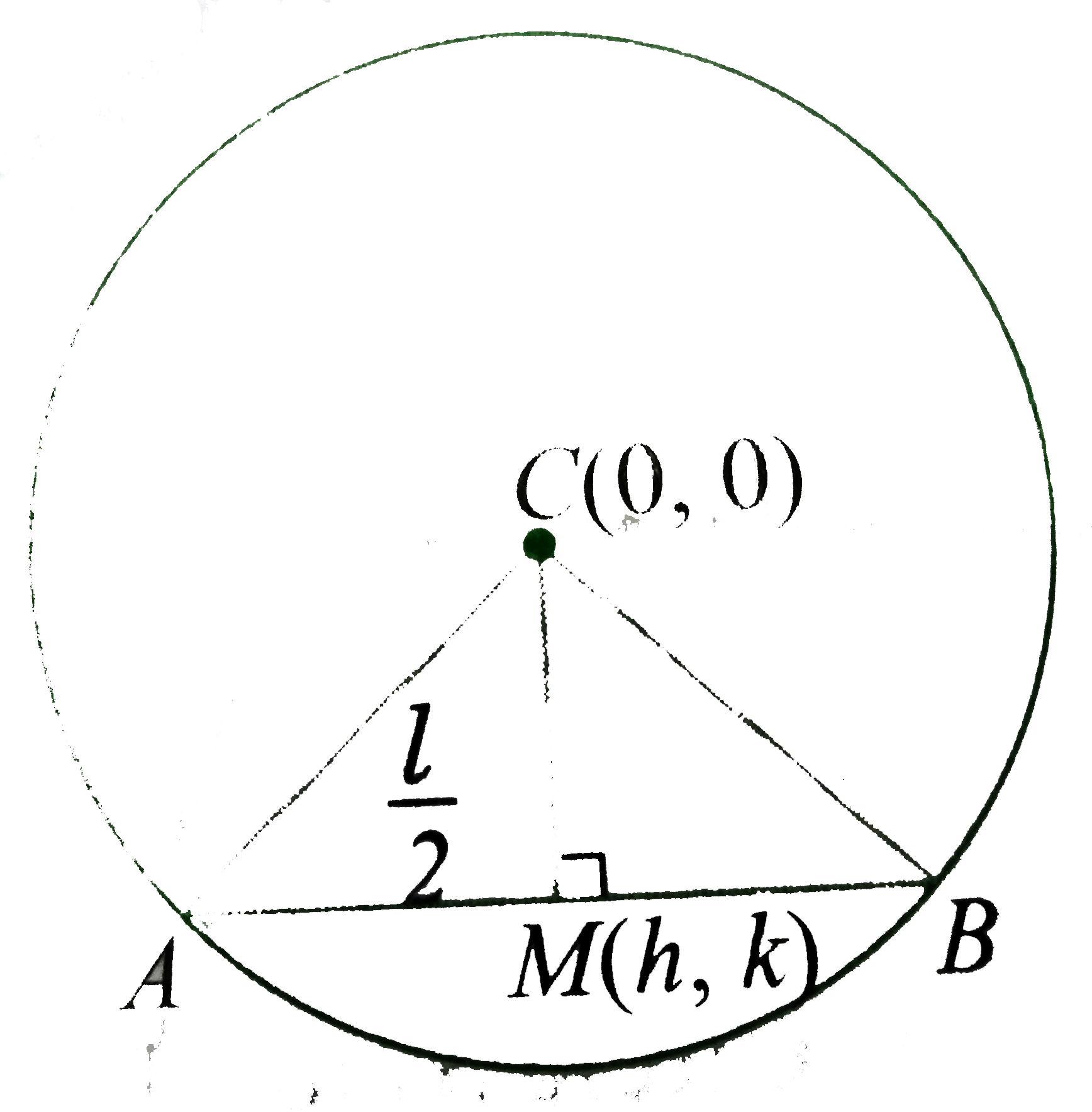
Find the locus of the midpoint of the chords of circle x2+y2=a2 having fixed length l. Solution in Tamil
As shown in the figure, AB is one of the variable chords having length l.
Let the midpoint of AB is M(h,k) .
The foot of perpendicular from centre C on AB is M.
In right angles triangled CMA, we have
AC2=CM2+AM2
∴a2=(h2+k2)+(l2)2
Hence, equation of required locus is x2+y2=a2−l24.
Topper's Solved these Questions
Similar Questions
If a circle whose center is (1,−3) touches the line 3x−4y−5=0 , then find its radius.
View SolutionFind the radius of the circle (x−5)(x−1)+(y−7)(y−4)=0 .
View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' is
View SolutionIf ax+by=10 is the chord of minimum length of the circle (x−10)2+(y−20)2=729 and the chord passes through (5,15) then the value of (4a+2b) is
View SolutionThe locus of midpoints of the chord of the circle x2+y2=25 which pass through a fixed point (4,6) is a circle.The radius of that circle is
View SolutionLocus of midpoints of chords of circle x^(2)+y^(2)=r^(2) having constant length 2l is
View SolutionLocus of midpoint of chord of circle x2+y2=1 which subtend right angle at (2,−1) is
View SolutionFind the locus of the midpoint of the chords of circle x2+y2=a2 having fixed length l.
View SolutionFind the maximum length of chord of the ellipse x2a2+y2b2=1 then find the locus of midpoint of PQ
View SolutionThe locus of midpoints of the chord of the circle x2+y2=25 which pass through a fixed point (4,6) is a circle. The radius of that circle is
View SolutionThe locus of the midpoints oof chords of the circle x2+y2=25 which touch the circle (x−12)2+(y−5)5=289 is
View SolutionThe equation to the locus of the midpoints of chords of the circle x2+y2=r2 having a constant length 2l is
View SolutionThe equation to the locus of the midpoints of chords of the circle x2+y2=r2 having a constant length 2l is
View SolutionThe length of the chords of the circle x2+y2−2x−6y−15=0 which makes an angle of 60∘ at (1,3) and the locus of the midpoints of all such chords are
View SolutionThe locus of midpoints of chords of the parabola y2=4ax which touch the circle x2+y2=a2 is
View SolutionThe equation to the locus of the midpoints of chords of the circle x2+y2=r2 having a constant length 2l is
View Solution
