Find the locus of the midpoint of the chord of the circle x2+y2−2x−2y=0 , which makes an angle of 1200 at the center.Solution in Tamil
The correct Answer is:(x−1)2+(y−1)2=1
Given circles is (x−1)2+(y−1)2=4.
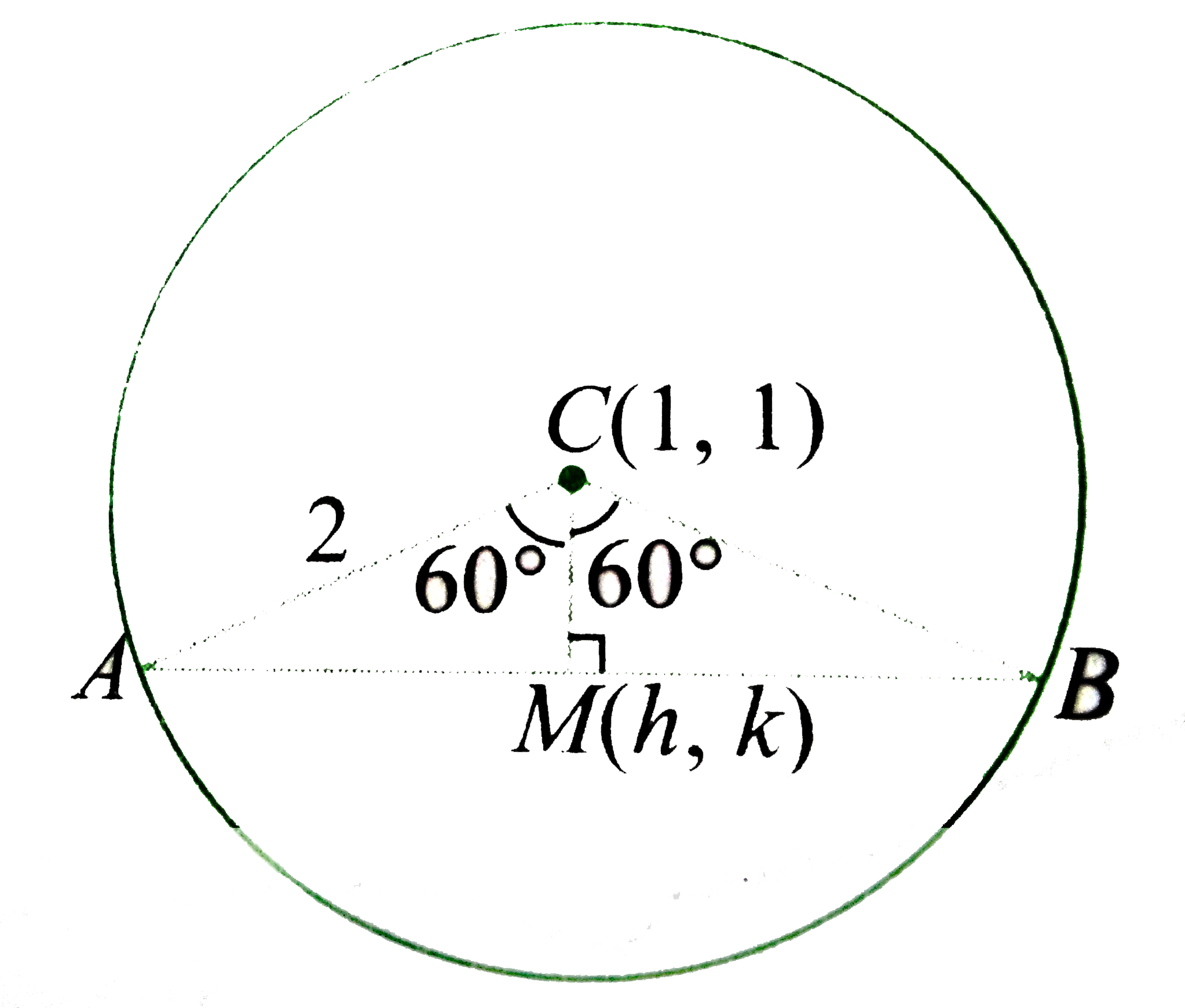
In the figure, chord AB subtends an angle of 120∘ at the centre . M (h,k) is the midpoint of chord AB.
In right angled triangle AMC,
CM=ACcos60∘
√(h−1)2+(k−1)2=2×12
Therefore, required locus is (x−1)2+(y−1)2=1
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise 4.414videos
CIRCLE
- BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise 4.512videos
CIRCLE
- BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise 4.25videos
CIRCLE
- BOOK - CENGAGECHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension11videos
BINOMIAL THEOREM
- BOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank16videos
CIRCLES
Similar Questions
Find the equation of the tangent to the circle x2+y2+4x−4y+4=0 which makes equal intercepts on the positive coordinates axes.
03:49View SolutionHow the following pair of circles are situated in the plane ? Als, find the number of common tangents . (i)x2+(y−1)2=9 and (x−1)2+y2=25 (ii) x2+y2−12x−12y=0 and x2+y2+6x+6y=0
03:32View SolutionFind the radius of the circle (x−5)(x−1)+(y−7)(y−4)=0 .
02:45View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' is07:07View SolutionIf ax+by=10 is the chord of minimum length of the circle (x−10)2+(y−20)2=729 and the chord passes through (5,15) then the value of (4a+2b) is
05:23View SolutionFind the locus of the midpoint of the chord of the circle x2+y2−2x−2y=0 , which makes an angle of 1200 at the center.
06:50View SolutionThe midpoint of the chord y=x-1 of the circle x^(2)+y^(2)-2x+2y-2=0 is
07:17View SolutionFind the locus of the midpoints of chords of hyperbola 3x2−2y2+4x−6y=0 parallel to y = 2x.
02:46View SolutionThe length of the chords of the circle x2+y2−2x−6y−15=0 which makes an angle of 60∘ at (1,3) and the locus of the midpoints of all such chords are
05:40View SolutionThe locus of the midpoint of the chord of the circle x2+y2−2x−2y−2=0 which makes an angle of 120∘ at the centre is
04:24View Solutionवृत्त x2+y2−2x−2=0 की उन जीवाओं के मध्य - बिन्दुओं का बिन्दुपथ ज्ञात कीजिए , जो केंद्र पर 120∘ का कोण अन्तरित करती है ।
06:44View SolutionThe locus of the mid-points of the chords of the circle x2+y2+2x−2y−2=0 which make an angle of 90∘ at the centre is
04:59View Solutionবৃত্ত x2+y2+2x−2y−2=0-এর যে সমস্ত জ্যা কেন্দ্রে 90°কোণ উৎপন্ন করে তাদের মধ্যবিন্দুসমূহের সঞ্চারপথ হল—
07:52View SolutionNews x^(2) + y^(2) - 2x - 2y - 2 = 0 Of chord, which is at the center 120^(@) Angle of Is the midpoint of the midpoint of
05:40View SolutionFind the locus of the midpoint of the chords of the circle x2+y2−ax−by=0 which subtend a right angle at the point (a2,b2). is
05:47View SolutionFind the locus of the midpoint of the chord of the circle x2+y2−2x−2y=0 , which makes an angle of 1200 at the center.
06:50View Solution

